
Trigonometry (MindTap Course List)
10th Edition
ISBN: 9781337278461
Author: Ron Larson
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Question
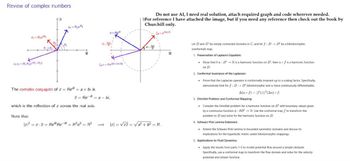
Transcribed Image Text:Review of complex numbers
22=R202
2122=R₁₂+8)
01+02
41=Rje
z=Re
Csz-Re(+2x/8)
The complex conjugate of z = Rei=a+bi is
z = Re = a - bi,
which is the reflection of z across the real axis.
Note that
Do not use AI, I need real solution, attach required graph and code wherever needed.
3For reference I have attached the image, but if you need any reference then check out the book by
Churchill only.
-2
CB-2/8
Iz²=zz Re Re = R2e0=R2
=|z|= √√zz = √√a² + b² = R.
Let D and D' be simply connected domains in C, and let f: DD' be a biholomorphic
(conformal) map.
1. Preservation of Laplace's Equation:
Show that if u : D'→R is a harmonic function on D', then u of is a harmonic function
on D.
2. Conformal Invariance of the Laplacian:
Prove that the Laplacian operator is conformally invariant up to a scaling factor. Specifically,
demonstrate that for f : DD' biholomorphic and u twice continuously differentiable.
A(f) = f(z)²(Au) of.
3. Dirichlet Problem and Conformal Mapping:
Consider the Dirichlet problem for a harmonic function on D' with boundary values given
by a continuous function : OD'→R. Use the conformal map f to transform this
problem to D and solve for the harmonic function on D.
4. Schwarz-Pick Lemma Extension:
⚫Extend the Schwarz-Pick Lemma to bounded symmetric domains and discuss its
implications for the hyperbolic metric under biholomorphic mappings.
5. Applications to Fluid Dynamics:
Apply the results from parts 1-3 to model potential flow around a simple obstacle.
Specifically, use a conformal map to transform the flow domain and solve for the velocity
potential and stream function.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 2 steps with 5 images

Knowledge Booster
Recommended textbooks for you
 Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage LearningAlgebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage LearningAlgebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning
College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning Algebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
Algebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning College AlgebraAlgebraISBN:9781305115545Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
College AlgebraAlgebraISBN:9781305115545Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning

Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781337278461
Author:Ron Larson
Publisher:Cengage Learning

Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage

College Algebra (MindTap Course List)
Algebra
ISBN:9781305652231
Author:R. David Gustafson, Jeff Hughes
Publisher:Cengage Learning

Algebra and Trigonometry (MindTap Course List)
Algebra
ISBN:9781305071742
Author:James Stewart, Lothar Redlin, Saleem Watson
Publisher:Cengage Learning

College Algebra
Algebra
ISBN:9781305115545
Author:James Stewart, Lothar Redlin, Saleem Watson
Publisher:Cengage Learning