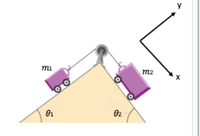
Displayed to the right are two carts connected by a cord that passes over a small frictionless pulley. Each cart rolls freely with negligible friction. Consider the mass of the cart to be m1 and the angle the first cart sits on to be θ1, while the mass of the second cart as m2 and the angle it sits on to be θ2.
a)Considering the positive x direction to be pointing as it is in the image, write an equation for the acceleration that the two blocks experience in terms of the mass of the first block m1, the angle θ1, the mass of the second block m2, the angle θ2, and the acceleration due to gravity g.
b) What is the equation for tension in regards to the rope coming from the second block? Answer in terms of the mass of the block m2, the acceleration due to gravity g, the angle θ2, and the acceleration of the blocks found previously a.
Trending nowThis is a popular solution!
Step by stepSolved in 2 steps with 1 images

- Parts D-F onlyarrow_forwardProve that launching a projectile at 45° provides the largest range. a. Write the range as a function of O. b. Take the derivative of the range with respect to and find the maximum angle.arrow_forwardMariana and Jun are both pushing on a box. Mariana pushes the box to the right and Jun pushes to the left. The box is on rollers and so is essentially frictionless with the floor, but Mariana and Jun do have friction with the floor. Jun is "winning" the pushing match and all three objects, Jun, the box, and Mariana are accelerating to the left. Consider the following forces: fm: the force of friction on Mariana f;: the force of friction on Jun FMB: the force of Mariana on the box FJB: the force of Jun on the box Which of the listed forces must be equal in magnitude and opposite in direction? Of; and FMB Mariana box Jun fm and FMB fm and fj no two of the four listed forces must be equal and opposite. FMB and FJBarrow_forward
- The graphs below show the position d of a puck plotted as a function of time t for 3 pucks moving across a smooth horizontal plane. Select the case that identifies the puck(s) experiencing equilibrium. II O A. I and II only O B. || only OC.I and III only O D.I only O E. I, II, and IIarrow_forwardin Monkey and hunter case . my doctor want me to write a 5 lines about what I have learned from this screenshot. ( The simulation shows the classic physics situation of a monkey and hunter. In this case, the hunter is trying to tranquilize the monkey, so the monkey can be relocated to a better habitat. The monkey is clever—when the tranquilizer dart (in blue) leaves the gun, the monkey (in purple) lets go of the tree branch, and starts to fall straight down. Note that there is a net (not shown) at the bottom to catch the monkey, so the monkey won't get hurt.)arrow_forwardActivity 2.12- Circular Motion Dynamics Lab Lab 1 - Horizontal Circle - Uniform (constant speed) Circular Motion Assume that a stopper is being twirled around in a horizontal circle at constant speed vas shown below. The mass of the stopper is m and the hanging mass is M. The length of the string between the stopper and the tube is L. side view FBD for stopper m FBD for M m stopper L hanging mass M glass tube +y Late +x PREDICTION: Use your knowledge of dynamics and circular motion kinematics to predict the speed. a. Draw the free body diagram for the stopper and for the hanging mass. To the right of the "=" draw an arrow labeled "ma" or "Ma" in the direction of the acceleration or write zero if the acceleration is zero. 11 b. Write Newton's 2nd law in the x direction for the stopper. Recall what you know about the acceleration for uniform circular motion and be careful with "r" - it is not equal to "L". (stopper) x: Write Newton's 2nd law in the y direction for the hanging mass…arrow_forward
- You decide to walk from your home to your friend's house. He lives 164 meters away from you at an angle 59° South of East. What is the eastern component of your displacement? m What is the southern component of your displacement? marrow_forwardE To Two blocks, labeled D and E, are connected by an essentially massless string that runs over an essentially massless and frictionless pulley, as shown in Figure 2, above. Block E hangs straight down and is initially moving downward with a speed of 3.82 meters per second. Block D is on an inclined plane, inclined at an angle of theta = 0 = 28.6 degrees above horizontal. There is no friction between block D and most of the plane, but there is friction (with a coefficient of kinetic friction equal to 0.49) between block D and a region of length L = 0.628 meters. Block D will slide over that region (and pass it completely) before it gets to the end of the plane. Block D has a mass of 0.74 kg, while block E has a mass of 0.98 kg. The only significant forces acting on the blocks are friction acting on block D (while in contact with the region with friction), normal forces, tension forces, and gravity (with g = 9.81 m/s2). Calculate the speed of block D (in units of meters per second)…arrow_forward