
College Physics
11th Edition
ISBN: 9781305952300
Author: Raymond A. Serway, Chris Vuille
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Concept explainers
Topic Video
Question
Sketch the vectors representing the projectile’s horizontal and vertical velocities. Note that the length of the directed line segment must be proportional to the vector’s magnitude and the arrowhead must point to the vector’s direction.
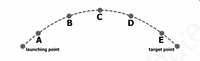
Transcribed Image Text:C
D
A
target point
launching point
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 3 steps with 1 images

Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.Similar questions
- As a projectile moves in its parabolic path, where are the velocity and acceleration vectors perpendicular to each other? (a) Everywhere along the projectile’s path, (b) at the peak of its path, (c) nowhere along its path, or (d) not enough information is given.arrow_forwardYou launch a projectile at an initial speed of 38.8 m/s from the ground. After 5.10 seconds of flight, the projectile lands on the ground. At what angle above the horizontal was the projectile launched?arrow_forwardConnor McDavid is working on his snapshot accuracy and during one particular shot, he is positioned a horizontal distance of 20 m from the net. The target he ends up hitting in the net is 0.7 m above the ice surface and when he makes the successful shot, the puck leaves his stick and the ice at an angle of 8° above the horizon.What is the initial speed (magnitude of resultant velocity) of the puck just as it leaves the stick or surface of the ice?During the shot, how long does it take for the puck to travel from the stick or ice to the raised target located in the net?arrow_forward
- Consider a projectile being launched with an initial speed of 43 m/s at a variety of initial angles. Refer to the figure. What is the range, in meters, of the projectile if it is launched at an angle of θ1 = 79.7°? What is the range, in meters, of the projectile if it is launched at an angle of θ2 = 40.5°? What is the range, in meters, of the projectile if it is launched at an angle of θ3 = 90 − 79.7°, the complement of θ1?arrow_forwardConsider the trajectory diagram shown below for a horizontally launched projectile. On the diagram, draw vector arrows representing the vx and vy velocity components during the course of the motion. The length of the arrows should represent the magnitude of the velocity components. Label each component. (Note that the velocity components are already shown for the first position.)arrow_forwardGoro Shigeno (Pitcher) and Joe Gibson Jr. (hitter) went head to head in the Major League Baseball (MLB) series. For the last pitch, Gibson hits the baseball with 82 ft/s at angle of 75°. When the ball is directly above Shigeno he begins to run under it. Determine the (a) the total distance that the pitcher runs and (b) the constant speed at which pitcher must run in order to make the catch at the same elevation at which the ball was hit. (Note: the distance between the pitcher and hitter is 70 ft.)arrow_forward
- You can use any coordinate system you like in order to solve a projectile motion problem. To demonstrate the truth of this statement, consider a ball thrown off the top of a building with a velocity v at an angle 0 with respect to the horizontal. Let the building be 54.0 m tall, the initial horizontal velocity be 9.10 m/s, and the initial vertical velocity be 10.5 m/s. Choose your coordinates such that the positive y-axis is upward, and the x-axis is to the right, and the origin is at the point where the ball is released. (a) With these choices, find the ball's maximum height above the ground and the time it takes to reach the maximum height. maximum height above ground time to reach maximum height (b) Repeat your calculations choosing the origin at the base of the building. maximum height above ground time to reach maximum heightarrow_forwardUse a graphing utility to obtain the path of a projectile launched from the ground (h = 0) at the specified values of θ = 35°, v0 = 300 feet per second. In the exercise, use the graph to determine the maximum height and the time at which the projectile reaches its maximum height. Also use the graph to determine the range of the projectile and the time it hits the ground. Round all answers to the nearest tenth.arrow_forwardThis problem will involve deriving a formula or two for a projectile launched from one height and angle and landing at a different height on Earth. Begin with a projectile launched at angle 0 above horizontal from a height y₁ with initial velocity Vo. The projectile lands at a point with height y₂. These are the given quantities: vo, 0, y₁, y2 and g. Construct formulae for each of the following, as. a function of given quantities the horizontal distance traveled. the maximum height reached. the time taken. the angle of impact. (find the final velocity components first).arrow_forward
- Two projectiles are launched from two different heights, as shown in the figure. Projectile A, on the left, is launched from a 30 m building, with an initial speed of 50 m/s in a horizontal direction. Projectile B, at right, it is launched from a building 100 m from the other building and from a height of 15 m. It is also known that the Projectile B is launched at an angle of 135° with respect to the horizontal, with unknown initial velocity. Find: a) The initial velocity with which projectile B is launched. b) The time elapsed from when the projectiles are launched to when they crash into each other. 30m √(4) 100m 2-18) 15m 135°arrow_forwardA student throws a water balloon with speed v0 from a height h = 1.82 m at an angle θ = 29° above the horizontal toward a target on the ground. The target is located a horizontal distance d = 5.5 m from the student’s feet. Assume that the balloon moves without air resistance. Use a Cartesian coordinate system with the origin at the balloon's initial position. A) What is the position vector , Rtarget, that originates from the balloons original position and terminates at the target? Put this in terms of h and d, and represent it as a vector using i and j. B) In terms of the variables in the problem , determine the time,t, after the launch it takes the balloon to reach the target. Your answer should not include h. C) Create an expression for the balloons vertical position as a function of time, y(t), in terms of t, v0, g, and θ.arrow_forwardAn arrow is fired with an initial velocity v0 at an angle θ0 above horizontal. Assume the arrow moves without air resistance. Use a Cartesian coordinate system with the origin at the arrow's initial position to analyze the arrow's motion. Some time later the arrow makes an angle of θ = 15 degrees with respect to the horizontal. Write an expression for the time, t, that passes between when the arrow was fired and this later point. t = ?arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
 College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning University Physics (14th Edition)PhysicsISBN:9780133969290Author:Hugh D. Young, Roger A. FreedmanPublisher:PEARSON
University Physics (14th Edition)PhysicsISBN:9780133969290Author:Hugh D. Young, Roger A. FreedmanPublisher:PEARSON Introduction To Quantum MechanicsPhysicsISBN:9781107189638Author:Griffiths, David J., Schroeter, Darrell F.Publisher:Cambridge University Press
Introduction To Quantum MechanicsPhysicsISBN:9781107189638Author:Griffiths, David J., Schroeter, Darrell F.Publisher:Cambridge University Press Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Lecture- Tutorials for Introductory AstronomyPhysicsISBN:9780321820464Author:Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina BrissendenPublisher:Addison-Wesley
Lecture- Tutorials for Introductory AstronomyPhysicsISBN:9780321820464Author:Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina BrissendenPublisher:Addison-Wesley College Physics: A Strategic Approach (4th Editio...PhysicsISBN:9780134609034Author:Randall D. Knight (Professor Emeritus), Brian Jones, Stuart FieldPublisher:PEARSON
College Physics: A Strategic Approach (4th Editio...PhysicsISBN:9780134609034Author:Randall D. Knight (Professor Emeritus), Brian Jones, Stuart FieldPublisher:PEARSON

College Physics
Physics
ISBN:9781305952300
Author:Raymond A. Serway, Chris Vuille
Publisher:Cengage Learning

University Physics (14th Edition)
Physics
ISBN:9780133969290
Author:Hugh D. Young, Roger A. Freedman
Publisher:PEARSON

Introduction To Quantum Mechanics
Physics
ISBN:9781107189638
Author:Griffiths, David J., Schroeter, Darrell F.
Publisher:Cambridge University Press

Physics for Scientists and Engineers
Physics
ISBN:9781337553278
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning

Lecture- Tutorials for Introductory Astronomy
Physics
ISBN:9780321820464
Author:Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina Brissenden
Publisher:Addison-Wesley

College Physics: A Strategic Approach (4th Editio...
Physics
ISBN:9780134609034
Author:Randall D. Knight (Professor Emeritus), Brian Jones, Stuart Field
Publisher:PEARSON