
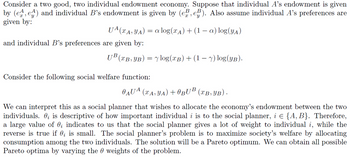
Write down the Planner’s problem, as well as the Lagrangian. (Hint: There should be two constraints because aggregate consumption of each good cannot exceed the economy’s endowment.) Label the multipliers ϕ1 and ϕ2.
What are the first order conditions of the Planner’s problem? (Hint: There should be 6.).
Find the solution to the Planner’s problem and label the quantities (xpA; ypA) and (xpB; ypB).
If we compare the first order conditions of the Planner’s problem to the first order conditions of a corresponding competitive equilibrium (CE), we can obtain a relationship between ϕx, ϕy, λ, px, and py so that the CE and the Planner’s problem give us the same solution. This is called decentralizing the Planner’s problem.
Decentralize the Planner’s problem. (find the
Can you relate this to the two welfare theorems?
Step by stepSolved in 1 steps with 7 images

- A consumer spends all her income on the two goods A and B. The quantity of good A bought is determined by the demand function QA = f(PA, PB,M) where PA and PB are the prices of the two goods and M is real income. A change in the price of A will also affect real income M via the function M = g(PA, PB, £M) where £M is money income. Derive an expression for the total effect of a change in PA on QA.arrow_forwardSuppose that there are only 10 individuals in the economy each with the following utility function over present and future consumption: U (c1, c2) = c1 +C2, where ci is consumption today, and c2 is consumption tomorrow. Consumption tomorrow is less valued because people are impatient and prefer consuming now rather than later. Buying 1 unit of consumption today costs $1 today and buying 1 unit of consumption tomorrow costs $1 tomorrow. All individuals have income of $10 dollars today and no income tomorrow (because they will be retired) but they can save at the market interest rater> 0. How much of his or her income will an individual consume today given that the interest rate is 0.3? O. Less than half of it O. Exactly half of it O. The individual is indifferent between consuming today and saving O. More than half of it O. All of it O. None of it How much of his or her income will an individual consume today given that the interest rate is 0.5? O. Less than half of it…arrow_forwardHello, I need some assistance with the attached question. It concerns constrained consumer optimisation.arrow_forward
- Please get this right. People keep getting wrong. Thank you.arrow_forwardHand written solutions are strictly prohibitedarrow_forwardplease only do: if you can teach explain steps of how to solve each part of formula ? x(p,w)={(2w/3p1,w/3p1) if p1<p2(2w/3p1,w/3p2) if p1≥p2 ? how to solve for thisarrow_forward
- QUESTION 1 x0.80Qy(1-0.80); and the budget 153 = 11Qx + 12Qy find the CHANGE in optimal consumption of X if the price of X increases by a factor of 1.9. as a positive number with 1 decimal and 5/4 rounding (e.g. 1.15 1.2, 1.14 = 1.1). Qx For the utility function U = Please enter your responsearrow_forwardFor the utility function U=Qx0.370,(1-0.37) and the budget 194 140x+6Qy find the CHANGE in optimal consumption of X if income increases by a factor of 1.4. Please enter your response as a positive number with 1 decimal and 5/4 rounding (e.g. 1.15 1.2, 1.14=1.1).arrow_forwardFor example, the economy of a country can be explained by several models as follows: Consumer: The consumer derives his satisfaction from his current consumption, future consumption and his free time (u(C,C',l,l'). He maximizes his utility by: C+wl+11+rC'+w'1+rl'=wh+π-T+w'h+'-T'1+r It is assumed that consumer preferences meet the general conditions that are often used (more is better, preference for diversity, all goods are normal, substitution effect is more dominant). Firm: In the first period, the firm has K capital and employs N workers to produce Y output: Y outputs: Y=zF(K,N) The profit in the first period is =Y-wN-I Where I is the company's investment. Investments increase the capital stock for the second period: K'=1-dK+I In the second period the firm will produce output of Y': Y'=z'F(K',N') Then the profits obtained in the second period are: '=Y'-w'N' Firms choose the demand for labor (N, N') and the demand for investment I to maximize their value: V=π+π'1-r…arrow_forward
- Use Lagrange multipliers to find expressions for x, and x₂ which maximise the utility function U = x/² + x1/² subject to the general budgetary constraint P₁x₁ + P₂x₂ = Marrow_forwardPlease get right, thank you.arrow_forwardConsider the following consumer’s problem: max u(c1 ) + βu(c2) c1c2 subject to c1 + s1 = y1 c2 = y2 + (1 + r)s1 u(c)={1/(1-1/σ}c1-1/σ a) Describe all equations using economic terminology.b) Derive the intertemporal budget constraint for this consumer.c) Set the Langrangean for this problem.d) Find the first order conditions for a maximum.e) What is the effect of an increase in the interest rate on savings? Show in equation and explain.arrow_forward

 Principles of Economics (12th Edition)EconomicsISBN:9780134078779Author:Karl E. Case, Ray C. Fair, Sharon E. OsterPublisher:PEARSON
Principles of Economics (12th Edition)EconomicsISBN:9780134078779Author:Karl E. Case, Ray C. Fair, Sharon E. OsterPublisher:PEARSON Engineering Economy (17th Edition)EconomicsISBN:9780134870069Author:William G. Sullivan, Elin M. Wicks, C. Patrick KoellingPublisher:PEARSON
Engineering Economy (17th Edition)EconomicsISBN:9780134870069Author:William G. Sullivan, Elin M. Wicks, C. Patrick KoellingPublisher:PEARSON Principles of Economics (MindTap Course List)EconomicsISBN:9781305585126Author:N. Gregory MankiwPublisher:Cengage Learning
Principles of Economics (MindTap Course List)EconomicsISBN:9781305585126Author:N. Gregory MankiwPublisher:Cengage Learning Managerial Economics: A Problem Solving ApproachEconomicsISBN:9781337106665Author:Luke M. Froeb, Brian T. McCann, Michael R. Ward, Mike ShorPublisher:Cengage Learning
Managerial Economics: A Problem Solving ApproachEconomicsISBN:9781337106665Author:Luke M. Froeb, Brian T. McCann, Michael R. Ward, Mike ShorPublisher:Cengage Learning Managerial Economics & Business Strategy (Mcgraw-...EconomicsISBN:9781259290619Author:Michael Baye, Jeff PrincePublisher:McGraw-Hill Education
Managerial Economics & Business Strategy (Mcgraw-...EconomicsISBN:9781259290619Author:Michael Baye, Jeff PrincePublisher:McGraw-Hill Education





