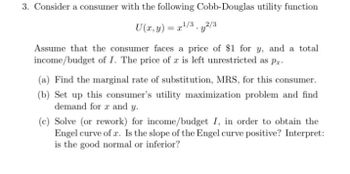
Consider a consumer with the following Cobb-Douglas utility function U(x,y) = x¹/³.y2/3 Assume that the consumer faces a price of $1 for y, and a total income/budget of I. The price of r is left unrestricted as pr. (a) Find the marginal rate of substitution, MRS, for this consumer. (b) Set up this consumer's utility maximization problem and find demand for x and y. (c) Solve (or rework) for income/budget I, in order to obtain the Engel curve of r. Is the slope of the Engel curve positive? Interpret: is the good normal or inferior?

Consider a consumer with the utility function U(F,C) = min(F,2C); that is, the two goods are perfect complements that have to be used in a fixed proportion. a) PF = 4 and PC = 8 while her income is 96. Determine the optimum consumption basket and the utility level. b) Now consider that price of food rises to 8. Calculate the income effect and substitution effect.

Compute marginal utilities and marginal rate of substitution for each of the utility functions given below. a) U(X,Y)= 1- e-ax -e-by b) U(X,Y)= XY + 3X + 5Y c) U(X,Y)= (0.3Xm +0.78m)/1 X1-a y1-b d) U(X,Y)= + 1-a 1-b

Solve the attachment

Consider a person who consumes two goods, x and y, and has a utility function given by U(x, y) = In(x)+y. This person has an income of $100 and faces a price of $0.50 for good x and $1 for good y. Price of x then rises to $0.60. Solve for the compensating variation (CV) and equivalent variation (EV) of this price change. Show your work.

Kim spends all her weekly income of R200 on two goods, X and Y. The prices of the two goods are R5 per unit for X and R2 per unit for Y. She has a utility function expressed as: U(X, Y) = 5XY Express the budget equation mathematically.  Determine the utility-maximising bundle of X and Y.  Calculate the total utility that will be generated per unit of time for this individual.

Laura's preferences over commodities X₁ and x₂ can be represented by U(x₁,x2)=min{3x₁, x₂}. She maximizes her utility subject to her budget constraint. Suppose there is an increase in p1. There are both income and substitution effects of this price change. There is an income effect but not a substitution effect of this price change. There is a substitution effect but not an income effect of this price change. It is unclear whether the consumer will buy more or less x1 as a result of the increase in p1.

A consumer’s preferences over pizza (x) and steak (y) are given by u(x,y) = x2y (HINT: MUx = 2xy and MUy = x2) and his income is I = $120 and py = $1. (a) Calculate his optimal bundle when pX = $8 (call this bundle A) and separately when pX = $1 (call this point C). (b) Finding the decomposition bundle B, calculate the income and substitution effects on the amount of pizza of a decrease in the price of pizza from pX = $8 down to pX = $1. (c) Forget about the decomposition bundle and the two effects. In (a), the price of pizza decreases, hence the agent ends up better off. Let’s quantify how much “better off” the agent becomes after this price drop, in dollars. For this, instead of the price drop, suppose the agent is given some money $m and he optimize utility with this additional gift included to his budget. What should m be, so that his optimal utility with his expanded budget is exactly equal to his utility at the bundle C (the bundle he chooses optimally when pizza price drops to…

Let t = 3 The consumer has a preference relation defined by the utility function u(x, y) = −(t + 1 − x)2 − (t + 1 − y)2. He has an income of w > 0 and faces prices px and py of goods X and Y respectively. He does not need to exhaust his entire income. The budget set of this consumer is thus given by B={(x,y)∈R2+ :pxx+pyy≤w } A.  Is the optimal demand for good 1 everywhere differentiable with respect to w? informal argument is sufficient

4. Assume Megan has preferences represented by utility function U (x₁, x2) = (x₁)¹/4 (x2)³/4 Good 1 is electricity (1) while good 2 is gasoline (x2). Suppose the consumer has an income level of m= 1000 dollars. In addition, the price of good 1 is p₁ = 5 and the price of good 2 is p2 = 5. (a) Compute the Marginal Rate of Substitution (MRS). (b) Find the optimal levels of electricity (x) and gasoline (x2) consumption for Megan. (c) Suppose the government imposes a per-unit tax of t = 5 dollars on gasoline. Find the optimal levels of electricity (x) and gasoline (x2) consumption for Megan with the tax.