Question
A long cylinder of charge q has a radius a. The charge density within its volume, p, is uniform (Figure). Describe the form of the electric field generated by the cylinder. Find the electric field strength at a distance r from the axis of the cylinder in the regions (i) r > a and (ii) 0 <r<a.
If a non-relativistic electron moves in a circle at a constant distance R from the axis of the cylinder, where R > a, find an expression for its speed.
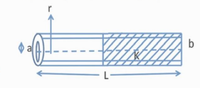
Transcribed Image Text:b
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 4 steps

Knowledge Booster
Similar questions
- Find the position vector r(arrow) that points from Proton 1 to the Proton 2, its magnitude |r(arrow)∣, and its unit vector, r(hat). Look at the image for the question.arrow_forwardProblem 2.01. Three plates with surface charge density |o| = 8.85 μC/mm² are stacked on top of each other. The top and bottom plates have charge density to while the center plate has charge density -0. (a) Find the magnitude and direction of the electric field between the plates. (b) Find the magnitude and direction of the electric field above and below the plate stack.arrow_forwardA simple and common technique for accelerating electrons is shown in the figure, which depicts a uniform electric field between two plates. Electrons are released, usually from a hot filament, near the negative plate, and there is a small hole in the positive plate that allows the electrons to pass through. Randomized Variables E = 2.7 × 104 N/C Calculate the horizontal component of the electron's acceleration if the field strength is 2.7 × 104 N/C. Express your answer in meters per second squared, and assume the electric field is pointing in the negative x-direction as shown in the figure.arrow_forward
- Suppose we have a charge, q1=1 μC. This charge makes an electric field some distance r=60 cm away from it. Now suppose our measurement of q1 is only accurate to within 0.1 μC, and our measurement of r is only accurate to within 2 cm. What is the uncertainty in our field calculation due only to the uncertainty in the charge separation r? for the uncertainty of q I got 2500 N/C. I keep getting 1667.67 N/C for the uncertainty for the separation of r but that is wrongarrow_forwardConsider the special shape pictured in the diagram below. It is a cylinder, centered on the origin with its axis oriented along z, and it has been partially hollowed to leave two cone-shaped cavities at the top and bottom of the cylinder. The radius of the object is a, its height is 2a, and the solid part of the object (the shaded region that is visible in the rightmost panel of the illustration above, which shows a drawing of the cross-section of the object) has a uniform volume charge density of po. Assume that the object is spinning counter clockwise about its cylinder axis at an angular frequency of w. Which of the following operations is part of the calculation of the magnitude of the current density that is associated with the motion of the rotating object as a function of r (select all that apply)?arrow_forwardAn electron is projected with an initial speed v0 = 1.70×106 m/s into the uniform field between the parallel plates in (Figure 1). Assume that the field between the plates is uniform and directed vertically downward, and that the field outside the plates is zero. The electron enters the field at a point midway between the plates. If the electron just misses the upper plate as it emerges from the field, find the magnitude of the electric field. Express your answer in newtons per coulomb. Suppose that in the figure the electron is replaced by a proton with the same initial speed v0v0. Would the proton hit one of the plates? What would be the direction of proton's displacement? displacement is upward displacement is downwardarrow_forward
- Consider a solid uniformly charged dielectric sphere where the charge density is give as ρ. The sphere has a radius R. Say that a hollow of charge has been created within the spherethat is offset from the center of the large sphere such that the small hollow has its center on the x axis where x = R/2. Using a standard frame where the large frame has its center at the origin, find the Electric field vector at the following points. a.The origin b.Anywhere inside the hollow (challenging) c.x = 0, y = R d.x = -R, y =0arrow_forwardWe have calculated the electric field due to a uniformly charged disk of radius R, along its axis. Note that the final result does not contain the integration variable r: R. Q/A 2€0 Edisk (x² +R*)* Edisk perpendicular to the center of the disk Uniform Q over area A (A=RR²) Show that at a perpendicular distance R from the center of a uniformly negatively charged disk of CA and is directed toward the disk: Q/A radius R, the electric field is 0.3- 2€0 4.4.1barrow_forwardSuppose we have a charge, q1=3 μC. This charge makes an electric field some distance r=69 cm away from it. Now suppose our measurement of q1 is only accurate to within 0.1 μC, and our measurement of r is only accurate to within 1 cm. a)If we were to calculate the electric field made by that charge at the indicated distance, what would be the uncertainty in our calculation due only to the uncertainty in the size of q1? b)What is the uncertainty in our field calculation due only to the uncertainty in the charge separation r? c)What is the total uncertainty in our electric field calculation due to the uncertainty in the size of q1 and the uncertainty in the charge separation r?arrow_forward
- Suppose we have a charge, q1=1 μC. This charge makes an electric field some distance r=73 cm away from it. Now suppose our measurement of q1 is only accurate to within 0.2 μC, and our measurement of r is only accurate to within 1.5 cm. What is the uncertainty in our field calculation due only to the uncertainty in the charge separation r? Every time I do this calculation I get a different number all of which have been wrong. I have gotten 694.06, 693.097, 46.2, .69405, and 71.56. Can you please help me with this?arrow_forwardConsider the following situation. A positive charge q is located a distance R from a charged wire bent into a circular arc as drawn. The arc has radius R, a net positive charge Q, and an angular extent of 30° (T/6 radians) centered on the same axis as q. 15° a. Calculate the electric field (vector) the arc of wire produces at q's location. Give the vector in component form. The vector only has one component. You should write down in words an argument using the problem's symmetry for this. b. Find the net force (vector) on q by the arc of wire. Give the vector in component form. c. Compare this force to the force produced by a positive point charge Q located a distance R away from q. Which force is greater, (b) or (c), and by what fraction? Show this algebraically.arrow_forwardProblems 4-5 refer to the following diagram and situation. A point charge (with charge Q) at the end of an insulating cord is observed to be in equilibrium in a uniform horizontal electric field of when the pendulum's angle with the vertical is 0 = 38.6°, is as shown in the Figure. The electric field is to the right and the strength of the electric field is 9500 N/C. The mass of the point charge is m = 0.0200 kg and the acceleration due to gravity is 9.8 m/sec². Problem 4: What is the tension on the cord? a. 0.251 N Problem 5: What is the charge Q? a. (1.65 x 10-5)C E b. 0.451 N b. (3.65 x 10-5)C 0 Q m c. 0.651 N C. (5.65 x 10-5) C d. 0.851 N d. (7.65 x 10-5)Carrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios