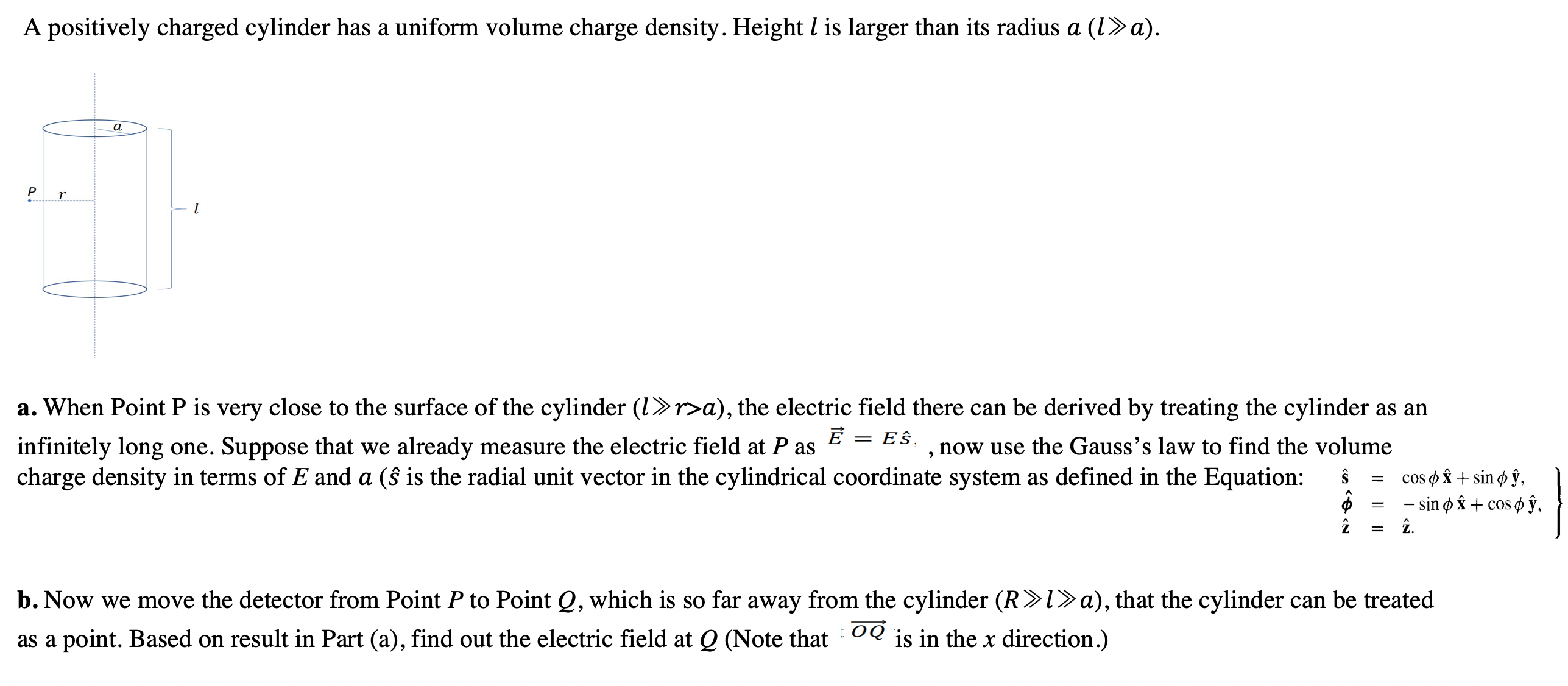
A positively charged cylinder has a uniform volume charge density. Height l is larger than its radius a (1»a). a P a. When Point P is very close to the surface of the cylinder (1>r>a), the electric field there can be derived by treating the cylinder as an = Eŝ. infinitely long one. Suppose that we already measure the electric field at P as charge density in terms of E and a (ŝ is the radial unit vector in the cylindrical coordinate system as defined in the Equation: now use the Gauss's law to find the volume cos o â + sin ø ŷ, - sin ø Âx + cos ø ŷ, î. b. Now we move the detector from Point P to Point Q, which is so far away from the cylinder (R>l>a), that the cylinder can be treated as a point. Based on result in Part (a), find out the electric field at Q (Note that OQ is in the x direction.)

A sphere shell of radius R, centered on the origin, has a uniform surface charge density /sigma and no charge in its interior. What is the magnitude of the electric field outside the sphere, at the distance r from the center, where r > R?

Kenny is a student in engineering physics, he wants to study about the infinite long cable that has an insulating cylinder with radius a with uniform charge volume density 2p surrounded by a uniform conducting material with outer radius of 2a and zero total charge, as he drawn in Figure Q1.1. 2a Figure Q1.1 a) Using Gauss's law, find the electric field inside the insulating cylinder. Draw a Gaussian surface and indicate your choice of a coordinate system. b) Find the inner and outer charge surface density of the conducting cylinder, oin and Tout. c) Determine the work required to move a charge qo from r₁ = 4a to rf = 1.57a where r is the distance from the cylindrical axis.