College Physics
11th Edition
ISBN: 9781305952300
Author: Raymond A. Serway, Chris Vuille
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Related questions
Concept explainers
Topic Video
Question
Part G
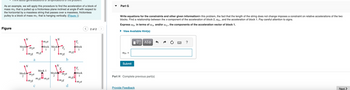
Transcribed Image Text:The content presented is part of a physics problem related to the dynamics of a block system on an inclined plane. It explains how to find the acceleration of a block of mass \( m_2 \), which is pulled up a frictionless plane inclined at an angle \( \theta \) with respect to the horizontal by a massless string. This string passes over a massless, frictionless pulley to a block of mass \( m_1 \) that is hanging vertically.
### Figure 1 Description:
The figure is divided into four parts labeled a, b, c, and d. They represent different configurations or components of the block system:
- **Figure a**: Shows Block 1 with mass \( m_{1g} \) moving on the inclined plane.
- **Figure b**: Similar to Figure a, shows another Block 2 with mass \( m_{2g} \) on the inclined plane.
- **Figure c**: Depicts Block 1 being pulled by a tension \( T_{3} \) and influenced by the gravitational force \( m_{1g} \).
- **Figure d**: Shows Block 2 with similar forces as in Figure c but with slight variations in the directions and magnitudes.
### Problem Explanation:
**Part G**: The task is to write equations for the constraints and given information in this block system. The aim is to find a relationship between the x-component of the acceleration of Block 2, \( a_{2x} \), and the acceleration of Block 1. The problem notes that the constant length of the string imposes a constraint on the relative accelerations of the two blocks. It emphasizes careful attention to signs when expressing \( a_{2x} \) in terms of \( a_{1x} \) and/or \( a_{1y} \), which are components of the acceleration vector of Block 1.
Below, you are prompted to input \( a_{2x} \) using a given formula box.
### Additional Note:
To solve this problem, consider Newton's laws of motion, constraints imposed by the system geometry, and how forces like tension and gravity affect the motion of both blocks.
)
**Figure Explanation:**
The figure illustrates a scenario with two blocks connected by a string over a pulley.
- **Block 2** is on an inclined plane, angled at \( \theta \), indicating the slope relative to the horizontal.
- **Block 1** is hanging vertically on the other side of the pulley.
- The pulley is represented as massless and frictionless, meaning it does not add any additional forces or resistance to the system.
- The string connecting the two blocks is also considered massless.
This setup is used to analyze the dynamics of the system by applying Newton's 2nd law, focusing on the forces and acceleration involved.](https://content.bartleby.com/qna-images/question/f0fdd9be-9990-44a9-8005-60989cbb2f12/e7073e7a-f159-49ce-9c14-8f2cbd76872e/ztgups_processed.png)
Transcribed Image Text:**Learning Goal:**
Once you have decided to solve a problem using Newton's 2nd law, there are steps that will lead you to a solution. One such prescription is the following:
- Visualize the problem and identify special cases.
- Isolate each body and draw the forces acting on it.
- Choose a coordinate system for each body.
- Apply Newton’s 2nd law to each body.
- Write equations for the constraints and other given information.
- Solve the resulting equations symbolically.
- Check that your answer has the correct dimensions and satisfies special cases.
- If numbers are given in the problem, plug them in and check that the answer makes sense.
- Think about generalizations or simplifications of the problem.
As an example, we will apply this procedure to find the acceleration of a block of mass \( m_2 \) that is pulled up a frictionless plane inclined at angle \( \theta \) with respect to the horizontal by a massless string that passes over a massless, frictionless pulley to a block of mass \( m_1 \) that is hanging vertically. ([Figure 1](#))
**Figure Explanation:**
The figure illustrates a scenario with two blocks connected by a string over a pulley.
- **Block 2** is on an inclined plane, angled at \( \theta \), indicating the slope relative to the horizontal.
- **Block 1** is hanging vertically on the other side of the pulley.
- The pulley is represented as massless and frictionless, meaning it does not add any additional forces or resistance to the system.
- The string connecting the two blocks is also considered massless.
This setup is used to analyze the dynamics of the system by applying Newton's 2nd law, focusing on the forces and acceleration involved.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 3 steps with 3 images
Follow-up Questions
Read through expert solutions to related follow-up questions below.
Follow-up Question
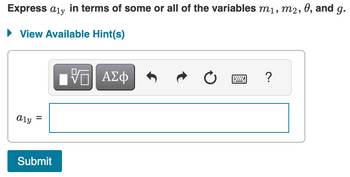
Transcribed Image Text:### Problem Statement
Express \( a_{1y} \) in terms of some or all of the variables \( m_1, m_2, \theta, \) and \( g \).
### Interface Elements
- **View Available Hint(s):** Button to display any hints available to solve the problem.
- **Input Area**: An input box labeled \( a_{1y} = \) where you can enter your answer.
- **Toolbar Options:**
- Square root and absolute value symbol.
- Greek letters and symbols option.
- Undo and redo buttons for correcting input.
- Keyboard icon for virtual keyboard access.
- **Submit Button**: Click to submit your answer.
### How to Approach
- Analyze the relationship between the given variables \( m_1, m_2, \theta, \) and \( g \).
- Use physical laws or mathematical equations relevant to the context (possibly Newton's second law, trigonometry, etc.) to express \( a_{1y} \).
- Input your derived expression in the box provided and submit it.
### Additional Notes
Make sure to consider all possible influences of the provided variables on \( a_{1y} \) and check for any simplifications.
Solution
by Bartleby Expert
Follow-up Questions
Read through expert solutions to related follow-up questions below.
Follow-up Question
Transcribed Image Text:### Problem Statement
Express \( a_{1y} \) in terms of some or all of the variables \( m_1, m_2, \theta, \) and \( g \).
### Interface Elements
- **View Available Hint(s):** Button to display any hints available to solve the problem.
- **Input Area**: An input box labeled \( a_{1y} = \) where you can enter your answer.
- **Toolbar Options:**
- Square root and absolute value symbol.
- Greek letters and symbols option.
- Undo and redo buttons for correcting input.
- Keyboard icon for virtual keyboard access.
- **Submit Button**: Click to submit your answer.
### How to Approach
- Analyze the relationship between the given variables \( m_1, m_2, \theta, \) and \( g \).
- Use physical laws or mathematical equations relevant to the context (possibly Newton's second law, trigonometry, etc.) to express \( a_{1y} \).
- Input your derived expression in the box provided and submit it.
### Additional Notes
Make sure to consider all possible influences of the provided variables on \( a_{1y} \) and check for any simplifications.
Solution
by Bartleby Expert
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.Similar questions
- Two blocks are connected by a massless rope. The rope passes over an ideal (frictionless and massless) pulley such that one block with mass m1 = 12.25 kg is on a horizontal table and the other block with mass m2 = 7.5 kg hangs vertically. Both blocks experience gravity and the tension force, T. Use the coordinate system specified in the diagram. a.) Assuming friction forces are negligible, write an expression, using only the variables provided, for the acceleration that the block of mass m1 experiences in the x-direction. Your answer should involve the tension, T. b.) Under the same assumptions, write an expression for the acceleration, a2, the block of mass m2 experiences in the y-direction. Your answer should be in terms of the tension, T and m2. c.) Carefully consider how the accelerations a1 and a2 are related. Solve for the magnitude of the acceleration, a1, of the block of mass m1, in meters per square second. d.)Find the magnitude of the tension in the rope, T, in newtons.arrow_forwardA 13.0-kg bucket is lowered vertically by a rope in which there is 150 N of tension at a given instant. Part A What is the acceleration of the bucket? Express your answer using two significant figures.arrow_forwardUD MY UTEP ← → C User Settings X Document Sharing A Mail-Alvarado, X mylabmastering.pearson.com/?courseld=12473856&key=14018101051816159781032023#/ Two packages at UPS start sliding down the 20.0° ramp shown in (Figure 1). Package A has a mass of 2.00 kg and a coefficient of kinetic friction of 0.350. Package B has a mass of 10.0 kg and a coefficient of kinetic friction of 0.110. Type here to search Blackboard Learr x PCourse Home X ℗ 7.3.3: A Strategy × b Answered: Two * G Two packages at x + For help with math skills, you may want to review: Vector Components Figure B A 1 of 1 2.00 m 20.0° W How long does it take package A to reach the bottom? Express your answer with the appropriate units. ► View Available Hint(s) At = Submit 0 Provide Feedback μA Value Units ? 74°F Sunny G✩ 4) 0 Next > 5:55 PM 11/3/2023 D x :arrow_forward
- An 80.0 kg spacewalking astronaut pushes off a 660 kg satellite, exerting a 120 N force for the 0.580 s it takes him to straighten his arms. Part A How far apart are the astronaut and the satellite 1.10 min after the push begins? Express your answer with the appropriate units. d=arrow_forwardThree sleds are being pulled horizontally on frictionless horizontal ice using horizontal ropes . The pull is of magnitude 130N. 30.0 kg 20.0 kg 10.0 kg Pull B Part A Find the acceleration of the system. Express your answer with the appropriate units. ANSWER: a = Part B Find the tension in rope A. Express your answer with the appropriate units. ANSWER: TA = %3Darrow_forwardPart A A block lies on a plane raised an angle e fram the horizantal. Three forces act upon the block: Fw, the force of gravity. Fn. the narmal force; and Fr. the force of friction. The coefficient of friction is large enaugh to prevent the block from sliding (Figure 1). Consider coordinate system a, with the x axis along e plane. Which forces lie along the axes? O Ée arly O F, anly O F. only O ři and F. O ř and F. O řa and F. O ř and řa and ř. Submit Requect Ancwor Part B Which forces lie along the axes af the coordinate system b, in which the y axis is vertical? O F anly O F. anly O F. only O F and P, O ř and F. O F, and F. O řr and Fa and Ě, Submit Request Answer Usually the best advice is to choose a coordinate system so that the acceleration of the system is directly along one of the coordinate axes. If the system isn't accelerating, then you are better off choosing the coordinate system with the most vectors along the coordinate axes. But now you are gaing to ignore that advice.…arrow_forward
- A marble is rolling on a table at a constant speed. At the same moment that it rolls off the edge of the table, a penny just drops off the edge. What will happen to them as they fall to the floor? Part A Both will land at the same time. The marble will land first because it is heavier. The marble will land first because of its greater speed. The penny will land first because it is lighter.arrow_forwardThe graphs below show the position d of a puck plotted as a function of time t for 3 pucks moving across a smooth horizontal plane. Select the case that identifies the puck(s) experiencing equilibrium. II O A. I and II only O B. || only OC.I and III only O D.I only O E. I, II, and IIarrow_forwardg force is max gravetional forcearrow_forward
- C Example 10 Three boxes are hung from a pulley system as shown in the following diagram. If box A has a mass of 25 kg, box B has a mass of 40 kg, and box C has a mass of 35 kg, what is the acceleration of the boxes? (cord can't be stretched, frictionless pulley. Both cord and pulley have negligible mass) B Carrow_forward9 For part 3, one group of students were given the following values m₁ = 75 g, m3 =70 g, and 01-55.0°. What should be their m2 value? Round your answer to an integer number. Your Answer: 12arrow_forwardwhat is the acceleration of M1 what is the acceleration of M2arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
- College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
University Physics (14th Edition)PhysicsISBN:9780133969290Author:Hugh D. Young, Roger A. FreedmanPublisher:PEARSON
Introduction To Quantum MechanicsPhysicsISBN:9781107189638Author:Griffiths, David J., Schroeter, Darrell F.Publisher:Cambridge University Press 
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Lecture- Tutorials for Introductory AstronomyPhysicsISBN:9780321820464Author:Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina BrissendenPublisher:Addison-Wesley
College Physics: A Strategic Approach (4th Editio...PhysicsISBN:9780134609034Author:Randall D. Knight (Professor Emeritus), Brian Jones, Stuart FieldPublisher:PEARSON
College Physics
Physics
ISBN:9781305952300
Author:Raymond A. Serway, Chris Vuille
Publisher:Cengage Learning
University Physics (14th Edition)
Physics
ISBN:9780133969290
Author:Hugh D. Young, Roger A. Freedman
Publisher:PEARSON
Introduction To Quantum Mechanics
Physics
ISBN:9781107189638
Author:Griffiths, David J., Schroeter, Darrell F.
Publisher:Cambridge University Press
Physics for Scientists and Engineers
Physics
ISBN:9781337553278
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
Lecture- Tutorials for Introductory Astronomy
Physics
ISBN:9780321820464
Author:Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina Brissenden
Publisher:Addison-Wesley
College Physics: A Strategic Approach (4th Editio...
Physics
ISBN:9780134609034
Author:Randall D. Knight (Professor Emeritus), Brian Jones, Stuart Field
Publisher:PEARSON