A. DATA FOR: Pupal Mass Sample Mass (g) Development Time (Days) 0.688 65 0.688 66 Frequency Distribution of Pupal Mass (g) 0.658 64 0.69 66 10 0.612 60 0.625 59 0.685 59 10 0.682 59 11 0.689 59 12 10 0.689 62 13 11 0.602 70 14 12 0.828 71 15 13 0.805 70 16 14 0.888 72 17 15 0.805 64 0.60 0.72 0.66 0.78 0.84 0.90 18 15 0.822 64 19 16 0.601 64 Pupal Mass (g) 20 18 0.766 64 21 19 0.772 65 22 20 0.755 65 Frequency Distribution of Development Time 23 21 0.756 65 20 24 22 0.743 65 25 23 0.798 65 26 24 0.773 65 15 27 25 0.773 65 28 25 0.771 65 29 26 0.60 66 10 30 28 0.805 66 31 29 0.885 66 32 30 0.801 66 33 34 mean =AVERAGE(range) median =MEDIAN(range) mode =MODE(range) 35 55.00 59.00 63.00 67.00 71.00 75.00 36 37 Development Time (Days) 38 standard deviation =STDEV(range) variance =VAR(range) coefficient of variation (SDimean) covariance =COVAR(range 1, range 2) 40 Development Time (Days) vs. Mass (g) 41 80 correlation coefficient =CORREL(range 1, range 2) 42 43 44 45 46 47 48 50 20 51 52 53 54 0.65 0.7 0.75 0.8 0.85 55 Mass (g) 55 57 Deveko pme nt Time (Days) Aoonba kosanba

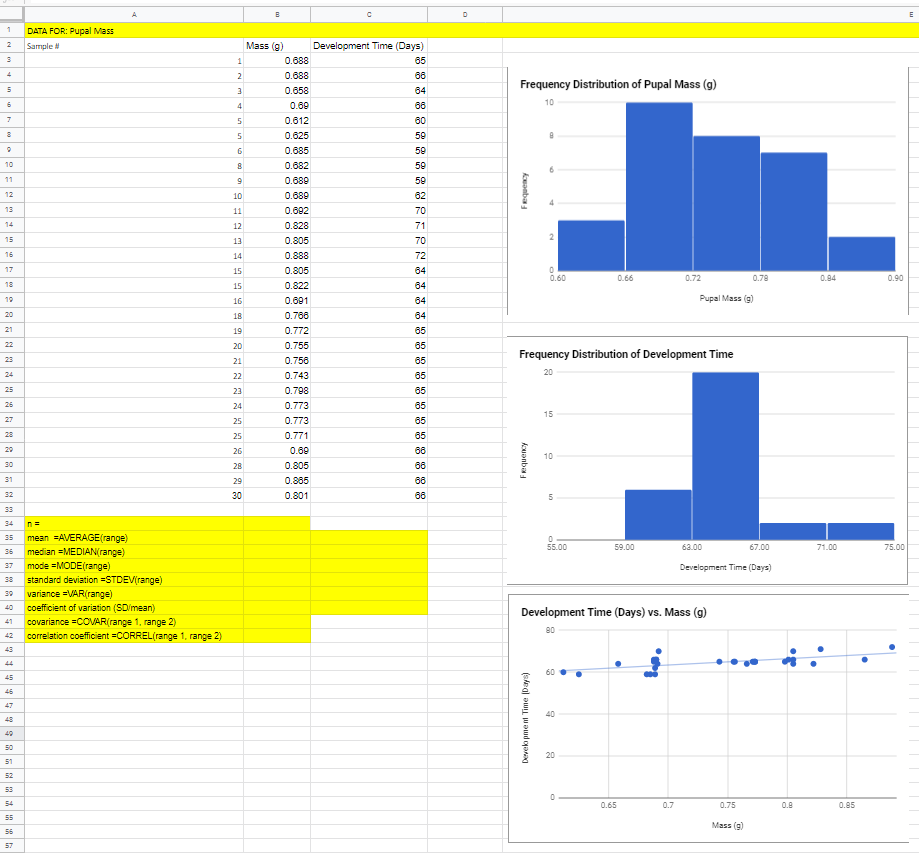
Doctors Jonert and Eckson were excited about their results of their previous study on Gypsy moth development. They decided to continue their study of moths, expanding it to native Virginia moths to learn about the pupal mass relationship to development.  As part of their study they have a colony of Pink-striped Oakworm moths that have been raised in a controlled environment in their labs. They have collected several pieces of data about the male Luna moths in their colony in order to determine if the pupal mass of the males was related to their rate of development.

17) Looking at the graph on Pupal Mass vs Development Time, what type of graph is this?  (pie chart, column chart, bar chart, histogram, line graph, scatter plot, area chart.)

18) Using the appropriate formula, calculate the covariance to 3 decimals. covariance = , now convert it to a percentage: %

19) Is the covariance positive, negative or zero? . 

20) Are the variables dependent on one another?  (yes, no)

21) Using the appropriate formula, calculate the correlation coefficient to 3 decimals: r = 

22) Is the correlation positive, negative or zero?  

23) The null hypothesis was that development time has no effect on pupal mass. More stats need to be done, but based on the data collected so far, does it appear that they will reject the null? (yes or no) 

24) Which program did you use for your calculations?  (Excel or Sheets)