Question
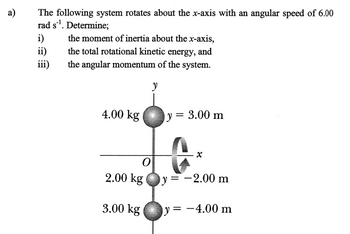
Transcribed Image Text:a)
The following system rotates about the x-axis with an angular speed of 6.00
rad s¹. Determine;
i)
ii)
iii)
the moment of inertia about the x-axis,
the total rotational kinetic energy, and
the angular momentum of the system.
y
4.00 kg
0
2.00 kg
3.00 kg
y = 3.00 m
C
y = -2.00 m
y = -4.00 m
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 3 steps with 1 images

Knowledge Booster
Similar questions
- #7arrow_forward19) A playground merry-go-round with a radius of 1.53 m and a rotational inertia of 103.3 kgm2 is stationary. A ogre with a mass of 30.3 kg gets on and walks around the edge of the merry-go-round. How many revolutions around the merry-go-round must the ogre make in order for the merry-go-round to make two full revolutions? a) 150revolutions b) 9.1 revolutions c) 2.9 revolutions d) 0.7 revolutions e) 4.6 revolutionsarrow_forwardA particle of mass 0.300 kg is attached to the 100-cm mark of a meterstick of mass 0.150 kg. The meterstick rotates on the surface of a frictionless, horizontal table with an angular speed of 6.00 rad/s. a) Calculate the angular momentum of the system when the stick is pivoted about an axis perpendicular to the table through the 50.0-cm mark. b) Calculate the angular momentum of the system when the stick is pivoted about an axis perpendicular to the table through the 0-cm mark.arrow_forward
- Diving from a high cliff, Cliff begins with a moderate rotational speed of a half rotation per second. Rank the following positions (and their moments of inertia) that cliff can assume during his dive, from the lowest rotational speed he will experience to the highest. + 1. Standing straight up, spinning about a head-to-toe axis: I = 0.3 kg m2 + 2. Bent in half, about an axis through his waist: I = 4 kg m- %3D + 3. Standing straight upwards, about an axis through his waist: I = 16 kg m2 + 4. Mass tucked inwards into a ball: I = 0.8 kg m- + 3. Arms out, spinning about a head-to-toe axis: I = 1.2 kg m2arrow_forwardii) A uniform sphere made of modeling clay has radius R and moment of inertia I₁ for rotation about a diameter. It is then flattened to a disk with the same radius R. What is the moment of inertia of the disk, Id, in terms of I₁? a. Id=511/4 b Id = 511/2 c. Id = 511 d. Id = 411/5 e. Id = 211/5 f. Id = I1/5arrow_forwardFind the moment of inertia for a solid sphere that rotates about a point on its surface. a) 3/5 MR^2 b) 3/2 MR^2 c) 5/3 MR^2 d) 7/5 MR^2arrow_forward
arrow_back_ios
arrow_forward_ios