
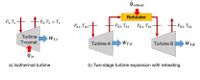
A steady-flow system operating on the ideal Ericsson cycle can achieve the Carnot efficiency. One of the salient features of this cycle is the isothermal expansion through a heated turbine, as shown in Fig. a. Heat is added to the turbine such that the outlet temperature is equal to the inlet temperature, i.e. T₁=T₂, and the overall pressure ratio for this process is rₚ=P₁/P₂.
However, an isothermal expansion through a heated turbine is very difficult to achieve in practical engineering applications. An alternate approach is two use a multi-stage expansion process with reheating. In this approach, the gas expansion process is carried out in multiple stages with reheating in between each stage. Consider the two-stage turbine expansion with reheating shown in Fig. b. The gas enters the first stage of the turbine at state A1, is expanded isentropically to an intermediate pressure Pₐ₂, is reheated at constant pressure to state B1, and is expanded in the second stage isentropically to the final pressure Pᵦ₂. The overall pressure ratio remains Pₐ₁/Pᵦ₂=rₚ. In this concept, the work output is minimized if the gas is reheated to the initial temperature Tᵦ₁=Tₐ₁, and the pressure ratio is equal across each turbine stage such that Pₐ₁/Pₐ₂=Pᵦ₁/Pᵦ₂=√rₚ.
For the same inlet temperature T₁=Tₐ₁, the same mass flow rate m˙, the same overall pressure ratio rₚ, and an ideal monoatomic gas as the working fluid for both scenarios.
Show that for the conditions given, the ratio of the total power output of the ideal two-stage expansion to the total power output of the ideal isothermal expansion is given as: (Picture 2)
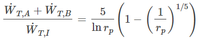
Step by stepSolved in 4 steps

- Consider a steam power plant that operates on the ideal reheat Rankine cycle as shown in Fig. Q5. The plant maintains the boiler at 7000 kPa, the reheat section at 800 kPa, and the condenser at 10 kPa. The mixture quality at the exit of both turbines is 93 percent. Determine the temperature at the inlet of each -1 turbine and the cycle's thermal efficiency. Reheater Boiler High-P turbine Pump Low-P turbine Condenserarrow_forwardAnswer this mcq urgentarrow_forwardA steady-flow system operating on the ideal Ericsson cycle can achieve the Carnot efficiency. One of the salient features of this cycle is the isothermal expansion through a heated turbine, as shown in Fig. a. Heat is added to the turbine such that the outlet temperature is equal to the inlet temperature, i.e. T₁=T₂, and the overall pressure ratio for this process is rₚ=P₁/P₂. However, an isothermal expansion through a heated turbine is very difficult to achieve in practical engineering applications. An alternate approach is two use a multi-stage expansion process with reheating. In this approach, the gas expansion process is carried out in multiple stages with reheating in between each stage. Consider the two-stage turbine expansion with reheating shown in Fig. b. The gas enters the first stage of the turbine at state A1, is expanded isentropically to an intermediate pressure Pₐ₂, is reheated at constant pressure to state B1, and is expanded in the second stage isentropically to the…arrow_forward
 Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press
Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON
Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education
Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY
Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY
Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY





