A recent article published by the Wall Street Journal said the Cincinnati airport had the most expensive airfares among the largest airports in the US. While they had some statistics to support this statement, a statistician decided to investigate further. A sample of 46 flights originating in Cincinnati was selected, and the round-trip airfare for each flight was recorded. The sample data is summarized in the StatCrunch output below: With 98% confidence, we estimate the mean airfare for flights out of Cincinnati is between $ and $. (Round the limits of your interval to 4 decimal places.) The mean airfare nationwide was reported as $376 for a round-trip ticket. Does the interval found above support the claim that Cincinnati has airfares which are more expensive than other airports in the US? The interval does not support the claim because the entire interval is not above $376. We can't make this conclusion based on a confidence interval. The interval does not support this claim because an airfare of $376 does not fall inside the interval. The interval supports the claim because the entire interval is greater than $376. You need to schedule a flight for the summer. If you want to estimate the cost of your flight, which interval would be most appropriate for estimating the cost? an interval based on the Empirical Rule or Chebyshev's Rule (depending on the shape of the data) the confidence interval Suppose the sample selected was taken so that the 46 most expensive flights from CVG were selected. Which assumption has been violated? normality random sample quantitative data

Name: A recent article published by the Wall Street Journal said the Cincinn
Uploaded: 2024-03-20T07:07:00.000Z
Channel: Bartleby
Description: A recent article published by the Wall Street Journal said the Cincinnati airport had the most expensive airfares among the largest airports in the US. While they had some statistics to support this statement, a statistician decided to investigate fu

Given : Data on sample of 46 flights originating in cincinnati and roundup trip airfare for each…

Answered: A recent article published by the Wall Street Journal said the Cincinnati airport had the most expensive airfares among the largest airports in the US. While…

MATLAB: An Introduction with Applications

6th Edition

ISBN: 9781119256830

Author: Amos Gilat

Publisher: John Wiley & Sons Inc

See similar textbooks

Concept explainers

2-Proportion Test

In a 2-proportion test, two separate proportions are measured at the same time, and their similarity is evaluated. It is also known as a 2-proportion z-test, and a comparison of two different proportions is done in this method.

Topic Video

Question

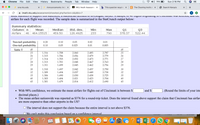
A recent article published by the Wall Street Journal said the Cincinnati airport had the most expensive airfares among the largest airports in the US. While they had some statistics to support this statement, a statistician decided to investigate further. A sample of 46 flights originating in Cincinnati was selected, and the round-trip airfare for each flight was recorded. The sample data is summarized in the StatCrunch output below:

With 98% confidence, we estimate the mean airfare for flights out of Cincinnati is between $ and $. (Round the limits of your interval to 4 decimal places.)
The mean airfare nationwide was reported as $376 for a round-trip ticket. Does the interval found above support the claim that Cincinnati has airfares which are more expensive than other airports in the US?
- The interval does not support the claim because the entire interval is not above $376.
- We can't make this conclusion based on a confidence interval.
- The interval does not support this claim because an airfare of $376 does not fall inside the interval.
- The interval supports the claim because the entire interval is greater than $376.
You need to schedule a flight for the summer. If you want to estimate the cost of your flight, which interval would be most appropriate for estimating the cost?
- an interval based on the Empirical Rule or Chebyshev's Rule (depending on the shape of the data)
- the confidence interval
Suppose the sample selected was taken so that the 46 most expensive flights from CVG were selected. Which assumption has been violated?
- normality
- random sample
- quantitative data

Chrome
File
Edit
View
History Bookmarks
Реople
Tab
Window
Help
)) 75%
Sun 2:16 PM
one.nku.edu
Announcements: Indu X
NKU Imath Assessme X
b This question requires X
C The Cleaning Institute x
Search Marquis
x +
imath.nku.edu/assessment/showtest.php?action=skip&to=7
a
SOIIC SlatistIcs LU SUPPOIT IIIS SlATCIICIIl, a StatiISTICIAll UCCIUcu LU IIVCStigaio TuI ICI, A Sallipic UI T0 IIiglis OIigllatillg III CIIC Mati yy AS SCICUICU, allu NC
airfare for each flight was recorded. The sample data is summarized in the StatCrunch output below:
Summary statistics:
Column n
Mean
Median
Std. dev.
Min
Маx
Q1
378.37
Q3
Airfare
46 464.15515
459.50
126.4925
233
790
522.44
Two-tail probability
One-tail probability
0.20
0.10
0.05
0.02
0.01
0.10
0.05
0.025
0.01
0.005
Table T
df
df
25
1.316
1.708
2.060
2.485
2.787
25
26
1.315
1.706
2.056
2.479
2.779
26
27
1.314
1.703
2.052
2.473
2.771
27
28
1.313
1.701
2.048
2.467
2.763
28
29
1.311
1.699
2.045
2.462
2.756
29
30
1.310
1.697
2.042
2.457
2.750
30
32
1.309
1.694
2.037
2.449
2.738
32
35
1.306
1.690
2.030
2.438
2.725
35
40
1.303
1.684
2.021
2.423
2.704
40
45
1.301
1.679
2.014
2.412
2.690
45
• With 98% confidence, we estimate the mean airfare for flights out of Cincinnati is between $
decimal places.)
• The mean airfare nationwide was reported as $376 for a round-trip ticket. Does the interval found above support the claim that Cincinnati has airfar
are more expensive than other airports in the US?
and $
(Round the limits of your int=
The interval does not support the claim because the entire interval is not above $376.
We can't make this conclusion based on a confidence interval
TIIvacy
TC IS
38
OCT
? 25
?
?
W
•..
lil

Definition Definition Measure of central tendency that is the average of a given data set. The mean value is evaluated as the quotient of the sum of all observations by the sample size. The mean, in contrast to a median, is affected by extreme values. Very large or very small values can distract the mean from the center of the data. Arithmetic mean: The most common type of mean is the arithmetic mean. It is evaluated using the formula: μ = 1 N ∑ i = 1 N x i Other types of means are the geometric mean, logarithmic mean, and harmonic mean. Geometric mean: The nth root of the product of n observations from a data set is defined as the geometric mean of the set: G = x 1 x 2 ... x n n Logarithmic mean: The difference of the natural logarithms of the two numbers, divided by the difference between the numbers is the logarithmic mean of the two numbers. The logarithmic mean is used particularly in heat transfer and mass transfer. ln x 2 − ln x 1 x 2 − x 1 Harmonic mean: The inverse of the arithmetic mean of the inverses of all the numbers in a data set is the harmonic mean of the data. 1 1 x 1 + 1 x 2 + ...

Expert Solution