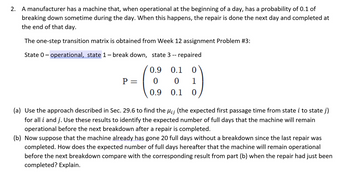
2. A manufacturer has a machine that, when operational at the beginning of a day, has a probability of 0.1 ofbreaking down sometime during the day. When this happens, the repair is done the next day and completed atthe end of that day.The one-step transition matrix is obtained from Week 12 assignment Problem #3:State O-operational, state 1 – break down, state 3 -- repaired0.9 0.1 000 10.90.1 0P =(a) Use the approach described in Sec. 29.6 to find the µ¡¡ (the expected first passage time from state i to state j)for all i and j. Use these results to identify the expected number of full days that the machine will remainoperational before the next breakdown after a repair is completed.(b) Now suppose that the machine already has gone 20 full days without a breakdown since the last repair wascompleted. How does the expected number of full days hereafter that the machine will remain operationalbefore the next breakdown compare with the corresponding result from part (b) when the repair had just beencompleted? Explain.

(a) To find the Mij values, we need to first construct the matrix N as N = (I - P), where I is the…

Answered: A manufacturer has a machine that, when…

MATLAB: An Introduction with Applications

6th Edition

ISBN:9781119256830

Author:Amos Gilat

Publisher:Amos Gilat

Chapter1: Starting With Matlab

Section: Chapter Questions

Problem 1P

See similar textbooks

Similar questions

StarHortons Cup, the coffee company, wants to determine how long caffeine lasts throughout the day when individuals have a cup of one of their 3 types of coffee at 9am. They will then measure the levels of caffeine in these individuals’ systems at 10am, 12am, 2pm, 4pm, 6pm and 8pm. They want to have at least 20 observations per group. How many separate/distinct groups of participants are needed if they want to conduct this experiment using a mixed-measures ANOVA? 18 6 20 3
The owner of a chain of mini-markets wants to compare the sales performance of two of her stores, Store 1 and Store 2. Sales can vary considerably depending on the day of the week and the season of the year, so she decides to eliminate such effects by making sure to record each store's sales on the same 10 days, chosen at random. She records the sales (in dollars) for each store on these days, as shown in the table below. Day 1 3 4 6. 9 10 Store 1 557 829 733 531 454 634 409 726 769 329 Store 2 413 732 567 484 650 586 269 684 705 371 Difference 144 97 166 47 - 196 48 140 42 64 -42 (Store 1- Store 2) Send data to calculator Based on these data, can the owner conclude, at the 0.10 level of significance, that the mean daily sales of the two stores differ? Answer this question by performing a hypothesis test regarding u (which is u with a letter "d" subscript), the population mean daily sales difference between the two stores. Assume that this population of differences (Store 1 minus Store…
The owner of a chain of mini-markets wants to compare the sales performance of two of her stores, Store 1 and Store 2. Sales can vary considerably depending on the day of the week and the season of the year, so she decides to eliminate such effects by making sure to record each store's sales on the same 8 days, chosen at random. She records the sales (in dollars) for each store on these days, as shown in the table below. Day 1 2 3 4 5 6 7 8 Store 1 889 699 534 398 432 213 252 929 Store 2 479 525 252 364 160 32 234 632 Difference(Store 1 - Store 2) 410 174 282 34 272 181 18 297 Send data to calculator Based on these data, can the owner conclude, at the 0.10 level of significance, that the mean daily sales of the two stores differ? Answer this question by performing a hypothesis test regarding μd (which is μ…
The following data consists of a matrix of transition probabilities (P) of three competing companies, the initial market share state ℼ(1), and the equilibrium probability states. Assume that each state represents a firm (Company 1, Company 2, and Company 3, respectively) and the transition probabilities represent changes from one month to the next. The market share of Company 2 after two periods is a. 0.283 b. 0.26 c. 0.261 d. 0.47
A car rental company has two locations. Each week, 80% of the cars rented at location A are returned to location A and the rest location B. Of the cars rented at location B, 30% are returned to location B by the end of the week and the rest to location A. a. Make a transition diagram for this process. b. Write the transition matrix T for this process. cIf 50% of the company's cars start this week at location A, and 50% at location B, find the proportion of cars that will be at each location one week later Label your answers. d. Write and solve a system of equations to find the stable distribution (correct to 3 decimal places) for this Markov process. Show all calculations and label the row operations.
QUESTION 5 A small assembly plant has 100 employees. Each worker is expected to complete work assignments on time and in such a way that the assembled product will pass a final inspection. On occasions, some of the workers fail to meet the performance standards by completing work late and/or assembling a defective product. At the end of a performance evaluation period, the production manager found that 10 workers had completed work late, 20 workers had assembled a defective product, and 8 workers had both completed work late and assembled a defective product. 1. What is the probability that a worker chosen at random had completed work late? 2. What is the probability that a worker chosen at random had assembled a defective product? 3. What is the probability that a worker chosen at random had completed work late and assembled a defective product? 4. What is the probability that a worker chosen at random had completed work late or assembled a defective product?
Scenario 3. A researcher creates a new program for college students to reduce feelings of loneliness and enhance students' engagement on campus. This new program helps students to find student groups and clubs that fit their interests. She randomly assigns students to either enroll in this new program (the experimental group) or to not enroll in the program (the control group). She compares students' feelings of engagement between the two groups, and expects that engagement will be higher among the students that are in the program. What is the most appropriate test statistic to use to test the hypothesis in scenario 3? A) z-test B) z-score C) Independent samples t-Test D) Correlation Coefficient E) One-way ANOVA F) Dependent samples t-Test G) Factor Analysis H) Structural Equation Model What is the null hypothesis for scenario 3? A) H0: engagement in the experimental group ≤ engagement in the control group B) H0 : engagement in the experimental group = engagement in the control…
In an office complex of 1000 employees, on any given day some are at work and the rest are absent. It is known that if an employee is at work today, there is an 85% chance that she will be at work tomorrow, and if the employee is absent today, there is a 70% chance that she will be absent tomorrow. Suppose that today there are 740 employees at work. (A graphing calculator is recommended.) (a) Find the transition matrix A for this scenario. at work absent A = (b) Predict the number that will be at work five days from now. (Round your answer to the nearest integer.) employees (c) Find the steady-state vector x. (Round your answer to two decimal places.)
A study conducted by the Urban Energy Commission in a large metropolitan area indicates the probabilities that homeowners within the area will use certain heating fuels or solar energy during the next 10 years as the major source of heat for their homes. The following transition matrix represents the transition probabilities from one state to another. Electricity 10.2 Natural Gas Fuel Oil Solar Energy Elec. Gas Oil 0.60 0.05 0.10 0.15 0.85 0.10 0.08 Solar 0 0.10 0.02 0.75 0.08 0.15 0.08 0.05 0.84 Among homeowners within the area, 20% currently use electricity, 35% use natural gas, 40% use oil, and 5% use solar energy as the major source of heat for their homes. In the long run, percentage of homeowners within the area will be using solar energy as their major source of heating fuel? (Round your answer to one decimal place. Assume the trend continues.) X % what
In any given day the air quality in a certain city is either good or bad. Records show that when the air quality is good on one day, then there is a 95% chance that it will be good the next day, and when the air quality is bad on one day, then there is 45% chance it will be bad the next day. a. Give the transition matrix. b. if the air quality is good today, what is the probability it will be good two days from now? c. if the air quality is bad today, what is the probability it will be bad three days from now? d. if the there is 20% chance the air quality is good today, what is the probability it will be good tomorrow?
.1 Certify X gin in a new win... ing.com/Portal/Lesson/lesson_certify Week 8 - FIN-341- Question 1 of 11, Step 1 of 1 Answer X Week 1- BIO-165-5... - Save & Exit Certify Lesson: 6.1 Solving Systems of Linear E... Ⓒ2022 Hawkes Learning X My Citation list 6/2 x W C My books Doxy Leona Doxy Kelsey Selecting an option will display any text boxes needed to complete your answer. How can derivative X 0/11 Correct Use any convenient method to solve the following system of equations. If the system is dependent, express the solution set in terms of one of the variables. Leave all fractional answers in fraction form. = -1 x + 2y y-32= 131 -4x - 6y + 6z = 30 Consumers and Th X 4 Digital Content | Up... O Only One Solution O Inconsistent System O Dependent System b Success Confirmat Textbook Solutions REBECCA BOONE Tables Keypad Keyboard Shortcuts Submit Answer

Recommended textbooks for you

MATLAB: An Introduction with Applications

Statistics

ISBN:

9781119256830

Author:

Amos Gilat

Publisher:

John Wiley & Sons Inc

Probability and Statistics for Engineering and th…

Statistics

ISBN:

9781305251809

Author:

Jay L. Devore

Publisher:

Cengage Learning

Statistics for The Behavioral Sciences (MindTap C…

Statistics

ISBN:

9781305504912

Author:

Frederick J Gravetter, Larry B. Wallnau

Publisher:

Cengage Learning

MATLAB: An Introduction with Applications

Statistics

ISBN:

9781119256830

Author:

Amos Gilat

Publisher:

John Wiley & Sons Inc

Probability and Statistics for Engineering and th…

Statistics

ISBN:

9781305251809

Author:

Jay L. Devore

Publisher:

Cengage Learning

Statistics for The Behavioral Sciences (MindTap C…

Statistics

ISBN:

9781305504912

Author:

Frederick J Gravetter, Larry B. Wallnau

Publisher:

Cengage Learning

Elementary Statistics: Picturing the World (7th E…

Statistics

ISBN:

9780134683416

Author:

Ron Larson, Betsy Farber

Publisher:

PEARSON

The Basic Practice of Statistics

Statistics

ISBN:

9781319042578

Author:

David S. Moore, William I. Notz, Michael A. Fligner

Publisher:

W. H. Freeman

Introduction to the Practice of Statistics

Statistics

ISBN:

9781319013387

Author:

David S. Moore, George P. McCabe, Bruce A. Craig

Publisher:

W. H. Freeman

SEE MORE TEXTBOOKS