MATLAB: An Introduction with Applications
6th Edition
ISBN: 9781119256830
Author: Amos Gilat
Publisher: John Wiley & Sons Inc
expand_more
expand_more
format_list_bulleted
Related questions
Question
![## Statistical Calculations and Hypothesis Testing
### Consider the following data:
\[
\begin{array}{|c|c|c|c|c|c|}
\hline
x_i & 3 & 12 & 6 & 20 & 14 \\
\hline
y_i & 55 & 40 & 55 & 5 & 15 \\
\hline
\end{array}
\]
### (a) Mean Square Error (MSE)
Compute the mean square error using the equation:
\[ s^2 = \text{MSE} = \frac{\text{SSE}}{n - 2} \]
- Result: **72.92**
### (b) Standard Error of the Estimate
Compute the standard error of the estimate using the equation:
\[ s = \sqrt{\text{MSE}} = \sqrt{\frac{\text{SSE}}{n - 2}} \]
- Result: **8.539**
### (c) Estimated Standard Deviation of \( b_1 \)
Compute the estimated standard deviation of \( b_1 \) using the equation:
\[ s_{b_1} = \frac{s}{\sqrt{\sum(x_i - \bar{x})^2}} \]
- Result: **0.636**
### (d) Hypothesis Testing
Use the t-test to evaluate the following hypotheses (\(\alpha = 0.05\)):
- \( H_0: \beta_1 = 0 \)
- \( H_a: \beta_1 \neq 0 \)
#### Test Statistic
- Value: **-5.106**
#### p-value
- Result: **0.0145**
#### Conclusion
- **Reject \( H_0 \). We conclude that the relationship between x and y is significant.**](https://content.bartleby.com/qna-images/question/f7b76bbb-1122-4c75-9c9e-2038b7c47d4e/a3298a90-94ab-4279-a1fe-47a52036f8df/hcz3gfj_processed.png)
Transcribed Image Text:## Statistical Calculations and Hypothesis Testing
### Consider the following data:
\[
\begin{array}{|c|c|c|c|c|c|}
\hline
x_i & 3 & 12 & 6 & 20 & 14 \\
\hline
y_i & 55 & 40 & 55 & 5 & 15 \\
\hline
\end{array}
\]
### (a) Mean Square Error (MSE)
Compute the mean square error using the equation:
\[ s^2 = \text{MSE} = \frac{\text{SSE}}{n - 2} \]
- Result: **72.92**
### (b) Standard Error of the Estimate
Compute the standard error of the estimate using the equation:
\[ s = \sqrt{\text{MSE}} = \sqrt{\frac{\text{SSE}}{n - 2}} \]
- Result: **8.539**
### (c) Estimated Standard Deviation of \( b_1 \)
Compute the estimated standard deviation of \( b_1 \) using the equation:
\[ s_{b_1} = \frac{s}{\sqrt{\sum(x_i - \bar{x})^2}} \]
- Result: **0.636**
### (d) Hypothesis Testing
Use the t-test to evaluate the following hypotheses (\(\alpha = 0.05\)):
- \( H_0: \beta_1 = 0 \)
- \( H_a: \beta_1 \neq 0 \)
#### Test Statistic
- Value: **-5.106**
#### p-value
- Result: **0.0145**
#### Conclusion
- **Reject \( H_0 \). We conclude that the relationship between x and y is significant.**
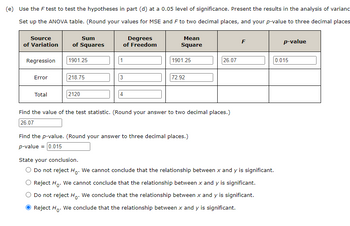
Transcribed Image Text:**ANOVA Table for Hypothesis Testing**
**Objective**: Use the F test to assess the hypotheses at a 0.05 level of significance. This involves an analysis of variance (ANOVA) table.
### ANOVA Table
| Source of Variation | Sum of Squares | Degrees of Freedom | Mean Square | F | p-value |
|---------------------|----------------|--------------------|-------------|------|---------|
| Regression | 1901.25 | 1 | 1901.25 | 26.07| 0.015 |
| Error | 218.75 | 3 | 72.92 | | |
| Total | 2120 | 4 | | | |
### Calculations
- **Test Statistic**: \( F = 26.07 \)
- **p-value**: \( p = 0.015 \)
### Conclusion Options
1. Do not reject \( H_0 \). We cannot conclude that the relationship between \( x \) and \( y \) is significant.
2. Reject \( H_0 \). We cannot conclude that the relationship between \( x \) and \( y \) is significant.
3. Do not reject \( H_0 \). We conclude that the relationship between \( x \) and \( y \) is significant.
4. **Reject \( H_0 \). We conclude that the relationship between \( x \) and \( y \) is significant.**
**Selected Conclusion**: Option 4 is chosen, indicating that there is a significant relationship between \( x \) and \( y \) since the p-value (0.015) is less than the significance level (0.05).
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 4 steps with 42 images
Knowledge Booster
Similar questions
- The systolic blood pressure (given in millimeters) of males has an approximately normal distribution with mean u = 125 and standard deviation o = 12. (a) Calculate the z-scores for the male systolic blood pressures 110 and 140 millimeters. (Round your answers to two decimal places.) 110 mm z = 140 mm z = (b) If a male friend of yours said he thought his systolic blood pressure was 2.5 standard deviations below the mean, but that he believed his blood pressure was between 110 and 140 millimeters, what would you say to him? (Enter your numerical answer to the nearest whole number.) He is ---Select--- because 2.5 standard deviations below the mean would give him a blood pressure reading of millimeters, which is ---Select--- the range of 110 to 140 millimeters.arrow_forwardIn the past, a chemical company produced 880 pounds of a certain type of plastic per day. Now, using a newly developed and less expensive process, the mean daily yield of plastic for the first 50 days of production is 871 pounds; the standard deviation is 21 pounds. Do the data provide sufficient evidence to indicate that the mean daily yield for the new process is less than that of the old procedure? (Use α=0.05) (a) Alternative hypothesis for the problem above is μ 871 Ομ 880 <arrow_forwardIn the past, a chemical company produced 880 pounds of a certain type of plastic per day. Now, using a newly developed and less expensive process, the mean daily yield of plastic for the first 50 days of production is 871 pounds; the standard deviation is 21 pounds. Do the data provide sufficient evidence to indicate that the mean daily yield for the new process is less than that of the old procedure? (Use α=0.05) (c) p-value for the test above isarrow_forward
- A random sample of 25 night students was taken with a sample mean GPA of 2.86 and a standard deviation of 0.06. A random sample of 30 day students was taken with a sample mean GPA of 2.88 and a standard deviation of 0.07. Test the claim that the mean GPA of night students (μ) is different from the mean GPA of day students (μD) at a = 0.05. Assume that the data come from normal populations with unequal variances. Round your answers to three decimal places, and round any interim calculations to four decimal places. Fill in the hypotheses below (click the circle to the left of the correct answer): Ho: ên p σ΄ UN Td μD = v pО µÑ¯ ʵ¯Ñ¿© µD Ha: pOād up μι ên # PO μN X d This test is two-tailed. What is the test statistic? HD ên Part 2 of 4arrow_forwardIn a sample of 12 randomly selected high school seniors, the mean score on a standardized test was 1182 and the standard deviation was 161.6.Further research suggests that the population mean score on this test for high school seniors is 1012. Does the t-value for the original sample fall between −t0.95 and t0.95? Assume that the population of test scores for high school seniors is normally distributed. What is The t-value? And does or does not it not fall between −t0.95 and t0.95 because t0.95=? (Round to two decimal places as needed.)arrow_forwardPulse rates of adult men are approximately normal with a mean of 70 and a standard deviation of 8. Which choice correctly describes how to find the proportion of men that have a pulse rate greater than 78? (a) Find the area to the right of z = -1 under a standard normal curve. (b) Find the area to the right of z=1 under a standard normal curve. (c) Find the area to the left of z = 1 under a standard normal curve. (d) Find the area between z=-1 and z=1 under a standard normal curve. (e) None of the abovearrow_forward
- The population of adult women in the U.S. have a mean foot length of 9.58 inches and a standard deviation of 0.51 inches, which is normally distributed. Using a TI 84 graphing calculator: Calculate P(1 woman has foot length < 9.6 inches) =Calculate P(Group of 22 women have a mean foot length < 9.5 inches) =Calculate P(Group of 6 women have a mean foot length between 9 and 10 inches) Be sure to show calculator commans used.arrow_forwardFrom generation to generation, the mean age when smokers first start to smoke varies. However, the standard deviation of that age remains constant at around 2.1 years. A survey of 38 smokers of this generation was done to see if the mean starting age is at least 19. The sample mean was 18.1 with a sample standard deviation of 1.3. Do the data support the claim at the 5% level? (i) Alpha (Enter an exact number as an integer, fraction, or decimal.)? =arrow_forwardA random sample of 51 adult coyotes in a region of northern Minnesota showed the average age to be x = 2.01 years, with sample standard deviation s = 0.76 years. However, it is thought that the overall population mean age of coyotes is u = 1.75. Do the sample data indicate that coyotes in this region of northern Minnesota tend to live longer than the average of 1.75 years? Use a = 0.01. (a) What is the level of significance? State the null and alternate hypotheses. O Ho: H 1.75 yr; Hi: и 1.75 yr; Hi: и %3D 1.75 yr II Но: и 3 1.75 уr; Hi: и > 1.75 yr Ho: H = 1.75 yr; H1: µ # 1.75 yr (b) What sampling distribution will you use? Explain the rationale for your choice of sampling distribution. O The standard normal, since the sample size is large and o is known. The Student's t, since the sample size is large and o is known. The standard normal, since the sample size is large and o is unknown. O The Student's t, since the sample size is large and o is unknown. What is the value of the…arrow_forward
- Assume that the readings at freezing on a batch of thermometers are normally distributed with a mean of 0°C and a standard deviation of 1.00°C.A single thermometer is randomly selected and tested. Let ZZ represent the reading of this thermometer at freezing. What reading separates the highest 8.92% from the rest? That is, if P(z>c)=0.0892P(z>c)=0.0892, find c.arrow_forwardFor a group of 20 students taking a PSDTF test, the mean heart rate was 96 beats per minute, and the standard deviation was 5. Find the 95% confidence interval of the true mean.arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
- MATLAB: An Introduction with ApplicationsStatisticsISBN:9781119256830Author:Amos GilatPublisher:John Wiley & Sons Inc
Probability and Statistics for Engineering and th...StatisticsISBN:9781305251809Author:Jay L. DevorePublisher:Cengage Learning
Statistics for The Behavioral Sciences (MindTap C...StatisticsISBN:9781305504912Author:Frederick J Gravetter, Larry B. WallnauPublisher:Cengage Learning 
Elementary Statistics: Picturing the World (7th E...StatisticsISBN:9780134683416Author:Ron Larson, Betsy FarberPublisher:PEARSON
The Basic Practice of StatisticsStatisticsISBN:9781319042578Author:David S. Moore, William I. Notz, Michael A. FlignerPublisher:W. H. Freeman
Introduction to the Practice of StatisticsStatisticsISBN:9781319013387Author:David S. Moore, George P. McCabe, Bruce A. CraigPublisher:W. H. Freeman
MATLAB: An Introduction with Applications
Statistics
ISBN:9781119256830
Author:Amos Gilat
Publisher:John Wiley & Sons Inc
Probability and Statistics for Engineering and th...
Statistics
ISBN:9781305251809
Author:Jay L. Devore
Publisher:Cengage Learning
Statistics for The Behavioral Sciences (MindTap C...
Statistics
ISBN:9781305504912
Author:Frederick J Gravetter, Larry B. Wallnau
Publisher:Cengage Learning
Elementary Statistics: Picturing the World (7th E...
Statistics
ISBN:9780134683416
Author:Ron Larson, Betsy Farber
Publisher:PEARSON
The Basic Practice of Statistics
Statistics
ISBN:9781319042578
Author:David S. Moore, William I. Notz, Michael A. Fligner
Publisher:W. H. Freeman
Introduction to the Practice of Statistics
Statistics
ISBN:9781319013387
Author:David S. Moore, George P. McCabe, Bruce A. Craig
Publisher:W. H. Freeman