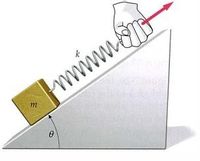
Question
A block of mass m rests on a plane inclined at θ with the horizontal. The block is
attached to a spring of constant k as shown in Figure-2. The coefficients of static and
kinetic friction between the block and plane are and respectively. Very slowly, the
spring is pulled upward along the plane until the block starts to move.
(a) Obtain an expression for the extension d of the spring the instant the block moves.
(b) Determine the value of such that the block comes to rest just as the spring is in its
unstressed condition, that is, neither extended nor compressed.
Transcribed Image Text:wwiw
θ
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 3 steps with 1 images

Knowledge Booster
Similar questions
- A 8.0 kg mass hanging from a spring scale is slowly lowered onto a vertical spring, as shown in (Figure 1). What does the spring scale read just before the mass touches the lower spring? The scale reads 16 NN when the lower spring has been compressed by 1.7 cmcm. What is the value of the spring constant for the lower spring? At what compression length will the scale read zero?arrow_forwardUse the principle of minimum potential energy developed in Section 3.10 to solve the bar problems shown in Figure P3-52. That is, plot the total potential energy for variations in the displacement of the free end of the bar to determine the minimum potential energy. Observe that the displacement that yields the minimum potential energy also yields the stable equilibrium position. Use displacement increments of O.002 in., beginning with x = -0.004. Let E = 30 x 106 psi and A = 2 in2 for the bars. 10,000 Ib 30 in. 10,000 lb 50 in. (b) Figure P3-52arrow_forwardThe images are the setup for this question below. ANSWER THIS QUESTION: The spring on Earth has stiffness 2k and relaxed length L0. When the package is at rest and ready to launch, the spring is maximally compressed. What does this compression have to be in order for the package to reach the launching speed you found in the previous part? Use the variable v to represent the speed found in part 1.arrow_forward
- Learning Goal: To understand the Equipartition Theorem and its implications for the mechanical motion of small objects. In statistical mechanics, thermal energy is the random motion of the microscopic world. The average kinetic or potential energy of each degree of freedom of the microscopic world therefore depends on the temperature. If heat is added, molecules increase their translational and rotational speeds, and the atoms constituting the molecules vibrate with larger amplitude about their equilibrium positions. It is a fact of nature that the energy of each degree of freedom is determined solely by the temperature. The Equipartition Theorem states this quantitatively: The average energy associated with each degree of freedom in a system at absolute temperature T is (1/2)k³T, where KB = : 1.38 × 10-2³ J/K is Boltzmann's constant. A "degree of freedom" corresponds to any dynamical variable that appears quadratically in the energy. For instance, (1/2)mv² is the kinetic energy of a…arrow_forwardA 15 kg block sliding horizontally across the floor has a coefficient of static friction of μs = 0.49 and a coefficient of kinetic friction of μk = 0.34. If it was sliding at an initial speed of 4.2 m/s, how far will it travel before stopping? Be clear and show all your work. (Dynamics)arrow_forwardplease answer all with stepsarrow_forward
- Using the geometrical approach based on areas just described in question 1, you can find the total work done by the approximate force over the displacement between x = 0 and x = x2. What is this approximate work for the case of N = 4?arrow_forwardA box with weight of magnitude FG=2.00N is lowered by a rope down a smooth plane that is inclined at an angle ϕ = 30.0 ∘ above the horizontal, as shown in (Figure 1) at left. The normal force acting on the box has a magnitude n=1.73N the tension force is 1.00 N, and the displacement Δr of the box is 1.80 m down the inclined plane. The work done on the box by gravity is 1.8 J What is the work Wn done on the box by the normal force? What is the work WT done by the tension force?arrow_forwardOnly parts A & B please.arrow_forward
arrow_back_ios
arrow_forward_ios