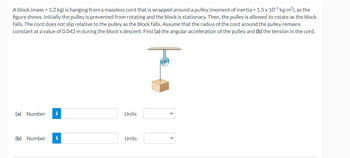
A block (mass = 1.2 kg) is hanging from a massless cord that is wrapped around a pulley (moment of inertia = 1.5 x 10-³ kg-m²), as the figure shows. Initially the pulley is prevented from rotating and the block is stationary. Then, the pulley is allowed to rotate as the block falls. The cord does not slip relative to the pulley as the block falls. Assume that the radius of the cord around the pulley remains constant at a value of 0.042 m during the block's descent. Find (a) the angular acceleration of the pulley and (b) the tension in the cord. (a) Number i (b) Number i Units Units

Problem 2: A pulley consists of a large disk of radius R and a small disk of radius r that are welded together and mounted on a horizontal frictionless axle through their common centers. The moment of inertia of this pulley is I. A box containing a turkey of mass M is suspended from a rope wound around the large disk; a box containing a pumpkin of mass m is suspended from a rope wound around the small disk. The ropes do not slip on the disks. The system is released from rest and the turkey begins to descend, while the pumpkin is being lifted up. M P A Derive an expression for the magnitude of the angular acceleration a of the pulley, in terms of system parameters. Assume that a counterclockwise motion of the pulley is the positive direction. MgR+mgR MgR-mgr MgR-mgR a a = a = I+MR2+mr2 I+MR²+mr² I+MR²+mR? MgR+mgr MgR-mgR MgR+mgR a = a = I+MR2+mr²2 I+MR²+mr² I+MR²+mR? Submit I give up! Hint Feedback

PLEASE DRAW AN FBD AND ANSWER IT ON A PAPER   The figure shown is on a fixed frictionless axle. It has a moment of inertia of I = 50 kg-m2 . The forces acting on the object are F1 = 100 N, F2 = 200 N, and F3 = 250 N acting at different radii R1 =60 cm, R2 = 42 cm, and R3 = 28 cm. Determine the angular acceleration of the object.

A block (mass = 1.7 kg) is hanging from a massless cord that is wrapped around a pulley (moment of inertia = 1.6 x 10-3 kg·m2), as the figure shows. Initially the pulley is prevented from rotating and the block is stationary. Then, the pulley is allowed to rotate as the block falls. The cord does not slip relative to the pulley as the block falls. Assume that the radius of the cord around the pulley remains constant at a value of 0.042 m during the block's descent. Find (a) the angular acceleration of the pulley and (b) the tension in the cord.

A 0.45 kg tetherball is attached to a pole and rotating in a horizontal circle of radius r₁ = 1.4 m and is circling at angular speed = 1.42 rad/s. As the rope wraps around the pole the radius of the circle shortens and became r2 = 0.9 m, that decreases the moment of inertia of the rotating tetherball. What is the moment of inertia of the rotating ball before the rope is wrapped around the pole measured in kgm² (answer with 3 decimal places)?

A thin stick of mass 0.2 kg and length L = 0.5 m is attached to the rim of a metal disk of mass M = 2.0 kg and radius R = 0.3 m . The stick is free to rotate around a horizontal axis through its other end (see the following figure). (a) If the combination is released with the stick horizontal, what is the speed of the center of the disk when the stick is vertical? (b) What is the acceleration of the center of the disk at the instant the stick is released? (c) At the instant the stick passes through the vertical?

What is the moment of inertia a 30 cm long and 3.0 cm wide ruler, with a mass of 50 g, around the axis passing through the geometric center. For how much does the moment of inertia change if we drill a hole in the ruler with a diameter of 1.0 cm, 5.0 cm from the end of the ruler? (The solutions are 3750 g×cm^2 and -44 g×cm^2 )

The figure above shows two barbells, which can be modeled as two point masses connected by a massless rod. In the figure, L = 0.77 m and m = 20.1 kg. The barbells can rotate about an axis that is perpendicular to the rod and passes through the midpoint of each rod. For the barbell with the larger moment of inertia, give the moment of inertia (in units of kg m2).

Consider the setup shown below. The blocks have masses 3.6 kg and 24 kg. The pulley has mass 7.4 kg, and is a uniform disc with radius 0.23 m. Assume the pulley to be frictionless, but the coefficient of friction between the block and the surface is 0.36. What is the acceleration of the blocks? Assume the 24 kg mass is descending with acceleration a. The moment of inertia of the disk is 1/2MR2 and the acceleration of gravity is 9.8 m/s2.

An oversized yo-yo is made from two identical solid disks each of mass M = 2.10 kg and radius R = 10.0 cm. The two disks are joined by a solid cylinder of radius r = 4.00 cm and mass m = 1.00 kg as in the figure below. Take the center of the cylinder as the axis of the system. R. M (a) What is the moment of inertia of the system? Give a symbolic answer. (Use any variable or symbol stated above as necessary.) moment of inertia %3D