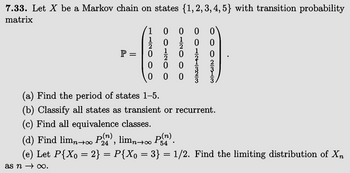
7.33. Let X be a Markov chain on states {1,2,3,4,5} with transition probabilitymatrixP =0 0023-3○ 0123230120 000 0 12O O1120 O O000(a) Find the period of states 1-5.0(b) Classify all states as transient or recurrent.(c) Find all equivalence classes.(d) Find lim∞ P(n), limn∞ P(n).54(e) Let P{X0 = 2} = P{X。 = 3} = 1/2. Find the limiting distribution of Xnas n → ∞.

Answered: Image /qna-images/answer/43e9cb49-be11-41ab-aa71-cdc75311b89c.jpg

Answered: 7.33. Let X be a Markov chain on states…

Advanced Engineering Mathematics

10th Edition

ISBN:9780470458365

Author:Erwin Kreyszig

Publisher:Erwin Kreyszig

Chapter2: Second-order Linear Odes

Section: Chapter Questions

Problem 1RQ

See similar textbooks

Similar questions

• General notation for Markov chains: P(A) is the probability of the event A when the Markov chain starts in state x, Pμ(A) the probability when the initial state is random with distribution μ. Ty = min{n ≥ 1: X₂ = y} is the first time after 0 that the chain visits state y. Px,y = Px(Ty < ∞) . Ny is the number of visits to state y after time 0. 4. Let Sn, n20 be a random walk on Z with step distribution 1-p 2 P 1) = 1/², P(X₁ = 1) = 2/₁ 2' 2' P(X₂ = 0) P(X₂ = − 1) = for some 0 < p < 1, p ‡ / . We may denote q=1 - p. That is, the increments (X₂)₁ are i.i.d. and S₂ = X₁ + … + Xn for n ≥ 1 and S = 0. Compute E[S2+1 Sn] for n ≥ 1. (b) Show that Mn = (q/p) Sn defines a martingale (with respect to (Xk)k-1). (c) Does the limit limn→∞ Mn exist almost surely? If yes, give a justification. If your answer is no, explain why. (d) Let T be the first time that S is equal to either −3 or 3. Compute P(ST = 3). Hint: You may use, without proof, the fact P(T<∞) = 1 and that the Optional Stopping Theorem…
The sequence (Xn)n>o is a Markov chain with transition matrix 0 을 0 0 0 3 1 0 0 0 4 4 0 } 0 0 0 1 2 1 1 0 0 3 3 0 } 0 } 0 0 0 0 0 0 0 (a) Draw the transition diagram for (Xn). (b) Determine the communicating classes of the chain, stating whether each class is recurrent or transient. (c) Determine the period of each communicating class.
Consider a continuous-time Markov chain whose jump chain is a random walk with reflecting barriers 0 and m where po,1 = 1 and pm,m-1 =1 and pii-1 = Pii+1 = for 1
18/ the transition frobabitity fis is Writtenas / if the markokchainisrefresentet by the fottoweing Brababitity matri A 0-85 o.15 0 fte f.0 theA the nuaberof equivalence rows is 2erz0r3ory Stachastic Process
1. For each of the transition matrices given below, list absorbing state(s), and determine the period of each of the states in each Markov chain. (a) P = (d) P = 1120 CLI2L 1434L3 0 ܚ . ܟ ܝ. | ܟ ܝܕ . ܚ 0 00 0 0 0 0 0 3I4LI4 LIABI+ 0 0 (b) P = 1 0 Lo (e) P = 0 1 1 0 0 1333 COLITOLIN 0 0 0 0 0 213 100 0 0 3 00 100 0 -0 WIN 1 0 0 0 0 0 0 00 1 0 0 4|012 TWO O 0 0 0 0 0 (c) P = (f) P = 0 1|2 1|3 OLT20IN +15 O N|TN|TO 120 HINO OLI3 O 0 LI5L4L 1113 2I5
2. Consider a Markov chain {Xn}n≥o having the following transition diagram: 1/2 1 2 5 1/4 3 4 1/2 1/4 6 7 1/2 For this chain, there are two recurrent classes R₁ = {6,7} and R₂ = {1,2,5}, and one transient class R3 = {3,4}. (a) Find the period of state 3. (b) Find f33 and f22. (c) Starting at state 3, find the probability that the chain is absorbed into R₁. (d) Starting at state 3, find the mean absorbation time, i.e., the expected number of steps that the chain is absorbed into R₁ or R₂. Note: there are missing transition probabilities for this chain, but no impact for your solution.
12. Let Z be an irreducible discrete-time Markov chain on a countably infinite state space S, having transition matrix H = (hij) satisfying hii = 0 for all states i, and with stationary distribution v. Construct a continuous-time process X on S for which Z is the imbedded chain, such that X has no stationary distribution.
It is not always possible to construct by ruler and compass, a square equal in area to the area of a circle.
2. For all permissible p values, determine the equivalence classes of the Markov chain with the following transition matrix P, classify states as transient or recurrent, and classify the Markov chain as irreducible or reducible. 0. 1-p 1 - 0. P = 0. 1-p 0. 1-p
3. Let Xi ∼ Poi(1), i = 1, . . . , n, be n = 20 independent Poisson r.v’s.Hint: you may use E(Y ) = λ and Var(Y ) = λ, for a Poisson r.v. Y ∼ Poi(λ). 3a. Use the Markov inequality to obtain a bound on Pr (Ln Xi > 30) (±0.05 is okay). 3b. Use the CLT to approximate the same probability (±0.01 is okay)
1. Prove that Weiner Process is Markovian (Markov Process).
Consider a Markov chain with two possible states, S = {0, 1}. In particular, suppose that the transition matrix is given by Show that pn = 1 P = [¹3 В -x [/³² x] + B x +B[B x] x 1- B] (1-x-B)¹ x +B x -|- -B ·X₁ В

SEE MORE QUESTIONS

Recommended textbooks for you

Advanced Engineering Mathematics

Advanced Math

ISBN:

9780470458365

Author:

Erwin Kreyszig

Publisher:

Wiley, John & Sons, Incorporated

Numerical Methods for Engineers

Advanced Math

ISBN:

9780073397924

Author:

Steven C. Chapra Dr., Raymond P. Canale

Publisher:

McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…

Advanced Math

ISBN:

9781118141809

Author:

Nathan Klingbeil

Publisher:

WILEY

Advanced Engineering Mathematics

Advanced Math

ISBN:

9780470458365

Author:

Erwin Kreyszig

Publisher:

Wiley, John & Sons, Incorporated

Numerical Methods for Engineers

Advanced Math

ISBN:

9780073397924

Author:

Steven C. Chapra Dr., Raymond P. Canale

Publisher:

McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…

Advanced Math

ISBN:

9781118141809

Author:

Nathan Klingbeil

Publisher:

WILEY

Mathematics For Machine Technology

Advanced Math

ISBN:

9781337798310

Author:

Peterson, John.

Publisher:

Cengage Learning,

Basic Technical Mathematics

Advanced Math

ISBN:

9780134437705

Author:

Washington

Publisher:

PEARSON

Topology

Advanced Math

ISBN:

9780134689517

Author:

Munkres, James R.

Publisher:

Pearson,

SEE MORE TEXTBOOKS