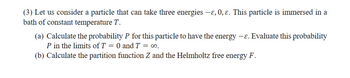
(3) Let us consider a particle that can take three energies —ɛ, 0, ɛ. This particle is immersed in a bath of constant temperature T. (a) Calculate the probability P for this particle to have the energy -ε. Evaluate this probability P in the limits of T = 0 and T = ∞o. (b) Calculate the partition function Z and the Helmholtz free energy F.

The energy spectrum of a particle consists of four states with energies 0,e, 2 e,3 e. Let Z, (T),Z, (T) and Ze(") denote the canonical partition functions for four non- interacting particles at temperature T. The subscripts B, F and C corresponds to bosons, fermions and distinguishable classical particles, respectively. Let y= exp k„T Which one of the following statements is true about ZB (T), ZF (T) and Zc (T)? (a) They are polynomials in y of degree 12,6 and 12, respectively. (b) They are polynomials in y of degree 16,10 and16, respectively (c) They are polynomials in y of degree 9,6 and 12, respectively. (d) They are polynomials in y of degree 12,10 and 16, respectively.

Obtain the expression for the average thermal energy when the partition function is Z: ħo (A) 극청고 2 (B) kzT 1 (C) -k,T 4 (D) 4k,T

The probability p; of occupying an available state j is: Pj 9je-BEj Z where g; is the degeneracy of the state and 1/ẞ=kBT. Derive an expression for the temperature I in terms of the probabilities of occupation of two different states i and k.

Given the internal energy U and entropy S of N weakly interacting particles in a closed system with fixed volume V. U = NkgT² (27In 2) U S = Nkg lnz + T (a) Prove the Helmholtz free energy (b) Prove the Pressure of the system is F = -NkBT ln z P = Nk Tln z) ( T

Consider N non interacting particles in a gas are in thermal equilibrium and each particle can be in any one of the possible non-degenerate states of energy 0 and 28. Find the probability of each particle when temperature of the gas (T) is very much E larger than the value of (a) (b) 1 alm (d) م اما م ادا

Show that the partition function for a simple harmonic oscillator is e-Bwħ/2 1-e-Bhw (a) Calculate the internal energy, U and show that the high-temperature result is consistent with the equipar- tition theorem result. (b) Calculate the heat capacity, Cy and show that the high-temperature result is consistent with the equipar- tition theorem result. (c) Calculate the Helmholtz function, F

In quantum statistical mechanics, consider a system of independent, distinguishable particles, each of which has only two accessible states; a ground state of energy 0 and an excited state of energy ε. If the system is in equilibrium with a heat bath of temperature T, calculate A (Helmholtz free energy), E (internal energy), S (entropy), and Cv (specific heat capacity at constant volume). Does the choice of the ground-state energy equal 0 affect P (pressure), Cv, or S? How would your results change if ε0 were added to both energy values?

one-dimensional A one-particle, system has the potential energy function V = V₁ for 0 ≤ x ≤ 1 and V = ∞ elsewhere (where Vo is a constant). a) Use the variation function = sin() for 0 ≤ x ≤ 1 and = 0 elsewhere to estimate the ground-state energy of this system. b) Calculate the % relative error.

2.6 (a), (b) • (a) Show that, if the kinetic energy of a particle with mass m, momentum p p²/2m, the single particle partition function can be written Z1 = V/23, where 1 = partition function for the ideal gas will then be is E = Vh2/2nmkgT is the thermal wavelength. The canonical VN ZN N! 23N • (b) Use Stirling's approximation to show that in the thermodynamic limit the Helmholtz free energy of an ideal gas is A = -NkgT In ( V +1 N23