28 b ab a² a 2 Euclid's approach to geometry 62 ab b Figure 2.8: The square of a sum of line segments • The square on the line is what we write as (a + b)². • The squares on the two segments a and b are a² and b², respectively. The rectangle "contained" by the segments a and b is ab. • The square (a+b)² equals (in area) the sum of a², b², and two copies of ab. It should be emphasized that, in Greek mathematics, the only inter- pretation of ab, the "product" of line segments a and b, is the rectangle with perpendicular sides a and b (or "contained in" a and b, as Euclid used to say). This rectangle could be shown "equal" to certain other regions, but only by cutting the regions into identical pieces by straight lines. The Greeks did not realize that this "equality of regions" was the same as equal- ty of numbers-the numbers we call the areas of the regions-partly be- ause they did not regard irrational lengths as numbers, and n ey did not think the product of lengtho al 2.4 Area of parallelograms and triangles Exercises In Figure 2.8, the large square is subdivided by two lines: one of them perpendic- ular to the bottom side of the square and the other perpendicular to the left side of the square. 29 2.3.1 Use the parallel axiom to explain why all other angles in the figure are necessarily right angles. Figure 2.8 presents the algebraic identity (a + b)2 = a² + 2ab + b² in geo- metric form. Other well-known algebraic identities can also be given a geometric presentation. 2.3.2 Give a diagram for the identity a(b+c) = ab + ac. 2.3.3 Give a diagram for the identity a²-b² = (a+b)(a - b). Euclid does not give a geometric theorem that explains the identity (a+b)³ = a³ +3a²b+3ab² + b3. But it is not hard to do so by interpreting (a+b)3 as a cube with edge length a + b, a³ as a cube with edge a, a²b as a box with perpendicular edges a, a, and b, and so on. 2.3.4 Draw a picture of a cube with edges a + b, and show it cut by planes (parallel to its faces) that divide each edge into a segment of length a and a segment of length b. 2.3.5 Explain why these planes cut the original cube into eight pieces: a cube with edges a, • a cube with edges b, • three boxes with edges a, a,b, • three boxes with edges a, b,b. 2.4 Area of parallelograms and triangles un "equal" to a rectar
28 b ab a² a 2 Euclid's approach to geometry 62 ab b Figure 2.8: The square of a sum of line segments • The square on the line is what we write as (a + b)². • The squares on the two segments a and b are a² and b², respectively. The rectangle "contained" by the segments a and b is ab. • The square (a+b)² equals (in area) the sum of a², b², and two copies of ab. It should be emphasized that, in Greek mathematics, the only inter- pretation of ab, the "product" of line segments a and b, is the rectangle with perpendicular sides a and b (or "contained in" a and b, as Euclid used to say). This rectangle could be shown "equal" to certain other regions, but only by cutting the regions into identical pieces by straight lines. The Greeks did not realize that this "equality of regions" was the same as equal- ty of numbers-the numbers we call the areas of the regions-partly be- ause they did not regard irrational lengths as numbers, and n ey did not think the product of lengtho al 2.4 Area of parallelograms and triangles Exercises In Figure 2.8, the large square is subdivided by two lines: one of them perpendic- ular to the bottom side of the square and the other perpendicular to the left side of the square. 29 2.3.1 Use the parallel axiom to explain why all other angles in the figure are necessarily right angles. Figure 2.8 presents the algebraic identity (a + b)2 = a² + 2ab + b² in geo- metric form. Other well-known algebraic identities can also be given a geometric presentation. 2.3.2 Give a diagram for the identity a(b+c) = ab + ac. 2.3.3 Give a diagram for the identity a²-b² = (a+b)(a - b). Euclid does not give a geometric theorem that explains the identity (a+b)³ = a³ +3a²b+3ab² + b3. But it is not hard to do so by interpreting (a+b)3 as a cube with edge length a + b, a³ as a cube with edge a, a²b as a box with perpendicular edges a, a, and b, and so on. 2.3.4 Draw a picture of a cube with edges a + b, and show it cut by planes (parallel to its faces) that divide each edge into a segment of length a and a segment of length b. 2.3.5 Explain why these planes cut the original cube into eight pieces: a cube with edges a, • a cube with edges b, • three boxes with edges a, a,b, • three boxes with edges a, b,b. 2.4 Area of parallelograms and triangles un "equal" to a rectar
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Question
Exercise 2.3.3

Transcribed Image Text:28
b
a
ab
a²
2 Euclid's approach to geometry
62
ab
b-
Figure 2.8: The square of a sum of line segments
• The square on the line is what we write as (a + b)².
• The squares on the two segments a and b are a² and b², respectively.
• The rectangle "contained" by the segments a and b is ab.
• The square (a + b)² equals (in area) the sum of a², b², and two copies
of ab.
It should be emphasized that, in Greek mathematics, the only inter-
pretation of ab, the "product" of line segments a and b, is the rectangle
with perpendicular sides a and b (or "contained in" a and b, as Euclid used
to say). This rectangle could be shown "equal" to certain other regions,
but only by cutting the regions into identical pieces by straight lines. The
Greeks did not realize that this "equality of regions" was the same as e
ty of numbers-the numbers we call the areas of the regions-partly be-
ause they did not regard irrational lengths as numbers, and no
ey did not think the product of length al
2.4
Area of parallelograms and triangles
29
Exercises
In Figure 2.8, the large square is subdivided by two lines: one of them perpendic-
ular to the bottom side of the square and the other perpendicular to the left side of
the square.
2.3.1 Use the parallel axiom to explain why all other angles in the figure are
necessarily right angles.
Figure 2.8 presents the algebraic identity (a + b)² = a² + 2ab + b² in geo-
metric form. Other well-known algebraic identities can also be given a geometric
presentation.
2.3.2 Give a diagram for the identity a(b+c) = ab + ac.
2.3.3 Give a diagram for the identity a² − b² = (a+b)(a −b).
Euclid does not give a geometric theorem that explains the identity (a + b)³ =
a³ +3a²b+3ab² + b³. But it is not hard to do so by interpreting (a+b)³ as a cube
with edge length a+b, a³ as a cube with edge a, a²b as a box with perpendicular
edges a, a, and b, and so on.
2.3.4 Draw a picture of a cube with edges a+b, and show it cut by planes (parallel
to its faces) that divide each edge into a segment of length a and a segment
of length b.
2.3.5 Explain why these planes cut the original cube into eight pieces:
• a cube with edges a,
• a cube with edges b,
• three boxes with edges a, a, b,
• three boxes with edges a, b, b.
2.4 Area of parallelograms and triangles
"equal" to a rectar
Expert Solution
Step 1: Square of side A
We have to give the diagram for identity
Since we use a 10*10 square.Let the side of square is A, then the area is .
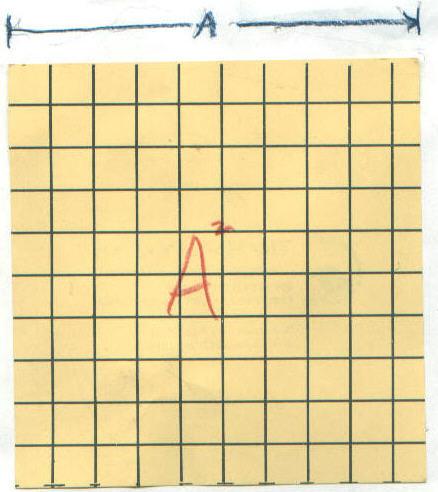
Step by step
Solved in 5 steps with 13 images

Recommended textbooks for you

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,

