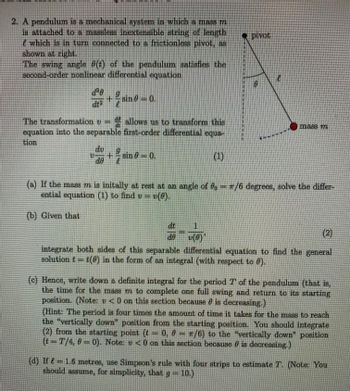
2. A pendulum is a mechanical system in which a mass m is attached to a massless inextensible string of length which is in turn connected to a frictionless pivot, as shown at right. The swing angle (t) of the pendulum satisfies the second-order nonlinear differential equation de + sin 0-0. The transformation v = allows us to transform this equation into the separable first-order differential equa- tion (1) 1- sin 0-0. pivot mass m

identify the differential equations defining the motion of the masses from the figure, via Newton’s 2nd law,      hence construct the system matrix, A, where the state vector is:x=[x;x ̇;y;y ̇ ]

The relative displacement u(t) of a single-storey shear building subjected to an earthquake ground motion is represented by the following second-order linear ordinary differential equation: d?u dt2 du + c + ku = a, (t) m dt where m, c, and k are the mass (kg), damping constant (Ns/m), and stiffness of the structure (N/m), respectively. Meanwhile, a,(t) is the function of earthquake ground acceleration. Suppose the building with a mass of 2000 kg and supported by columns of combined stiffness of 32 x 103 N/m is subjected to earthquake with ground acceleration given by the following function: ag(t) = 36000 cos 2t Find the equation of the displacement, u(t), given the damping is not installed to the building. Then, find how much the building is displaced from t =30 seconds to t =60 seconds of earthquake.

An object attached to a spring undergoes simple harmonic motion modeled by the differential equation d²x = 0 where x (t) is the displacement of the mass (relative to equilibrium) at time t, m is the mass of the object, and k is the spring constant. A mass of 3 kilograms stretches the spring 0.2 meters. dt² Use this information to find the spring constant. (Use g = 9.8 meters/second²) m k = + kx The previous mass is detached from the spring and a mass of 17 kilograms is attached. This mass is displaced 0.45 meters below equilibrium and then launched with an initial velocity of 2 meters/second. Write the equation of motion in the form x(t) = c₁ cos(wt) + c₂ sin(wt). Do not leave unknown constants in your equation. x(t) = Rewrite the equation of motion in the form ä(t) = A sin(wt + ), where 0 ≤ ¢ < 2π. Do not leave unknown constants in your equation. x(t) =

Differential equations: mechanical vibrations Show one example. Summarize the method. How would you solve these applications without using a linear system?

My question and answer is in the image. Can you please check my work? A 2 kg mass is attached to a spring with spring constant 50 N/m. The mass is driven by an external force equal tof(t) = 2 sin(5t). The mass is initially released from rest from a point 1 m below the equilibrium position. (Use theconvention that displacements measured below the equilibrium position are positive.)(a) Write the initial-value problem which describes the position of the mass. 2y"+50y=2cos(5t) (b) Find the solution to your initial-value problem from part (a). (1+(1/2)tcos(t))cos(5t)-(1/2)tcos(t) (c) Circle the letter of the graph below that could correspond to the solution. B (d) What is the name for the phenomena this system displays? Resonance

A weighing scale can be modelled as a dynamic system, as shown in Figure. The transfer function of the system, using Laplace transforms, is: F(t) m Oms² + cs+k=F(s) mx+cx+kx = F(t) X (s) F(s) 1 mx + cx + k X (s) F(s) ms 2 1 +cs + k 2

For the following water liquid level system, 9mi(t) is the mass flow rate input deviation to the system and the restrictions can be considered linear resistances about the equilibrium: 9mi h2 Ro R1 a) Using basic fluids principles, develop the Dynamic Equations for the deviation of the two liquid level heights from their equilibrium height as a function of the input mass flow rate, Imi-

Problem Derive the differential equation governing the motion of the one degree-of-freedom system by applying the appropriate form(s) of Newton's laws to the appropriate free-body diagrams. Use the generalized coordinate shown in Figure P2.427. Linearize nonlinear differential equations by assuming small displacements. 2k - Rigid massless link Identical slender bars of mass m, length L. FIGURE P 2.47

(k - 1)/k P2 Tds relationship: Tds = dh -vdp. Start from the Tds function, please derive that . Please T1 P1 list clearly the assumptions/conditions applied for the derivation