
Elements Of Electromagnetics
7th Edition
ISBN: 9780190698614
Author: Sadiku, Matthew N. O.
Publisher: Oxford University Press
expand_more
expand_more
format_list_bulleted
Topic Video
Question
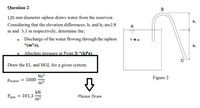
Transcribed Image Text:Question 2.
B
120 mm diameter siphon draws water from the reservoir.
hị
Considering that the elevation differences, hị and h2 are2.8
m and 5.2 m respectively, determine the;
A
a. Discharge of the water flowing through the siphon
*(m³/s),
h2
b. Absolute pressure at Point B *(kPa).
Draw the EL and HGL for a given system.
Ns?
Figure 2
Pwater = 1000
m4
kN
Patm = 101,3
m2
Please Draw
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 2 steps with 1 images

Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- = Water flows into a tank and out through another pipe, as shown in the figure below. The water in the tank has a surface area, Asur f 5.6 m². At the bottom of the tank there is a door inclined at an angle = 25 degrees with respect to the horizontal. The door has a length L = 1.1 m and a width w=1 m (out of the page). The flowrate into the tank is Q₁ (t) flowrate out is Qo(t) = 0.05 m³/s At time t = Asurf = 0.26 m³/s and the 0, the water has a depth ho = 2.5 m. The density of water is p = 1000 kg/m³. Ө h(t) Qoutarrow_forwardWater flows over a dam into a rectangular channel of width b (out of page). At the bottom of the dam, the depth of flow is h₁. As you may have seen on watching rapid channel flow, the water may suddenly change elevation to height h₂ as it passes through a highly disturbed region called the hydraulic jump. If velocity is assumed uniform at 1 and 2, compute the height h2 using the control volume analysis. Take the pressures at 1 and 2 as hydrostatic, and assume that the flow is steady. Neglect friction at the channel bed and walls. (Courtesy of the Wright Water Engineers, Inc. and ASDSO) Hydraulic jump h₁ = 3 ft V₁ = 25 ft/s BE 2 h₂ =? V₂ = ? (a) Find the cubic equation, in terms of h₁, V₁, and g, that you must solve for the downstream depth h₂ of the water channel. (b) For h₁ = 3 ft and V₁ = 25 ft/s, find the downstream depth h₂. Use the standard value for g. Note that you are solving a cubic equation, and only one of the solutions is correct.arrow_forwardPlease helparrow_forward
- Determine the available suction head of the pump that is taking a gasoline at 204°C at a closed tank with a pressure 585 kPa gauge. the specific gravity of gasoline is 0.78 and it’s vapor pressure is 620 kPa absolute. the loss in suction pipe is 0.60 m and the pump center line is located 3.60 m above the gasoline level A. 8.66m B. 3.67m C. 7.66m D. 4.47marrow_forward8. In the figure below a manometer uses oil with specific gravity of 0.8. (a) Calculate the discharge when R = 1 ft. (b) Repeat the problem if the pipe is inclined at 30° upward. oil R 6" 3" -- 6"arrow_forwardWater flows at the rate of 0.015 m/sec from open reservoir A to open reservoir B (both are large reservoirs that are open to the atmosphere) through two concrete (8 = 0.32 mm) pipes connected in series. If L1 = 800 m, Di = 16 cm, L2 = 200 m, and D2 = 8 cm, determine the difference in water surface elevations (Az) of the reservoirs. The coefficient of contraction (K.) is 0.36, the entrance coefficient is K = 0.5, the exit coefficient is K = 1.0, and assume fully turbulent flow (Xx = 9810 N/m?, Ku = 1.12 x 10-6 m²/s). You do not need to check if the assumption of fully turbulent flow is valid, just assume that it is valid. А Az =? Pipe 1 В Pipe 2 undefinedarrow_forward
- A pipe, 12 inch in diameter at A, discharges 4.0 cfs of heavy fuel oil (sp gr = 0.899) into the air at B, where the diameter is 6 inch. If B is 12 ft above A and the frictional loss between the two points is equivalent to 3.0 lb per sq in, determine the pressure at A in pounds per square inch.arrow_forwardDetermine the flow in L/s into or out of each reservoir in the pipe system shown; Use n = 0.011 for all pipes L=1200 m; L2= 900 m; La= 1500 m; Di= 30 cm; D; = 20 cm; D3 = 15 cm %3D %3D El 30 m El 24 m 1 El 15 m El 0 m B. 3.arrow_forwardO1: A stream of water of diameter d = 0.1 m flows steadily from a tank of diameter D= 1.0 m. Determine The Weight Flowrate, needed from the inflow pipe if the water depth remains constant, 0 — d=010marrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press
Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON
Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education
Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY
Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY
Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY

Elements Of Electromagnetics
Mechanical Engineering
ISBN:9780190698614
Author:Sadiku, Matthew N. O.
Publisher:Oxford University Press

Mechanics of Materials (10th Edition)
Mechanical Engineering
ISBN:9780134319650
Author:Russell C. Hibbeler
Publisher:PEARSON

Thermodynamics: An Engineering Approach
Mechanical Engineering
ISBN:9781259822674
Author:Yunus A. Cengel Dr., Michael A. Boles
Publisher:McGraw-Hill Education

Control Systems Engineering
Mechanical Engineering
ISBN:9781118170519
Author:Norman S. Nise
Publisher:WILEY

Mechanics of Materials (MindTap Course List)
Mechanical Engineering
ISBN:9781337093347
Author:Barry J. Goodno, James M. Gere
Publisher:Cengage Learning

Engineering Mechanics: Statics
Mechanical Engineering
ISBN:9781118807330
Author:James L. Meriam, L. G. Kraige, J. N. Bolton
Publisher:WILEY