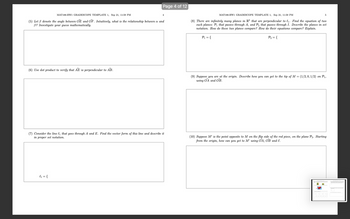
MAT188-HW1 GRADESCOPE TEMPLATE 1, Sep 21, 11:59 PM Page 4 of 12 (5) Let ẞ denote the angle between OE and OF. Intuitively, what is the relationship between a and B? Investigate your guess mathematically. (6) Use dot product to verify that AE is perpendicular to AB. MAT188-HW1 GRADESCOPE TEMPLATE 1, Sep 21, 11:59 PM 5 (8) There are infinitely many planes in R³ that are perpendicular to l₁. Find the equation of two such planes: P₁ that passes through A, and P2 that passes through I. Describe the planes in set notation. How do these two planes compare? How do their equations compare? Explain. P₁ = { P2 = { (9) Suppose you are at the origin. Describe how you can get to the tip of M = (1/2, 0, 1/2) on P1, using OA and OB. (7) Consider the line l₁ that goes through A and E. Find the vector form of this line and describe it in proper set notation. (10) Suppose M' is the point opposite to M on the flip side of the red piece, on the plane P2. Starting from the origin, how can you get to M' using OA, OB and v. 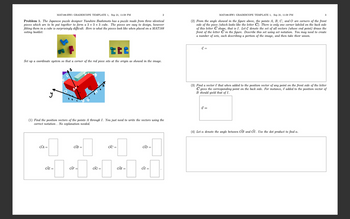l₁ = { MAT188-HW1 GRADESCOPE TEMPLATE 1, Sep 21, 11:59 PM 2 Problem 1. The Japanese puzzle designer Yasuhiro Hashimoto has a puzzle made from three identical pieces which are to be put together to form a 3 × 3 × 3 cube. The pieces are easy to design, however fitting them in a cube is surprisingly difficult. Here is what the pieces look like when placed on a MAT188 voting booklet: 3 MAT188-HW1 GRADESCOPE TEMPLATE 1, Sep 21, 11:59 PM (2) From the angle showed in the figure above, the points A, B, C, and O are corners of the front side of the piece (which looks like the letter C). There is only one corner labeled on the back side of this letter C shape, that is I. Let C denote the set of all vectors (whose end point) draws the front of the letter C in the figure. Describe this set using set notation. You may need to create a number of sets, each describing a portion of the image, and then take their union. tte Set up a coordinate system so that a corner of the red piece sits at the origin as showed in the image. C = (3) Find a vector that when added to the position vector of any point on the front side of the letter C gives the corresponding point on the back side. For instance, v added to the position vector of B should yield that of I. y (1) Find the position vectors of the points A through I. You just need to write the vectors using the correct notation. No explanation needed. √ = (4) Let a denote the angle between OB and OI. Use the dot product to find a. OA = OB = OC = OD= OE= OF OG = OH = σI =

An ant is sitting on a spinning CD 26 centimeters from the center. The ant rides the CD for 14 seconds and travels 820 centimeters. What angle does the ant turn through in radians? rad What is the linear speed of the ant in cm/sec? cm/sec What is the angular velocity of the ant in radians per second? rad/sec What is the ant's rotational speed in revolutions per minute? грт Question Help: Video D Post to forum Submit Question esc @ 3 4 e r t y tab W 个

I need help

SHOW ALL WORK!!! 2) (3, 50"), Convert to rectangular coordinates and state which quadrant it is in. Page acer -> Cc 23 24 & * ( 4. 6 7 8 9. e y

Your answer 10) If a point M, located at (3, -2), is rotated clockwise 90 about the origin, what are the coordinates of its image?" Your answer Submit Never submit passwords through Google Foma Ths iorm was created inside of San Juan Linified Bchool District Bepat Alng Google Forms INTL O1

Workbook 12.2| 30-60-90 Triang x Desmos | Graphing Calculator 5.strongmind.com/courses/13572/assignments/2131826?module_item_id%3D5792940 Which image shows the correct ratios for a 30-60-90 triangle that can be used as a formula to figure out the missing side lengths? B 60 30° © 2020 StrongMind. Created using GeoGebra. 60° rV3 30

a boat travels towards the northeast at 23 knots and there is an 8 knot crosswind out of the southeast affecting the boat convert the boats motion (not the crosswind) into rectangular coordinates

Did I do it correctly?

lverado High School C Clever | Portal O Quiz: Rotations QUIZ /courses/124251/quizzes/1064975/take Question 7 Which mapping represents a rotation of 270° clockwise about the origin?

find the polar coordinates  (-8, 2?/3)