What is meant by chords in a circle?
A chord of a circle is a line segment with its ends placed on the circle. A chord that passes through the center of the circle is the diameter of the circle. It must be noted that it is the longest chord of a circle. The word chord originated from the Latin word ‘chorda’ which means bowstring. Several line segments exist but it is the chord that is the only line segment whose endpoints lie on the periphery of the circle.

Properties of Chords
- All the chords are at the same lengths (equidistant) from the center of a circle provided all of them are equal in length.
- The perpendicular bisector of a chord always passes through the center of a circle.
- A perpendicular line passing from the center of a circle bisects the chord.
- The line segment bisecting a chord through its center is perpendicular to the chord.
- If a central angle and an inscribed angle are subtended by the same chord as well as on the same part of the chord of a circle, then the central angle is twice the value of the inscribed angle.
- If a pair of angles are inscribed on the same chord as well as on the same side of the chord, then they are said to be equal.
- If two angles are inscribed on the same chord and opposite sides of the chord, then they are supplementary.
- In the case of a cyclic quadrilateral, the exterior angle is equal to the interior opposite angle.
- According to Thales’ theorem, an inscribed angle extended by a diameter is a right angle
- The longest chord of the circle is known to be the diameter which is twice the length of the radius of the circle.
- If the crossing of any two chords separates one chord into lengths ‘a’ and ‘b’ and the other chord into lengths ‘c’ and ‘d’, then ‘ab’ is equal to ‘cd’.
- If the convergence of any two perpendicular chords divides one chord into lengths ‘a’ and ‘b’ and the other chord into lengths ‘c’ and ‘d’, then the summation of a2, b2, c2, and d2 equals the square of the circle’s diameter.
- The addition of the squared lengths of any two chords crossing at right angles at a particular point is the same as that of any other two perpendicular chords intersecting at the same point. The equation is 8r2 − 4p2, where ‘r’ is the circle radius and ‘p’ is the distance from the central point to the point of intersection of the circle.
- The length from a given point on the circle to a given chord multiplied by the diameter of the circle equals the product of the distances from the given point to the endpoints of the chord in the circle.
- There exists only a single circle that passes through all the three collinear points
- When a chord is made, it segregates the circle into two sections also known as segments of the circle, one is the major segment, and another one is the minor segment.
- For two unequal chords of a circle, the larger chord will always be nearer to the center than the smaller chord. (Unequal Chords Theorem)
- In the same or congruent circles, the minor arcs are congruent provided their corresponding chords are congruent as well.
Theorems in chords
Theorem 1: Equal chords subtend equal angles at the center of a circle.
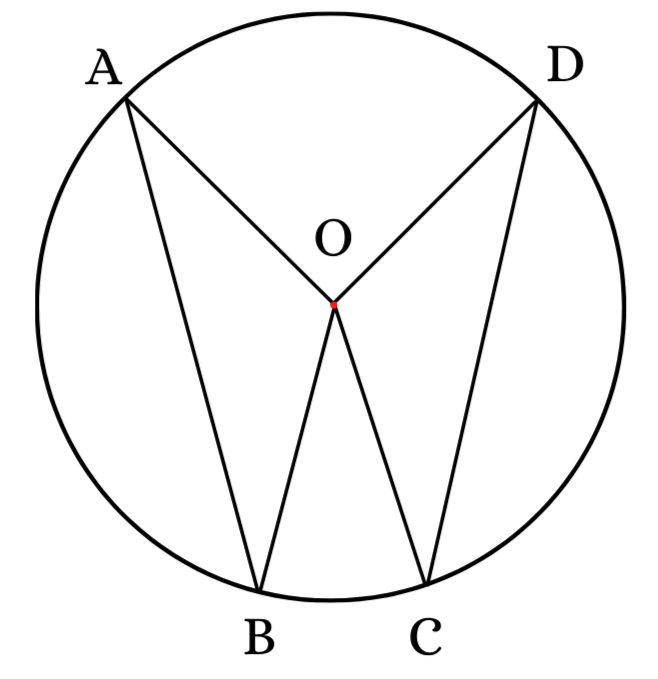
Given: A circle having center ‘O’ and AB and CD are the equal chords of the circle; so, AB = CD.
Prove that: ∠ AOB = ∠ DOC
Solution:
In △ AOB and △ DOC,
AO = OD (radii of the congruent circles)
AB = CD (given)
OB = OC (radii of the congruent circles)
Thus,
△ AOB △ COD (all the three corresponding sides have the same properties.)
Hence, ∠ AOB = ∠ DOC. (Corresponding Parts of Congruent Triangles)
Theorem 2: A perpendicular drawn from the center of the circle bisects the chord and it implies that both the halves of the chords are equal in length.
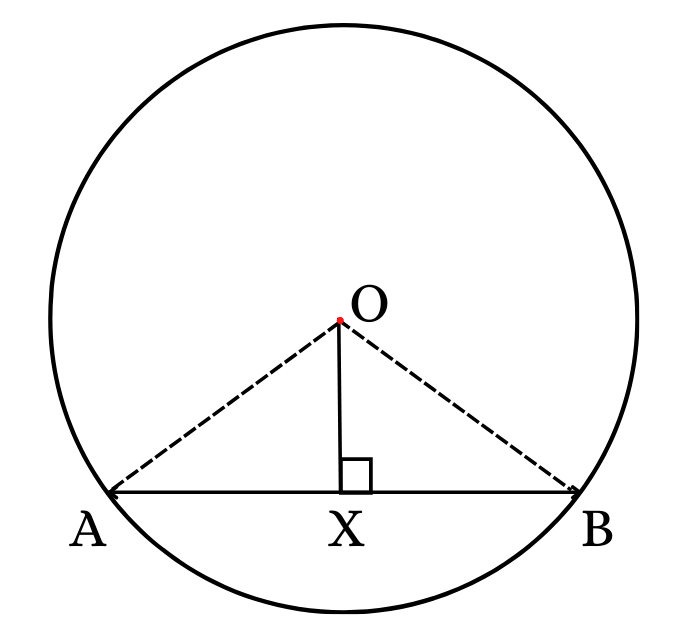
Given: A circle having center ‘O’ and AB is the chord, i.e., OX is perpendicular to AB
Prove: That OX bisects the AB chord or AX = BX
Solution:
In △ OAX and △ OBX,
∠ OXA = ∠ OXB (given that both are at right angles)
OA = OB (both radii)
OX = OX (common line)
△ OAX △ OBX
Theorem 3: The line that is drawn through the center of the circle to the midpoint of the chords is perpendicular to it. In another way, any line from the center that bisects a chord is perpendicular to the chord.
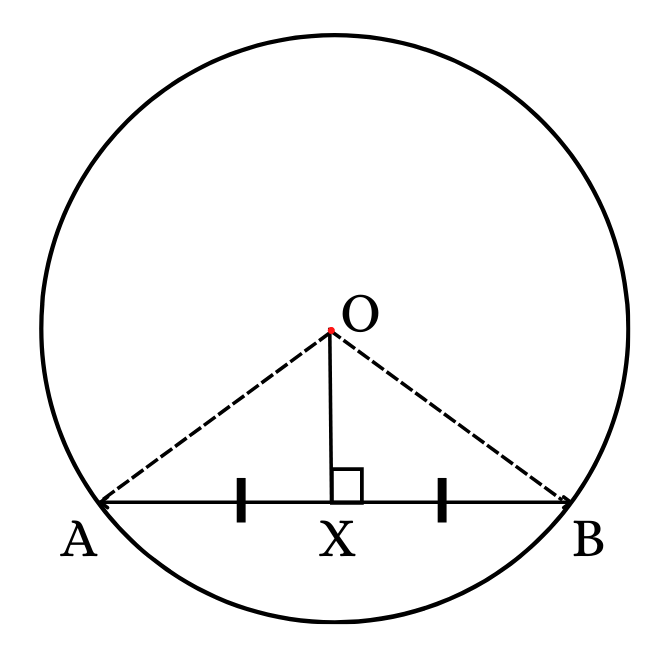
Given: A circle having center ‘O’, AB is the chord and OX bisects AB or AX = BX
Prove: That OX is perpendicular to AB
Solution:
In △ OAX and △ BOX,
OA = OB (Both radii)
OX = OX (common line)
AX = BX (given)
△ OAX △ BOX (all the three corresponding sides have same properties.)
∠ AXO = ∠ BXO (Corresponding Parts of Congruent Triangles) ……………. (1)
In line AB,
∠ AXO and ∠ BXO both form a linear pair,
∠ AXO + ∠ BXO = 180
∠ AXO = ∠ AXO = 180 (from (1))
2 ∠ AXO = 180
∠ AXO =
Thus, ∠ AXO = 90
Therefore, ∠ AXO = ∠ BXO = 90 and hence, OX is perpendicular to AB.
Theorem 4: If there are three non-collinear points, then there is only one circle that can pass through all of them.
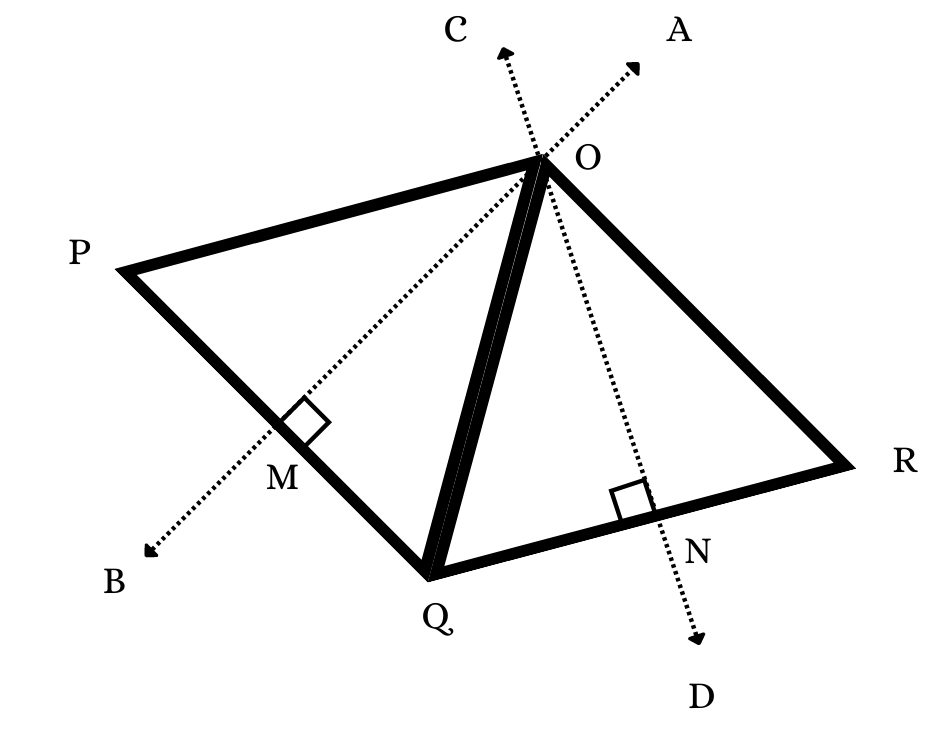
Given: PQR are three collinear points
Prove: That only a single circle will pass through the points PQR
Construction: make AB the perpendicular bisector of PQ at 'M' and CD the perpendicular bisector of QR at 'N'.
Solution:
PQ is not parallel to QR as given they are non-collinear
Thus, AB and CD are also not parallel and hence, they need to intersect at a certain point, assume here as 'O'
Connect OP, OQ and OR
In △ OPM and △ OQM,
OM = OM (common line)
∠ OMP = ∠ OMQ (both are right angles and AB is perpendicular to PQ)
PM = QM (since AB bisects PQ)
Therefore, △ OPM △ OQM (side-angle-side rule)
Thus, OP = OQ (Corresponding Parts of Congruent Triangles) …………. (1)
and similarly, OQ = OR …………. (2)
from (1) and (2), we get,
OP = OQ = OR
Now, let OP = OQ = OR = r
Take ‘O’ as a center and ‘r’ as a radius, and draw a circle. We observe that the circle passes through points P, Q, and R. Thus, they are concyclic.
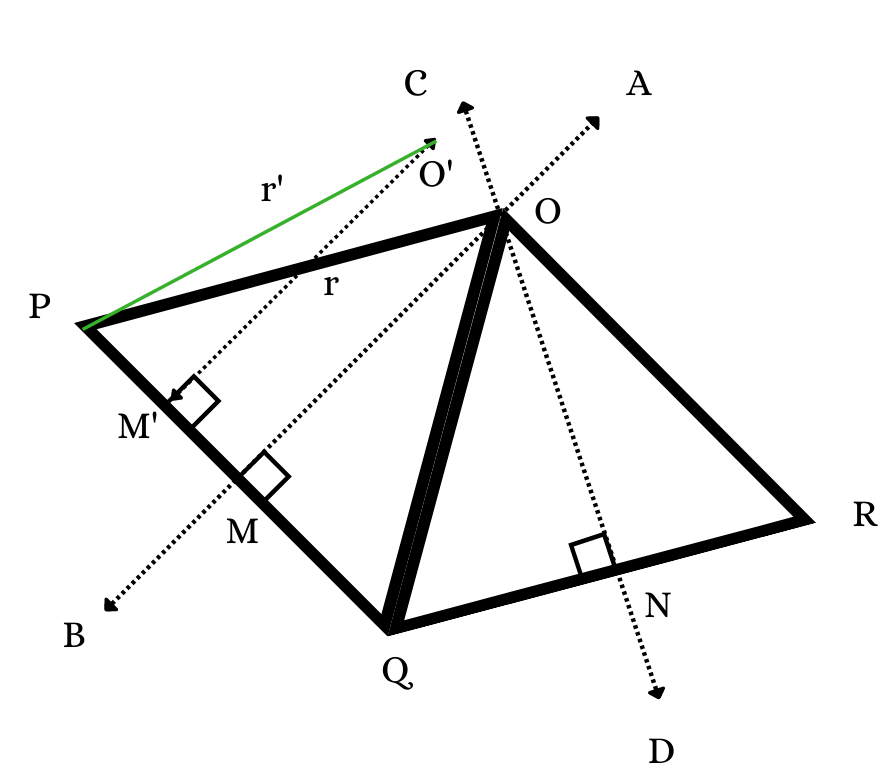
Consider another circle with center ‘O!’ and radius ‘r1’ passing through P, Q, and R. Drop a perpendicular such that OM’ is perpendicular to PQ.
So, PM’ = QM’ (perpendicular dropped from center bisects it), and thus M’ is the mid-point of PQ
OM’ is perpendicular bisector of PQ but OM is the perpendicular bisector of PQ. Since a line is capable of having only one perpendicular bisector, O1M’ coincides with OM and O1 coincides with O.
Thus, only one such circle can be present which passes through the points P, Q, and R.
Theorem 5: Equal chords of a circle are at the same distance from the center of a circle. The converse is also true.
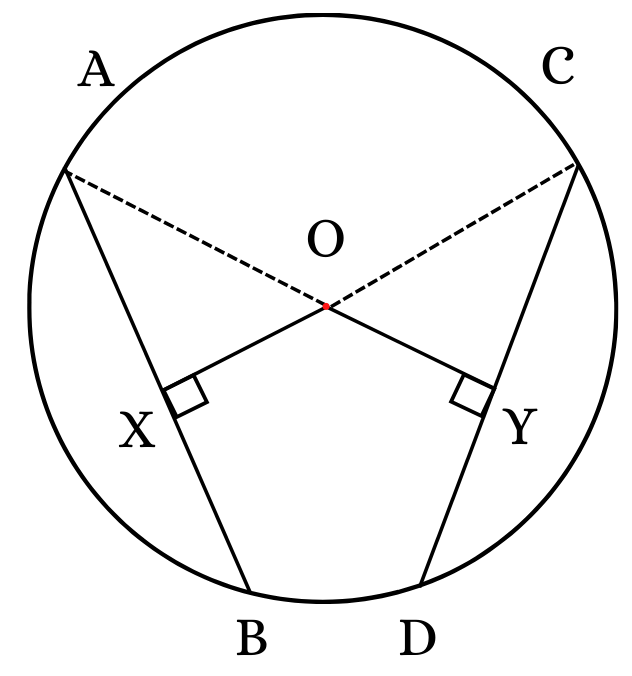
Given: A circle having center ‘O’, AB and CD are the two chords of a circle equal in length (AB = CD) and OX and OY are perpendicular to AB and CD respectively.
Prove: That OX = OY
Solution:
OX is perpendicular to AB (as perpendicular from the center towards the chord bisects it)
So, AX = BX = …………… (1)
Similarly, OY is perpendicular to CD (as perpendicular from the center towards the chord bisects it)
So, CY = DY = …………… (2)
Now, AB = CD (given)
Then,
AX = CY (from (1) and (2)) …………… (3)
In △ AOX and △ COY,
∠ OXA = ∠ OYC (Given that both are right angles)
OA = OC (Radius)
AX = CY (from (3))
△ AOX △ COY (By Right Hand Side Rule)
Hence, OX = OY (Corresponding Parts of Congruent Triangles)
Theorem 6: The angle subtended by an arc at the center of a circle is two times the angle that the arc subtends at any other given point on the circumference of the circle.
Given: A circle having center ‘O’, the arc of the circle is PQ, it subtends the ∠ POQ at ‘O’ and ∠ PAQ at ‘A’ point of the circle.
Construction: Join AO and extend till ‘B’ point
Prove: The two cases are:
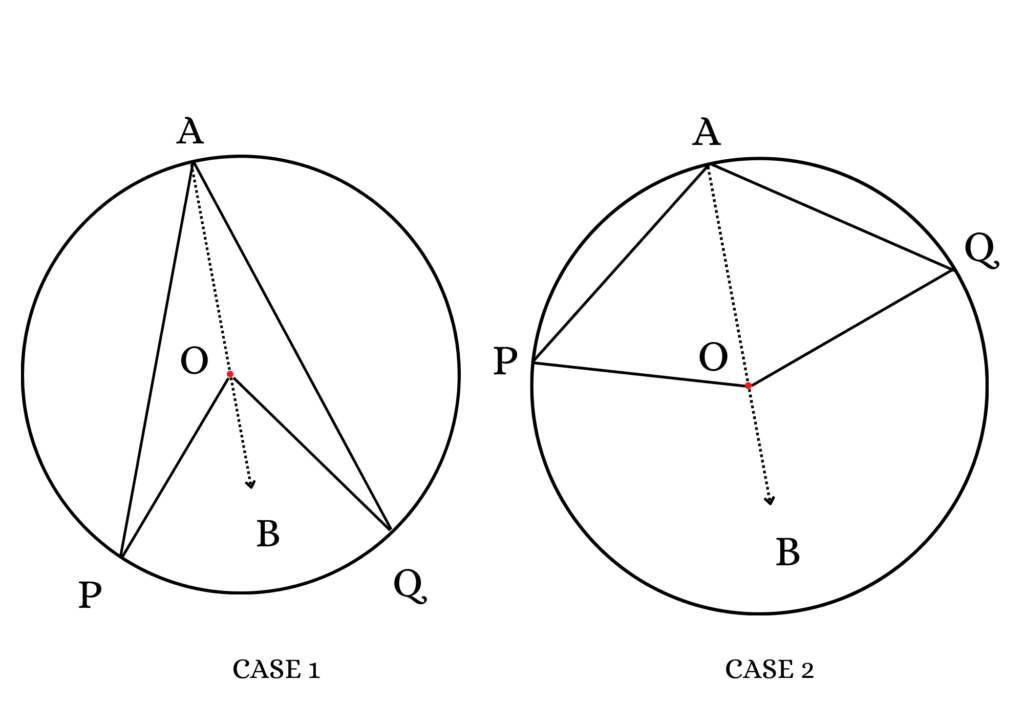
Solution (Case 1):
In △ APO,
OP = OA (Radius)
∠ OPA = ∠ OAP (the angles opposite to equal sides are equal in length) ………. (1)
Using the exterior property of angles which states that the external angle is the addition of interior opposite angles, we get,
∠ BOP = ∠ OPA + ∠ OAP
∠ BOP = ∠ OAP + ∠ OAP
∠ BOP = 2 ∠ OAP ………… (3)
In △ AQO,
OQ = OA (Radius)
∠ OQA = ∠ OAQ (the angles opposite to equal sides are equal in length) ………. (2)
Using the exterior property of angles which states that the external angle is the addition of interior opposite angles, we get,
∠ BOQ = ∠ OQA + ∠ OAQ
∠ BOP = ∠ OAQ + ∠ OAQ
∠ BOP = 2 ∠ OAQ ………… (4)
On summing up equations (3) and (4), we get,
∠ BOP + ∠ BOQ = 2 ∠ OAP + 2 ∠ OAQ
∠ POQ = 2 (∠ OAP + ∠ OAQ)
Hence, ∠ POQ = 2 ∠ PAQ
Solution (Case 2):
In △ APO,
OP = OA (Radius)
∠ OPA = ∠ OAP (the angles opposite to equal sides are equal in length) ………. (1)
Using the exterior property of angles which states that the external angle is the addition of interior opposite angles, we get,
∠ BOP = ∠ OPA + ∠ OAP
∠ BOP = ∠ OAP + ∠ OAP
∠ BOP = 2 ∠ OAP ………… (3)
In △ AQO,
OQ = OA (Radius)
∠ OQA = ∠ OAQ (the angles opposite to equal sides are equal in length) ………. (2)
Using the exterior property of angles which states that the external angle is the addition of interior opposite angles, we get,
∠ BOQ = ∠ OQA + ∠ OAQ
∠ BOP = ∠ OAQ + ∠ OAQ
∠ BOP = 2 ∠ OAQ ………… (4)
On summing up equations (3) and (4), we get,
∠ BOP + ∠ BOQ = 2 ∠ OAP + 2 ∠ OAQ
Reflex angle ∠ POQ = 2 (∠ OAP + ∠ OAQ)
Reflex angle ∠ POQ = 2 ∠ PAQ
Hence, 360° - ∠ POQ = 2 ∠ PAQ
Theorem 7: The angle subtended by a diameter or semicircle on any point of a circle is a right angle.
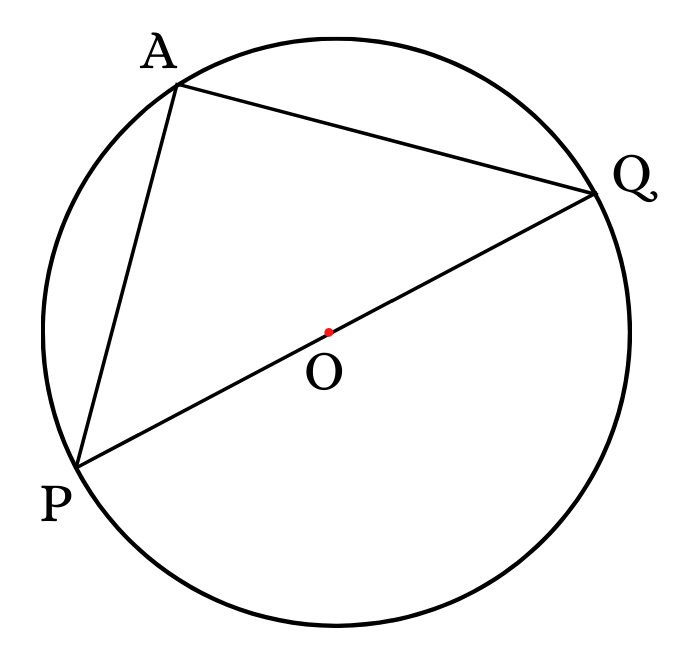
Given: A circle having center ‘O’, the diameter of the circle is PQ and it subtends the ∠ PAQ at the ‘A’ point on the circle.
Prove: That ∠ PAQ = 90°
Solution:
POQ is the straight line intersecting through the center of circle ‘O’.
So, the angle that is subtended by the arc PQ at ‘O’ is ∠ PAQ = 180° …………. (1)
According to the theorem of the chord of a circle, the angle subtended by an arc at the center is twice the angle subtended by it at any point on the remaining part of the circle.
Thus, ∠ POQ = 2 ∠ PAQ
∠ POQ /2 = ∠ PAQ
180° / 2 = ∠ PAQ
Hence, ∠ PAQ = 90°
Formulae used in Chords
The two formulas to determine the chord length of a circle:
- The length of the chord using the perpendicular distance from the center of the circle equals to, , where‘’ is the radius of the circle and ‘’ is the perpendicular distance of the right-angled triangle.
- The length of the chord using trigonometry equals to , where, ‘’ is the radius of the circle and ‘’ is the angle subtended at the chord’s central portion.
Context and application
The topic of chords is applicable in many fields such as architecture and design. Wherever we use circles, we need to know the concepts of chords as well. This topic is studied by the students who undertake courses such as Bachelor of Technology (Engineering), Masters in Science (Algebra, Geometry, and Cryptography), and Masters in Science (Mathematics and Applications).
Related topics
- Synthetic geometry
- Inverse Pythagorean Theorem
Practice problems
Q1. What is meant by the word ‘chord’ in Latin?
- chorda
- chords
- chime
- chain
Correct Option: 1.
Explanation: The word ‘chord’ in Latin means chorda. Several line segments exist but it is the chord that is the only line segment whose endpoints lie on the periphery of the circle.
Q2. What are the parts called which are produced when a chord is drawn in the circle?
- Minor sector and major sector
- Minor segment and major segment
- Minor region and major region
- Minor part and the major part
Correct Option: 2.
Explanation: When a chord is drawn in the circle, the parts called are the minor segment and major segment.
Q3. Which is the longest chord of the circle?
- Radius
- Tangent
- Diameter
- Arc
Correct Option: 3.
Explanation: The longest chord of the circle is the diameter. A chord that passes through the center of the circle is the diameter of the circle.
Q4. What does Thales’ theorem state?
- An inscribed arc extended by a radius is a right angle
- An inscribed sector extended by a chord is a right angle
- An inscribed arc extended by a tangent is a right angle
- An inscribed angle extended by a diameter is a right angle
Correct Option: 4.
Explanation: Thales’ theorem states that an inscribed angle extended by a diameter is a right angle.
Q5. Which of the following statements are incorrect?
- Equal chords extend equal angles at the center of a circle.
- There exist many circles which pass through all the three collinear points
- Equal chords of a circle are at the same distance from the center of a circle.
- In the case of a cyclic quadrilateral, the exterior angle is equal to the interior opposite angle.
Correct Option: 2.
Explanation: There exists only a single circle that passes through all the three collinear points.
Want more help with your geometry homework?
*Response times may vary by subject and question complexity. Median response time is 34 minutes for paid subscribers and may be longer for promotional offers.
Chords in Circle Homework Questions from Fellow Students
Browse our recently answered Chords in Circle homework questions.