Physics for Scientists and Engineers: Foundations and Connections
1st Edition
ISBN: 9781133939146
Author: Katz, Debora M.
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Related questions
Concept explainers
Question
thumb_up100%
For each of the situations below, determine which forms of Newton's second law (N2L) are appropriate: N2L rotation, N2L translation, either, or both.
You are asked to find the
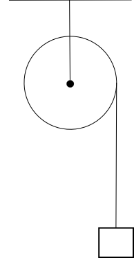
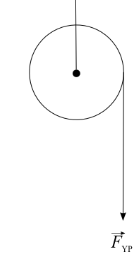
You are asked to find the angular acceleration of a low-friction pulley due to a mass hanging from it by a rope.
You are asked to determine the maximum height of a spinning ball that is thrown upwards.
You are asked to calculate the time it takes a ball to roll down an inclined plane.
You are asked to find the tangential acceleration of a small satellite in a circular orbit that results from it firing its thruster.
You are asked to determine the orbital period of a small satellite around Earth.
Transcribed Image Text:YP
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 2 steps
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.Similar questions
- An ultracentrifuge accelerates from to 100,000 rpm in 2.00 min. (a) What is the average angular acceleration in rad/s2 ? (b) What is the tangential acceleration of a point 9.50 cm from the axis of rotation? (c) What is the centripetal acceleration in m/s2 and multiples of g of this point at full rpm? (d) What is the total distance travelled by a point 9.5 cm from the axis of totation of the ultracentrifuge?arrow_forwardA system of point particles is rotating about a fixed axis at 4 rev/s. The particles are fixed with respect to each other. The masses and distances to the axis of the point particles are m1=0.1kg , r1=0.2m , m2=0.05kg , r2=0.4m , m3=0.5kg , r3=0.01m . (a) What is the moment of inertia of the system? (b) What is the rotational kinetic energy of the system?arrow_forwardSuppose when Earth was created, it was not rotating. However, after the application of a uniform torque after 6 days, it was rotating at 1 rev/day. (a) What was the angular acceleration during the 6 days? (b) What torque was applied to Earth during this period? (c) What force tangent to Earth at its equator would produce this torque?arrow_forward
- Keratinocytes are the most common cells in the skins outer layer. As these approximately circular cells migrate across a wound during the healing process, they roll in a way that reduces the frictional forces impeding their motion. (a) Given a cell body diameter of 1.00 105 m (10 m), what minimum angular speed would be required to produce the observed linear speed of 1.67 107 m/s (10 m/min)? (b) How many complete revolutions would be required for the cell to roll a distance of 5.00 103 m? (Because of slipping as the cells roll, averages of observed angular speeds and the number of complete revolutions are about three times these minimum values.)arrow_forwardIn testing an automobile tire for proper alignment, a technicianmarks a spot on the tire 0.200 m from the center. He then mountsthe tire in a vertical plane and notes that the radius vector to thespot is at an angle of 35.0 with the horizontal. Starting from rest,the tire is spun rapidly with a constant angular acceleration of 3.00 rad/s2. a. What is the angular speed of the wheel after 4.00 s? b. What is the tangential speed of the spot after 4.00 s? c. What is the magnitude of the total accleration of the spot after 4.00 s?" d. What is the angular position of the spot after 4.00 s?arrow_forwardAn aircraft is coming in for a landing at 300 meters height when the propeller falls off. The aircraft is flying at 40.0 m/s horizontally. The propeller has rotation rate of 20 rev/s, a moment of inertia fo 70.0kgm2 , and mass of 200 kg. Neglect air resistance. (a) With what translational velocity does the propeller hit the ground? (b) What is the rotation rate of the propeller at impact?arrow_forward
- A flywheel (l=50kgm2) starting from rest acquires an angular velocity of 200.0 rad/s while subject to a constant torque from a motor for 5 s. (a) What is the angular acceleration of the flywhell? (b) What is the magnitude of the torque?arrow_forwardUnreasonable Results You are told that a basketball player spins the ball with an angular acceleration of 100 rad/s2. (a) What is the ball's final angular velocity if the ball stairs from rest and the acceleration lasts 2.00 s? (b) What is unreasonable about the result? (c) Which premises are unreasonable or inconsistent?arrow_forwardConsider an object on a rotating disk a distance r from its center, held in place on the disk by static friction. Which of the following statements is not true concerning this object? (a) If the angular speed is constant, the object must have constant tangential speed. (b) If the angular speed is constant, the object is not accelerated. (c) The object has a tangential acceleration only if the disk has an angular acceleration. (d) If the disk has an angular acceleration, the object has both a centripetal acceleration and a tangential acceleration. (e) The object always has a centripetal acceleration except when the angular speed is zero.arrow_forward
- Suppose you exert a force of 180 N tangential to a 0.280-m-radius, 75.0-kg grindstone (a solid disk). (a) What torque is exerted? (b) What is the angular acceleration assuming negligible opposing friction? (c) What is the angular acceleration if there is an opposing frictional force of 20.0 N exerted 1.50 cm from the axis?arrow_forwardAs shown in Figure OQ10.7, a cord is wrapped onto a cylindrical reel mounted on a fixed, friction less, horizontal axle. When does the reel have a greater magnitude of angular acceleration? (a) When the cord is pulled down with a constant force of 50 N. (b) When an object of weight 50 X is hung from the cord and released, (c) The angular accelerations in parts (a) and (b) are equal, (d) It is impossible to determine.arrow_forwardA bicyclist is testing a new racing bike on a circular track ofradius 53.0 m. The bicyclist is able to maintain a constant speedof 24.0 m/s throughout the test run. a. What is the angularspeed of the bike? b. What are the magnitude and direction ofthe bikes acceleration?arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
- Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University
College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning 
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
College PhysicsPhysicsISBN:9781938168000Author:Paul Peter Urone, Roger HinrichsPublisher:OpenStax College
Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...
Physics
ISBN:9781133939146
Author:Katz, Debora M.
Publisher:Cengage Learning
University Physics Volume 1
Physics
ISBN:9781938168277
Author:William Moebs, Samuel J. Ling, Jeff Sanny
Publisher:OpenStax - Rice University
College Physics
Physics
ISBN:9781305952300
Author:Raymond A. Serway, Chris Vuille
Publisher:Cengage Learning
Principles of Physics: A Calculus-Based Text
Physics
ISBN:9781133104261
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
College Physics
Physics
ISBN:9781938168000
Author:Paul Peter Urone, Roger Hinrichs
Publisher:OpenStax College
Physics for Scientists and Engineers, Technology ...
Physics
ISBN:9781305116399
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning