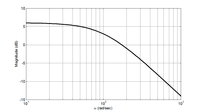
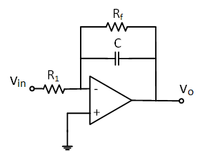
You would like to design the lowpass filter above that has a frequency response shown by the Bode plot below.   For a lowpass filter, the passband (the frequency range where signals are passed) is at low frequencies and the stop band (the frequency range where signals are attenuated) is at high frequencies. The corner frequency marks the transition between passband and stopband.  If Rf= 5000Ω you will determine the values of R1 and C  to complete the design. The design is done in steps.  First, determine  the corner frequency (or bandwidth) in rad/sec.  Enter Wb in the box below.   Enter the magnitude of the passband gain, KDC. Recall that a gain in dB is related to the linear gain  by the formula KdB = 20log10|k|.   Enter the value for R1 in ohms . Enter the value for C in μF.

Q: Complete Part A, Thank you! I will give a great rating!

A: Step 1:

Q: Bode Diagram 20 10 -10 -20 -30- 40 -50- -60 -70 -80 -45 -90- -135 -180 102 101 10° Frequency (rad/s)…

A: (a) Phase crossover frequency: The frequency at which the phase of the bode plot becomes -1800 is…

Q: 7. The bode plot of a certain transfer function is given below. From the plot determine; Magnitude…

A: Since you have posted a question with multiple sub-parts, we will solve first three sub-parts for…

Q: The critical frequency of a Bode Plot is 338 kHz. This filter passes low frequencies and will not…

A:

Q: Figure Q3(a) shows an approximate and actual Bode Plot for magnitude and phase. From the given Bode…

A: So for solving this question, we will find the gain margin and phase margin. Therefore, by suing…

Q: -20 dB/decade A, -40 dB/decade fOH

A: Given the waveform: We need to design the filter circuit.

Q: 16V for the given circuit: Fake 5ka a) Set the re find AVmid = loke c) zi= ? n 313

A: Note :    It is the kind notice that according to the guidelines of the company we supposed to…

Q: B: For the circuit shown, is this a possible Bode Plot? R1 www Va *I C1 Vb 40db Gain Frequency

A: Yes this is a possible bode plot.   Because transfer function of the circuit will be    H(s) =…

Q: The frequency responses of the filter F1 and F2 are given below and they are connected in the…

A: In this question, choose the correct option What is the value of resultant circuit if F1 and F2…

Q: Q2: Design a basic RC band stop filter with a lower cut-off frequency of 2001z and a higher cut- off…

A: It is given that: fL=200Hzfh=800Hz

Q: 1. For the series circuit below find frequency that would create resonance (fs), the Quality Factor…

A: Given that,

Q: Draw bode plof and determine gain margin and phase margin G(s)H(s) aa 720(st125) G10)(+2+9)

A: The bode plot of the system will be plotted as:-

Q: Draw polar plot for the open loop function given. Determine Phase margin, Gain  margin, Phase cross…

A:

Q: [Q3] Choose appropriate values for this Sallen-Key high-pass filter circuit to give it a cutoff…

A:

Q: Find the transfer function for the circuit shown in the figure. Plot the amplitude graph of…

A:

Q: A filter section is to have a characteristic impedance at zero frequency of 600 ohm and a cut-off…

A:

Q: 45 9 20 с) 40 10 101 -135 10° 10 10 Frequency - w, rad-sec 10 10 10° 10 102 Frequency - w, rad-sec…

A:

Q: 4. Figure 6 shows a filter circuit diagram. Answer the following questions: H C R out Figure 6 If R…

A:

Q: Below you see a Bode diagrams and a Nyquist diagrams of the same systems G2. Mark the correct…

A: From the analysis of the bode plot and Nyquist plot, we can understand the stability of the system…

Q: Solve the following: Find the phase margin and zero dB frequency. i. ii. Use your results in…

A:

Q: QUESTION Proposed an industrial application with a transfer function. Using the proposed transfer…

A: Taking transfer function as

Q: Design an active band pass filter with Gain= -20 and cutoff frequencies at 100 Hz and 10 kHz using…

A: The band pass filter will allow only range of frequency and reject the remaining frequency.

Q: Vin(t) = 5 cos(250t) + 5cos(2000t) + 5cos(20,000rt) R = 1000 = 318.3 N TT + C = 1 µF Vin(1) Vout(t)…

A: “Since you have asked multiple question, we will solve the first question for you. If you want any…

Q: Use a 36-mH inductor to design a low-pass filter having a cutoff frequency of 36 kHz. Draw a…

A:

Q: For the bode plot attached to this question. Can you please work out the approximate damping ratio,…

A: Given bode plot  Gain margin is defined as the amount of change in open-loop gain needed to make a…

Q: I need some help. I am designing a bandpass filter with fl=3400 Hz and fh= 7500 Hz. Based on that I…

A: In this question,   We need to determine the center frequency, bandwith, Q, and RLC parameters for…

Q: Calculate the values of L and C in part A. Make it clear how you found them

A:

Q: o filter out some noise and undesirable stray signals, a passive band-pass filter was utilised. When…

A:

Q: 5. Some circuits (a) Use E = L to calculate the impedance of an inductor. (b) Caleulate the transfer…

A:

Q: Use the bode plot shown above to determine the approximate Gain crossover frequency wc, phase…

A: The Bode magnitude and phase plot is given and from that, we have to analyze the gain crossover…

Q: Find the transfer function for the circuit shown in the figure. Plot the amplitude graph of…

A:

Q: 4. The Bode plot shown below represents the voltage gain of a particular amplifier. Sketch the input…

A: Consider the input of the amplifier, The input of the amplifier is given. If the magnitude and the…

Q: 9 - In Bode diagram the frequency at which the two asymptotes meet is called ... O A) the corner…

A: In this question we need to choose a correct option

Q: no ohms

A: GivenVs=50mvR=220ΩXL=100ΩXC=100Ω

Q: Q5/ design LPF with R= 4KQand C=40nF then calculate cutoff frequency and draw the frequency response…

A: We need to analyse the low pass filter .

Q: Required information A parallel resonance circuit has a resistance of 2 kQ and half-power…

A:

Q: Complete the design of the following active low pass filter. Choose values for R4 and R7 to get…

A:

Filter

A filter is a device or process which removes some of the unwanted features or components like frequencies from a signal in signal processing. The feature of filters is the complete or partial suppression of some part of the signal, and filtering is a class of signal processing. This most often means removing some frequency bands or some frequencies. In the frequency domain, the filters do not exclusively act, especially in the image processing field many other targets for filtering exist. Without having to act in the frequency domain, the correlations can be removed for certain frequency components and not for others. The filters are widely used in electronics and telecommunication, in television, radio, radar, audio recording, music synthesis, control systems, computer graphics, and image processing.