Bartleby Related Questions Icon

Related questions

Q: can you explain further, inside a finite well, the wave function is either cosine or sine, so…
A: For symmetric potential we can generalled the form of the wave function is either cosine or sine.…
Q: A particle of mass m is confined to a one-dimensional potential well. The potential energy U is 0…
A:
Q: How is Ro related to L? Where, mr = 0 and R GmM mR
A: Solution Given, GmMR02=LmR03 The relation between R0 and L can be calculated as follows…
Q: The wave function of a particle at time t=0 is given by|w(0)) = (u,) +|u2}), where |u,) and u,) are…
A:
Q: Don't use chat gpt It
A:
Q: Why don't you include the time dependent part of the wave equation when finding the expectation…
A:
Q: A particle is trapped in an infinite one-dimensional well of width L. If the particle is in its…
A:
Q: Calculate the average position of the particle in a cube with length L for the ground and first…
A:
Q: A quantum mechanical particle is confined to a one-dimensional infinite potential well described by…
A: Step 1: Given: Particle in a 1-D infinite potential well described by the potential:V(x) =0,…
Q: If the absolute value of the wave function of a proton is 2 times as large at location A than at…
A:
Q: goes from -∞ to +∞. salg 0.1 loe 18. Normalize the wavefunction, = (2-)e . alpos 90
A:
Q: 2, Given Ax (a-x), A, a are limit orxa at to (x,0) 2- If (NY/(x, 0) RAM Constant 1- Find A for…
A:
Q: Suppose you measure A with eigenvalues A1, 2, and X3 with corresponding eigenvectors |1), |2), and…
A: Solution:   Given that, Normalized wave function (ψ)=α |1>+β|2>+γ|3>
Q: What does population vector, Π=(P1,P2,P3r,P3w)T mean ? How do this formula describe the overall…
A: The population vector Π represents the probability distribution over a set of discrete states. In…
Q: A particle inside an infinite square well ( a = 1 ) start at the initial state Y(x, 0) = v3(1 – x)0…
A: (a)
Question
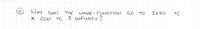
WHY DOES THE WAVE- FUNCTION GO TO ZERO AS X GOES TO * INFINITY ?
expand button
Transcribed Image Text:WHY DOES THE WAVE- FUNCTION GO TO ZERO AS X GOES TO * INFINITY ?
Expert Solution
Check Mark
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
See solutionCheck out a sample Q&A here
Step 1
VIEW
Step 2
VIEW
bartleby

Step by stepSolved in 2 steps

See solution
Check out a sample Q&A here
Blurred answer
Knowledge Booster
Background pattern image
Similar questions