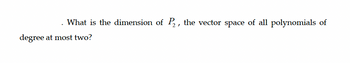
What is the dimension of P₂, the vector space of all polynomials of degree at most two?

Let V be the set of all positive real numbers. Determine whether V is a vector space with the operations shown below. x+y=xyAddition cx=xcScalar multiplication If it is, verify each vector space axiom; if it is not, state all vector space axioms that fail.

Find a basis for the vector space of all 33 diagonal matrices. What is the dimension of this vector space?

Let P2 be the vector space of all polynomials of degree ≤ 2 with coefficients in R, andS= {1 + 2x, 1 + 3x + 5x^2 , 4x + 5x^2 } .Is Span(S)= P2?

Show that the set of all real polynomials with a degree n < 3 associated with the addition of polynomials and the multiplication of polynomials by a scalar form a vector space.

Why do the set of all polynomials of degree n or less form a vector space, while the set of all polynomials of degree n or more do not?

What is the dimension of the complexification VC thought of as a real vector space?

Show that the vectors are linearly dependent or linearly independent. note: {2t^2 − 1, t + 1},P2:The space of all polynomials with a degree less than 2 and 2

Is the set of rational numbers with the usual definitions and scalar multiplication a vector space? Please show justification for answer and explain steps.

Show that the set of all pairs of real numbers (x, y) with the operations (X1, Y1) + (x2, Y2) = (x1 + x2 + 1, y1 + Y2+ 1) and k (*1, Y1) = (kx1, kyı) is not a vector space.