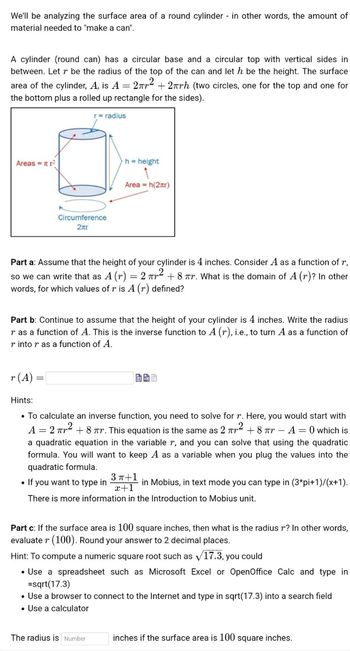
We'll be analyzing the surface area of a round cylinder - in other words, the amount o material needed to "make a can". A cylinder (round can) has a circular base and a circular top with vertical sides between. Let r be the radius of the top of the can and let h be the height. The surfac area of the cylinder, A, is A = 2rr2+2πrh (two circles, one for the top and one fo the bottom plus a rolled up rectangle for the sides). r-radius Areas = r² Circumference 2лr r (A) = h-height Part a: Assume that the height of your cylinder is 4 inches. Consider A as a function of so we can write that as A (r) = 2 r2 +8 r. What is the domain of A (r)? In othe words, for which values of r is A (r) defined? Area - h(2x) Part b: Continue to assume that the height of your cylinder is 4 inches. Write the radiu r as a function of A. This is the inverse function to A (r), i.e., to turn A as a function o r into r as a function of A. Hints: • To calculate an inverse function, you need to solve for r. Here, you would start with A = 22 +8 πr. This equation is the same as 2 r2 +8 πr-A=0 which i a quadratic equation in the variable r, and you can solve that using the quadrati formula. You will want to keep A as a variable when you plug the values into th quadratic formula. • If you want to type in 3+1 in Mobius, in text mode you can type in (3*pi+1)/(x+1) x+1 There is more information in the Introduction to Mobius unit. Part c: If the surface area is 100 square inches, then what is the radius r? In other words evaluate r (100). Round your answer to 2 decimal places. Hint: To compute a numeric square root such as √17.3, you could The radius is Number • Use a spreadsheet such as Microsoft Excel or OpenOffice Calc and type i = sqrt(17.3) • Use a browser to connect to the Internet and type in sqrt(17.3) into a search field . Use a calculator inches if the surface area is 100 square inches.