Introduction to Chemical Engineering Thermodynamics
Introduction to Chemical Engineering Thermodynamics
8th Edition
ISBN: 9781259696527
Author: J.M. Smith Termodinamica en ingenieria quimica, Hendrick C Van Ness, Michael Abbott, Mark Swihart
Publisher: McGraw-Hill Education
Bartleby Related Questions Icon

Related questions

Q: 2) Describe the different types of fluids and illustrate them on a graph of shear stress vs du/dy.…
A:
Q: 4. Rework inviscid flow past a cylinder in terms of the stream function y.
A: The inviscid flow past a cylinder in terms of stream function is to be mentioned.
Q: Question 5 The velocity distribution for a laminar, incompressible flow of a Newtonian fluid in a…
A:
Q: Which of the following method measures spatial variations in the earth?
A:
Q: Ex. Which arrow indicates Tyx? А. В. C. / С. D. None of the above N
A: Dear user, since you have asked two different question ,according to our bartleby rule ,we can solve…
Q: Derive the velocity profile of the fluid inside the two stationary plates. The plate is initially…
A: Considering a small volume of a cube and velocity profile is obtained then it is integrated to…
Q: Shear stress profile for a Newtonian fluid flowing in a circular pipe is O A. Linear В. Parabolic…
A:
Q: For the Eulerian flow field given in Cartesian coordinates by u = −2x2, v = 2z3,w = 4xz: (a)…
A: Since you have posted a question with multiple sub-parts, we will solve first three sub-parts for…
Q: In the derivation of the equation of motion, the "no-slip" condition is no longer valid and…
A: To state whether the given statement is true or false
Q: Write down the 2 key parameters that affect the friction losses of the fluid flowing through a…
A:
Q: Which of the following statement is FALSE? Flow is described as uniform if the velocity, pressure…
A: OPTION "A" , C, D, E are true statement.  Velocity of a fluid at each point is parallel to a fixed…
Q: If the shear stress profile of a fluid flows within laminar boundary layer is given by (1-) 21 U T =…
A:
Q: An incompressible fluid is confined between two infinite, parallel horizontal plates separated by a…
A: Note: as per our guidelines, we are supposed to solve only 3 subparts of a question. please send the…
Q: Which of the following statement is FALSE? (A) At steady uniform flow, conditions do not change with…
A: The flow behavior of fluids is the base for fluid mechanics. The fluid analysis and all calculations…
Q: Q3. A Newtonian Fluid flows between two parallel plates, a distance 2h apart. The upper plate moves…
A:
Q: A fluid whose apparent viscosity increases with shear rate is termed as the fluid. Viscous…
A: Viscosity is a fluid [property. This property studies liquid motion behavior. The measure of the…
Q: The pressure decreases across the length of a converging nozzle when the velocity of sound is…
A: The converging nozzle accelerates the flow whenever required. This is used in aircraft, dams, and in…
Q: The equation of motion is reduced to what equation if the fluid has constant density and viscosity?…
A: Write the expression for the equation of motion. ρdVdt=−∇→P−∇→.τ+ρg…
Q: Steady, uniform, and laminar flow of a fluid with dynamic viscosity (n) occurs between two…
A: A fluid flows between two horizontal, infinite, parallel plates separated by distance h.
Q: The velocity distribution for a laminar, incompressible flow of a Newtonian fluid in a long vertical…
A:
Q: What is the approximation of fick's equation
A: The approximation of Fick’s law are as follows: Flux is taken only in one direction in which the…
Q: A liquid flows between two paralell plates which are inclined against the horizontal by an angle α.…
A: For the given system, the schematic diagram is prepared as:
Q: The initial free fall of water from a faucet can be thought of as an inviscid flow because friction…
A: Given: The distance between points 1 and 2 is 7 cm i.e. z1 - z2 = 7 cm or 0.07 m The diameter of…
Q: 1. Define the physical meaning in each following term of the equations: a) Equation of continuity…
A: Equation of Continuity is based on the principle of conservation of mass, while the equation of…
Q: HOMEWORK Derive the velocity profile of the fluid inside the two stationary plates. The plate is…
A:
Q: Derive Navier-Stokes equation of fluid mechanics from fundamentals. Give two different but real…
A: Navier Stokes Equation derivation  Consider an infinitesimally small fluid element .The region of…
Q: The 800-N force F is applied to the vertical pole as shown. y 30° F 30° it
A: Given data: F=800 N Unit vectors in x axis i and in y axis j
Q: A Newtonian fluid with constant density flows in a parallel-plate apparatus that separated by a…
A:
Q: Consider steady state, laminar flow of a fluid in the y direction between two parallel and vertical…
A:
Q: A disk 15 cm in diameter is placed in a wind tunnel at right angles to the incoming flow. The drag…
A: The drag force on the disk when water is flowing through it is to be estimated.
Q: 2- The steady state flow of a liquid əx Space between two very large static parallel plates at a…
A: A steady state flow of fluid is flowing between two very large parallel plates in the…
Q: In order to understand all issues regarding the analysis of flow around a sphere, first (a) Argue…
A: Hello!  Since you have posted a question with multiple sub-parts, we will solve first three subparts…
Q: . I change my experiment around a bit, so that it will allow me to change the viscosity of the fluid…
A: a)Reynolds number is given as Re=ρuDμ Where ρ- Density of fluid μ- Dynamic viscosity u- Average…
Q: measures local or point velocities by measuring the pressure difference between the total pressure…
A: We have to answer which instrument is used to measure the point or local velocity by measuring the…
Q: For flow over a flat plate wherein a laminar boundary layer is present for the case of a zero-…
A:
Q: A liquid flows under steady state conditions along an open channel of a fixed inclined planeto the…
A: Given Question is explained in detail.Explanation:Step 1: a) Factors affecting the depth of the…
Q: Water is in steady fully developed laminar flow between two horizontal, very wide (W) and long (L)…
A:
Q: Pascal second is the Unit of Oa. Viscosity O b. Surface tension O c. kinematic viscosity O d. Power
A: b) Surface tension:   SI Unit: N/m CGS Unit: dyne/cm kinematic viscosity (m2 s−1). The English…
Q: The distance between the two stationary parallel plates of a fluid is h. Both plates have width W…
A: The schematic diagram for the flow of fluid between parallel plates with all the given information…
Q: two plates which are aligned parallel above eachother.The top plate is moving horizontally with a…
A: A Newtonian fluid is flowing between two parallel plates where top plate is moving with a constant…
Question

Consider the following system where the fluid is moving upward in an annulus of height L using cylindrical coordinates

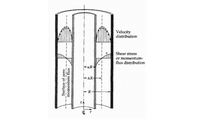
Velocity distribution Shear stress or momentum- flux distribution AR Surface of zero momentum flux
expand button
Transcribed Image Text:Velocity distribution Shear stress or momentum- flux distribution AR Surface of zero momentum flux
Expert Solution
Check Mark
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
See solutionCheck out a sample Q&A here
Step 1
VIEW
Step 2
VIEW
bartleby

Step by stepSolved in 2 steps

See solution
Check out a sample Q&A here
Blurred answer
Knowledge Booster
Background pattern image
Recommended textbooks for you
Text book image
Introduction to Chemical Engineering Thermodynami...
Chemical Engineering
ISBN:9781259696527
Author:J.M. Smith Termodinamica en ingenieria quimica, Hendrick C Van Ness, Michael Abbott, Mark Swihart
Publisher:McGraw-Hill Education
Text book image
Elementary Principles of Chemical Processes, Bind...
Chemical Engineering
ISBN:9781118431221
Author:Richard M. Felder, Ronald W. Rousseau, Lisa G. Bullard
Publisher:WILEY
Text book image
Elements of Chemical Reaction Engineering (5th Ed...
Chemical Engineering
ISBN:9780133887518
Author:H. Scott Fogler
Publisher:Prentice Hall
Text book image
Process Dynamics and Control, 4e
Chemical Engineering
ISBN:9781119285915
Author:Seborg
Publisher:WILEY
Text book image
Industrial Plastics: Theory and Applications
Chemical Engineering
ISBN:9781285061238
Author:Lokensgard, Erik
Publisher:Delmar Cengage Learning
Text book image
Unit Operations of Chemical Engineering
Chemical Engineering
ISBN:9780072848236
Author:Warren McCabe, Julian C. Smith, Peter Harriott
Publisher:McGraw-Hill Companies, The
SEE MORE TEXTBOOKS