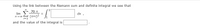
Using the link between the Riemann sum and definite integral we se 70 n dx , lim n- co (n+1)2

No → C below +11 10 MMX MM Gar G SEG pl G SE + myopenmath.com/assess2/?cid=175669&aid=1.. The rectangles in the graph below illustrate a left endpoint Riemann sum for -x² f(x) = + 4x on the interval [3, 7] 2 9 8 7 6 5 4 3- 2 1 1 2 3 4 5 6 7 V The value of this left endpoint Riemann sum is 57.6 M-Ox OX ☐ ⠀ X 0 This Riemann sum is insufficient information of the area beneath the curve on [3, 7] Question Help: Video Message instructor O th.com/a Appro 117 Mc 0:27/5:14 n ME

Evaluate the definite integral 플 2 sin (a) do 4 Give your answer exactly, i.e.as an integer, fraction ar using surds.