Advanced Engineering Mathematics
10th Edition
ISBN: 9780470458365
Author: Erwin Kreyszig
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Related questions
Question
thumb_up100%
Hello. I wanted to make sure I am on the write track with this problem. The t^2 should become odd and cos becomes odd? How would you go about this problem?
Thank you for the help and your time.
Transcribed Image Text:Untitled document - Google Docs X
← → с
✰ https://docs.google.com/document/d/1kBg0GQ6JxWly68MgSGaPYKg0gmnwf6rA8i4xpmy_9Ho/edit
Search the menus (Alt+/)
+
Type here to search
1000|
√2
1
0.1*
100%
Normal text
Arial
2 | 13|
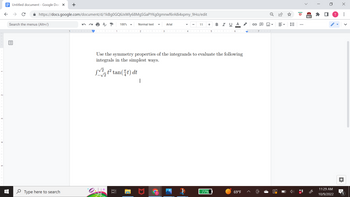
Use the symmetry properties of the integrands to evaluate the following
integrals in the simplest ways.
t² tan(t) dt
|
11 + B I U A
4 CO 5617|
77%
→
69°F
2
E-
im
ID
901
n
11:29 AM
10/9/2022
X
:
Expert Solution
arrow_forward
Step 1
Properties of integration
The function f is odd if .
The function f is even if .
Step by stepSolved in 2 steps
Knowledge Booster
Similar questions
- Hi there I need some help solving the second part to this problem. Let f(x)=x^2+8x A.) Evaluate f(4)= B.) Solve f(x)=-16 For A, I got 48 which is correct, but I cannot seem to figure out part B. Any help would be greatly appreciated. Thank you.arrow_forwardA roasted turkey is taken from an oven when its temperature has reached 185° Fahrenheit and is placed on a table in a room where the temperature is 75° Fahrenheit. Give answers accurate to at least 2 decimal places. (a) If the temperature of the turkey is 156° Fahrenheit after half an hour, what is its temperature after 45 minutes? Fahrenheit (b) When will the turkey cool to 100° Fahrenheit? hours.arrow_forwardFor each of the following, find y^1 You do not need to simplify your answer. PLEASE attach an image so that I can understand each and every step.arrow_forward
- Can someone please explain the steps to this one please?arrow_forwardQuestion 3. Please show your work. Also it should not be p(x) equation it should be P(A) instead so the equation is actually Let p(A) = 2x^2 - 3x + 4. Thanks. Sorry for the confusion.arrow_forwardA roasted turkey is taken from an oven when its temperature has reached 185° Fahrenheit and is placed on a table in a room where the temperature is 75° Fahrenheit. Give answers accurate to at least 2 decimal places. (a) If the temperature of the turkey is 149° Fahrenheit after half an hour, what is its temperature after 45 minutes? Fahrenheit (b) When will the turkey cool to 100° Fahrenheit? hours.arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
- Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY 
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Advanced Engineering Mathematics
Advanced Math
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Wiley, John & Sons, Incorporated
Numerical Methods for Engineers
Advanced Math
ISBN:9780073397924
Author:Steven C. Chapra Dr., Raymond P. Canale
Publisher:McGraw-Hill Education
Introductory Mathematics for Engineering Applicat...
Advanced Math
ISBN:9781118141809
Author:Nathan Klingbeil
Publisher:WILEY
Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,