
Advanced Engineering Mathematics
10th Edition
ISBN: 9780470458365
Author: Erwin Kreyszig
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Concept explainers
Question
Use Newton's Method to approximate the zeros.

Transcribed Image Text:Use Newton's Method to approximate the zero(s) of the function f(x) =x' +x+1
accurate to three decimal places.
Expert Solution
arrow_forward
Step 1

arrow_forward
Step 2
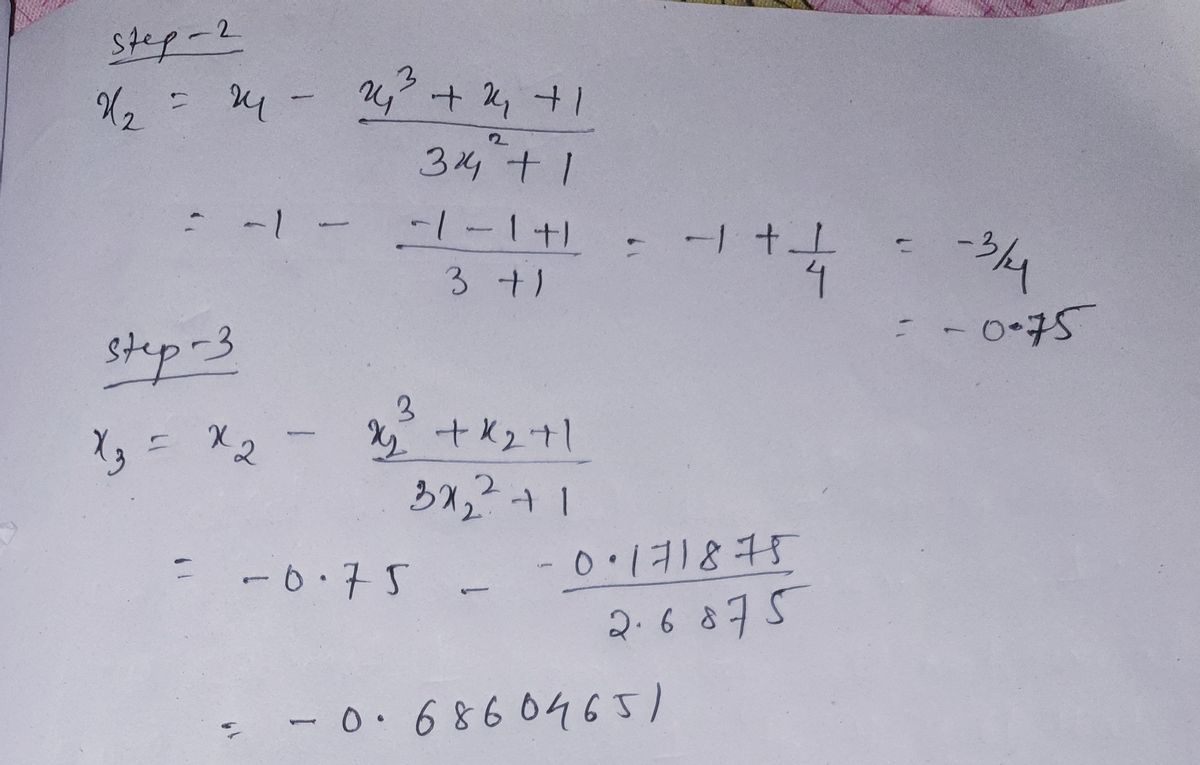
Step by stepSolved in 3 steps with 3 images

Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, advanced-math and related others by exploring similar questions and additional content below.Similar questions
- Use Newton's method to approximate a root of the equation 5x³ + 6x + 4 = 0 as follows. Let x1 = - 1 be the initial approximation. The second approximation x2 is and the third approximation x3 is (Although these are approximations of the root, enter exact expressions for each approximation.)arrow_forwardplease show full workarrow_forwardUse Newton's method to estimate the two zeros of the following function. Start with x = 0 for the left-hand zero and Xo = 5 for the right-hand zero. Then for each case, find x2. 2x-x² +4 If x = 0, then x2 (Do not round until the final answer. Then round to five decimal places as needed.) =arrow_forward
- 03:A: Estimate the roots of the function f(x) = x² + x-3. by using Newton's method. B: Use the Newton-Raphson method of finding roots of | f(x) = 3x³ - 1.arrow_forwardUse Newton's method to approximate a root of the equation 2x + 5x + 3 = 0 as follows. Let r1 = 1 be the initial approximation. The second approximation x2 is and the third approximation x3 is (Although these are approximations of the root, enter exact expressions for each approximation.)arrow_forwardPlease don't provide handwritten solution ....arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,


Advanced Engineering Mathematics
Advanced Math
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:9780073397924
Author:Steven C. Chapra Dr., Raymond P. Canale
Publisher:McGraw-Hill Education

Introductory Mathematics for Engineering Applicat...
Advanced Math
ISBN:9781118141809
Author:Nathan Klingbeil
Publisher:WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,

