1 Units, Trigonometry. And Vectors 2 Motion In One Dimension 3 Motion In Two Dimensions 4 Newton's Laws Of Motion 5 Energy 6 Momentum, Impulse, And Collisions 7 Rotational Motion And Gravitation 8 Rotational Equilibrium And Dynamics 9 Fluids And Solids 10 Thermal Physics 11 Energy In Thermal Processes 12 The Laws Of Thermodynamics 13 Vibrations And Waves 14 Sound 15 Electric Forces And Fields 16 Electrical Energy And Capacitance 17 Current And Resistance 18 Direct-Current Circuits 19 Magnetism 20 Induced Voltages And Inductance 21 Alternating-Current Circuits And Electromagnetic Waves 22 Reflection And Refraction Of Light 23 Mirrors And Lenses 24 Wave Optics 25 Optical Instruments 26 Relativity 27 Quantum Physics 28 Atomic Physics 29 Nuclear Physics 30 Nuclear Energy And Elementary Particles expand_more
1.1 Standards Of Length, Mass, And Time 1.2 The Building Blocks Of Matter 1.3 Dimensional Analysis 1.4 Uncertainty In Measurement And Significant Figures 1.5 Unit Conversions For Physical Quantities 1.6 Estimates And Order-of-Magnitude Calculations 1.7 Coordinate Systems 1.8 Trigonometry Review 1.9 Vectors 1.10 Cornoonentsofavector 1.11 Problem-Solving Strategy Chapter Questions expand_more
Problem 1CQ: Estimate the order of magnitude of the length, in meters, of each of the following; (a) a mouse, (b)... Problem 2CQ: What types of natural phenomena could serve as time standards? Problem 3CQ: Find the order of magnitude of your age in seconds. Problem 4CQ: An object with a mass of 1 kg weighs approximately 2 lb. Use this information to estimate the mass... Problem 5CQ: (a) Estimate the number of times your heart beats in a month, (b) Estimate the number of human... Problem 6CQ: Estimate the number of atoms in 1 cm5 of a solid. (Note that the diameter of an atom is about 1010... Problem 7CQ: Lacking modern timepieces, early experimenters some-times measured time intervals with their pulse.... Problem 8CQ Problem 9CQ Problem 10CQ: List some advantages of the metric system of units over most other systems of units. Problem 11CQ: Estimate the time duration of each of the following in the suggested units in parentheses: (a) a... Problem 12CQ: Suppose two quantities, A and B, have different dimensions. Determine which of the following... Problem 13CQ: Answer each question yes or no. Must two quantities have the same dimensions (a) if you are adding... Problem 14CQ: Two different measuring devices are used by students to measure the length of a metal rod. Students... Problem 15CQ: If B is added to A, under what conditions does the resultant vector have a magnitude equal to A + B?... Problem 16CQ: Under what circumstances would a vector have components that are equal in magnitude? Problem 1P: The period of a simple pendulum, defined as the time necessary for one complete oscillation, is... Problem 2P: (a) Suppose the displacement of an object is related to time according to the expression x = Bt2.... Problem 3P: A shape that covers an area A and has a uniform height h has a volume V = Ah. (a) Show that V = Ah... Problem 4P: Each of the following equations was given by a student during an examination: (a) 12mv2=12mv02+mgh... Problem 5P Problem 6P: Kinetic energy KE (Topic 5) has dimensions kg m2/s2. It can be written in terms of the momentum p... Problem 7P: A rectangular airstrip measures 32.30 in by 210 m, with the width measured more accurately than the... Problem 8P: Use the rules for significant figures to find the answer to the addition problem 21.4 + 15 + 17.17 +... Problem 9P: A carpet is to be installed in a room of length 9.72 m and width 5.3 m. Find the area of the mom... Problem 10P Problem 11P: How many significant figures are there in (a) 78.9 0.2, (b) 3.788 109, (c) 2.46 1026, (d) 0.003 2 Problem 12P: The speed of light is now defined to be 2.997 924 58 108 m/s. Express the speed of light to (a)... Problem 13P: A rectangle has a length of (2.0 0.2) m and a width of (1.5 0.1) m. Calculate (a) the area and (b)... Problem 14P: The radius of a circle is measured to be (10.5 0.2) m. Calculate (a) the area and (b) the... Problem 15P: The edges of a shoebox are measured to be 11.4 cm, 17.8 cm, and 29 cm. Determine the volume of the... Problem 16P: Carry out the following arithmetic operations: (a) the sum of the measured values 756, 37.2, 0.83,... Problem 17P: The Roman cubitus is an ancient unit of measure equivalent to about 0.445 m. Convert the 2.00m... Problem 18P: A house is advertised as having 1 420 square feet under roof. What is the area of this house in... Problem 19P: A fathom is a unit of length, usually reserved for measuring the depth of water. A fathom is... Problem 20P: A small turtle moves at a speed of 186 furlongs per fortnight. Find the speed of the turtle in... Problem 21P: A firkin is an old British unit of volume equal to 9 gallons. How many cubic meters are there in... Problem 22P: Find the height or length of these natural wonders in kilometers, meters, and centimeters: (a) The... Problem 23P: A car is traveling at a speed of 38.0 m/s on an interstate high-way where the speed limit is 75.0... Problem 24P: A certain car has a fuel efficiency of 25.0 miles per gallon (mi/gal). Express this efficiency in... Problem 25P: The diameter of a sphere is measured to be 5.36 in. Find (a) the radius of the sphere in... Problem 26P: Suppose your hair grows at the rate of 1/32 inch per day. Find the rate at which it grows in... Problem 27P: The speed of light is about 3.00 108 m/s. Convert this figure to miles per hour. Problem 28P: A house is 50.0 ft long and 26 ft wide and has 8.0-ft-high ceilings. What is the volume of the... Problem 29P: The amount of water in reservoirs is often measured in acre-ft. One acre-ft is a volume that covers... Problem 30P: The base of a pyramid covers an area of 13.0 acres (1 acre = 43 560 ft2) and has a height of 481 ft... Problem 31P: A quart container of ice cream is to be made in the form of a cube. What should be the length of a... Problem 32P: Estimate the number of steps you would have to take to walk a distance equal to the circumference of... Problem 33P: Estimate the number of breaths taken by a human being during an average lifetime. Problem 34P: Estimate the number of people in the world who are suffering from the common cold on any given day.... Problem 35P: The habitable part of Earths surface has been estimated to cover 60 trillion square meters. Estimate... Problem 36P: Treat a cell in a human as a sphere of radius 1.0 m. (a) Determine the volume of a cell. (b)... Problem 37P: An automobile tire is rated to last for 50 000 miles. Estimate the number of revolutions the tire... Problem 38P: A study from the National Institutes of Health stales that the human body contains trillions of... Problem 39P: A point is located in a polar coordinate system by the coordinates r = 2.5 m and = 35. Find the x-... Problem 40P: A certain corner of a room is selected as the origin of a rectangular coordinate system. If a fly is... Problem 41P: Express the location of the fly in Problem 40 in polar coordinates. Problem 42P: Two points in a rectangular coordinate system have the coordinates (5.0, 3.0) and (3.0, 4.0), where... Problem 43P: Two points are given in polar coordinates by (r, ) = (2.00 m, 50.0) and (r, ) = (5.00 m, 50.0),... Problem 44P: Given points (r1, 1) and (r2, 2) in polar coordinates, obtain a general formula for the distance... Problem 45P: For the triangle shown in Figure P1.45, what are (a) the length of the unknown side, (b) the tangent... Problem 46P: A ladder 9.00 m long leans against the side of a building. If the ladder is inclined at an angle of... Problem 47P: A high fountain of water is located at the center of a circular pool as shown in Figure P1.47. Not... Problem 48P: A right triangle has a hypotenuse of length 3.00 m, and one of its angles is 30.0. What are the... Problem 49P: In Figure P1.49, find (a) the side opposite , (b) the side adjacent to . (c) cos , (d) sin , and (c)... Problem 50P: In a certain right triangle, the two sides that are perpendicular to each other are 5.00 m and 7.00... Problem 51P: In Problem 50, what is the tangent of the angle for which 5.00 in is the opposite side? Problem 52P: A woman measures the angle of elevation of a mountaintop as 12.0. After walking 1.00 km closer to... Problem 53P: A surveyor measures the distance across a straight river by the following method: starting directly... Problem 54P: Vector A has a magnitude of 8.00 units and makes an angle of 45.0 with the positive x-axis. Vector B... Problem 55P: Vector A has a magnitude of 29 units and points in the positive y-direction. When vector B is added... Problem 56P: An airplane flies 2.00 102 km due west from city A to city B and then 3.00 102 km in the direction... Problem 57P: Vector A is 3.00 units in length and points along the positive x-axis. Vector B is 4.00 units in... Problem 58P: A force F1, of magnitude 6.00 units acts on an object at the origin in a direction = 30.0 above the... Problem 59P: A roller coaster moves 2.00 102 ft horizontally and then rises 135 ft at an angle of 30.0 above the... Problem 60P: Calculate (a) the x-component and (b) the y-component of the vector with magnitude 24.0 m and... Problem 61P: A vector A has components Ax = 5.00 m and Ay = 9.00 m. Find (a) the magnitude and (b) the direction... Problem 62P: A person walks 25.0 north of east for 3.10 km. How far due north and how far due east would she have... Problem 63P: The magnitude of vector A is 35.0 units and points in the direction 325 counterclockwise from the... Problem 64P: A figure skater glides along a circular path of radius 5.00 m. If she coasts around one half of the... Problem 65P: A girl delivering newspapers covers her route by traveling 3.00 blocks west, 4.00 blocks north, and... Problem 66P: A quarterback takes the ball from the line of scrimmage, runs backwards for 10.0 yards, and then... Problem 67P: A vector has an x-component of 25.0 units and a y-component of 40.0 units. Find the magnitude and... Problem 68P: A map suggests that Atlanta is 730. miles in a direction 5.00 north of east from Dallas. The same... Problem 69P: The eye of a hurricane passes over Grand Bahama Island in a direction 60.0 north of west with a... Problem 70P: The helicopter view in Figure P1.70 shows two people pulling on a stubborn mule. Find (a) the single... Problem 71P: A commuter airplane starts from an airport and takes the route shown in Figure P1.71. The plane... Problem 72AP: (a) Find a conversion factor to convert from miles per hour to kilometers per hour. (b) For a while,... Problem 73AP: The displacement of an object moving under uniform acceleration is some function of time and the... Problem 74AP: Assume it takes 7.00 minutes to fill a 30.0-gal gasoline tank. (a) Calculate the rate at which the... Problem 75AP: One gallon of paint (volume = 3.79 103 m3) covers an area of 25.0 m2. What is the thickness of the... Problem 76AP: A sphere of radius r has surface area A = 4r2 and volume V = (4/3)r3. If the radius of sphere 2 is... Problem 77AP: Assume there are 100 million passenger can in the United States and that the average fuel... Problem 78AP Problem 80AP: An average person sneezes about three times per day. Estimate the worldwide number of sneezes... Problem 81AP: The nearest neutron star (a collated star made primarily of neutrons) is about 3.00 1018 m away... format_list_bulleted

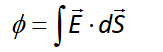
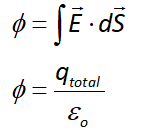

 College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning University Physics (14th Edition)PhysicsISBN:9780133969290Author:Hugh D. Young, Roger A. FreedmanPublisher:PEARSON
University Physics (14th Edition)PhysicsISBN:9780133969290Author:Hugh D. Young, Roger A. FreedmanPublisher:PEARSON Introduction To Quantum MechanicsPhysicsISBN:9781107189638Author:Griffiths, David J., Schroeter, Darrell F.Publisher:Cambridge University Press
Introduction To Quantum MechanicsPhysicsISBN:9781107189638Author:Griffiths, David J., Schroeter, Darrell F.Publisher:Cambridge University Press Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Lecture- Tutorials for Introductory AstronomyPhysicsISBN:9780321820464Author:Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina BrissendenPublisher:Addison-Wesley
Lecture- Tutorials for Introductory AstronomyPhysicsISBN:9780321820464Author:Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina BrissendenPublisher:Addison-Wesley College Physics: A Strategic Approach (4th Editio...PhysicsISBN:9780134609034Author:Randall D. Knight (Professor Emeritus), Brian Jones, Stuart FieldPublisher:PEARSON
College Physics: A Strategic Approach (4th Editio...PhysicsISBN:9780134609034Author:Randall D. Knight (Professor Emeritus), Brian Jones, Stuart FieldPublisher:PEARSON




