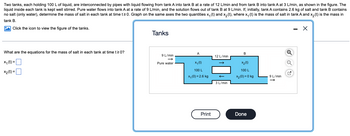
Two tanks, each holding 100 L of liquid, are interconnected by pipes with liquid flowing from tank A into tank B at a rate of 12 L/min and from tank B into tank A at 3 L/min, as shown in the figure. The liquid inside each tank is kept well stirred. Pure water flows into tank A at a rate of 9 L/min, and the solution flows out of tank B at 9 L/min. If, initially, tank A contains 2.6 kg of salt and tank B contains no salt (only water), determine the mass of salt in each tank at time t≥ 0. Graph on the same axes the two quantities x₁ (t) and x₂ (t), where x₁ (t) is the mass of salt in tank A and x₂(t) is the mass in tank B. Click the icon to view the figure of the tanks. X What are the equations for the mass of salt in each tank at time t≥ 0? X₁ (t)= X₂ (t) = Tanks 9 L/min Pure water A x₁ (1) 100 L x₁ (0)=2.6 kg Print 12 L/min 3 L/min B x₂ (1) 100 L x₂(0)=0 kg Done 9 L/min Q Q

Consider three connected lakes, A, B, and C, where lake B has a volume of 2650 km, and water flows from lake A into lake B, and from lake B into lake C, both inflows and outflows at the same rate of 525 km2/year. Suppose that at time t=0 (years), the pollutant concentration of lake B, caused by past industrial pollution that has now been ordered to cease, is five times that of lake A. If the outflow from lake B to lake C is perfectly mixed lake water, how long will it take to reduce the pollution concentration in lake B to twice that of lake A? (Choose all correct answers) OA More than five years. OB Less than eight years. OC Less than six years. D D. More than 11 years.

Consider the mixing process shown in the figure. A mixing chamber initially contains 4 liters of a clear liquid. Clear liquid flows into the chamber at a rate of 10 liters per minute. A dye solution having a concentration of 0.6 kilograms per liter is injected into the mixing chamber at a constant rate of r liters per minute. When the mixing process is started, the well-stirred mixture is pumped from the chamber at a rate of 10 + r liters per minute. (a) Develop a mathematical model for the mixing process. Let Q represent the amount of dye in kilograms in the mixture. dQ kg/min dt (b) The objective is to obtain a dye concentration in the outflow mixture of 0.5 kilograms per liter. What injection rate r is required to achieve this equilibrium solution? 7 = L/min Would this equilibrium value of r be different if the fluid in the chamber at time t = 0 contained some dye? no (c) Assume the mixing chamber contains 4 liters of clear liquid at time t = 0. How many minutes will it take for the…

Two connected tanks, each with a capacity of 50 liters, contain brine (saltwater). Initially, the first tank contains 18 liters of brine with a salt concentration of 3 grams per liter and the second contains 17 liters of brine with a salt concentration of 2 grams per liter. At t = 0 brine with a salt concentration of 6 grams per liter flows into the first tank at 8 liters per hour. Well-stirred brine flows from the first tank into the second at 7 liters per hour, from the second into the first at 5 liters per hour, from the first into a drain at 4 liters per hour, and from the second into a drain at 3 liters per hour.   (a)  Determine the volume (liters) of brine in each tank as a function of time.(b) Give an initial-value problem that governs the amount (grams) of salt in eachtank as a function of time.(c) Give the interval of definition for the solution of this initial-value problem.

The brine solution is recycled using a series of mixing tanks before being released outside of the tanks. Tank A receives pure water at a rate of 4 L/min. The solution is pumped to Tanks B and C at rates of 2 L/min and x L/min, respectively, from Tank A, which originally contains 500 L of brine solution in which 200 g of salt is first dissolved. At a rate of 4 L/min, Tank B from its original 400 L of brine solution in which 1000 g of salt has been dissolved pumps the solution to Tank C. At a rate of y L/min, Tank C, which originally holds 400 L of pure water, pumps the solution back to Tank B. Meanwhile, Tank C releases z L/min of solution out of the system. a. Determine the values of x, y, and z if the volume of the solution within each tank stays constant throughout time. b. Find out the brine solution's concentration coming out of each tank at time t-8 minutes. 2 L/min TANK B TANKA 4 L/min y L/min Pure water @4 L/min x L/min TANK C z L/min

Consider three connected lakes, A, B, and C, where lake B has a volume of 2650 km3, and water flows from lake A into lake B, and from lake B into lake C, both inflows and outflows at the same rate of 525 km3/year. Suppose that at time t=0 (years), the pollutant concentration of lake B, caused by past industrial pollution that has now been ordered to cease, is five times that of lake A. If the outflow from lake B to lake C is perfectly mixed lake water, how long will it take to reduce the pollution concentration in lake B to twice that of lake A? Choose all correct answers. B Approximately 6.3 years. Approximately 4.4 years. Approximately 5 years. Approximately 8.3 years. Approximately 7 years.

My cat Pythagoras has purchased and installed in my back yard a large barrel with a 400-gallon capacity. It is initially half full of clean water. But he has three hoses feeding this barrel, each with a rate of flow of 2 gal./min. of water. Two provide the barrel with clean water, but the third contains a concentration of 2 g/gal. of liquid calculus text books. My other cat, Jolie, has decided to sabotage this process by puncturing a hole toward the bottom of this barrel that allows 4 gal./min. to leak. If we assume that there is a benevolent feline deity that blissfully stirs this barrel with a rather large spoon, then write a differential equation that mathematically models the rate at which the amount of liquid calculus text books changes in the barrel (at least up to the time it fills). Thereafter, solve this differential equation.

A pasta company with a blue box produces 1,400 tons of semolina pasta per day. The pasta is fed into a machine as a large, loose dough and then squeezed through a bronze die to form a desired shape. As the pasta is squeezed through the die, the volume of space it takes up decreases while the mass remains constant, and thus the resulting shape is more dense. If m is the constant mass of the pasta in grams and V is the volume of the pasta in mL, then the density p is given by p= - m (a) If the mass remains constant while the volume changes with time, find dp dt (b) Suppose the mass of the pasta is 80g, the density is 0.8 g/mL, and the volume is decreasing at a rate of 400 mL/sec. How fast is the density of the pasta changing at that moment? mL · sec

Let T : R2 → R² be the linear transformation which rotates vectors counter-clockwise about the origin by 7T/3 radians (60 degrees). (i) Find the standard matrix of the linear transformation T. (ii) Use your matrix to rotate the vector v = counterclockwise about the origin by 4 T/3 radians.

Consider the two tank apparatus shown in the figure. Each tank has capacity 500 liters and initially contains 50 liters of fresh water. At time t = 0, the well-stirred mixing process begins. Suppose that the concentration of brine flowing into Tank 1 via the top tube is 0.75 kilograms per liter, and that the flow rates are r1 = r3 = 5 liters per minute, and r2 = r4 = 12 liters per minute. (a.) Determine the volume of solution in each tank as a function of time, t, in minutes. V1(t) = ? V2(t) = ? (b) Determine the time interval of interest (The process when a tank is full or empty) Stopping time is ? minutes (c) Let Q1(t) and Q2(t) denote the amount of salt (in kilograms) in the tanks at time t (in minutes). Derive the initial value problem with Q1(t) and Q2(t) as dependent variables describing the mixing process. Enter Q1(t) as Q1(t) and Q2(t) as Q2(t) (d/dt) (Q1(t)) = ...................................................... kg/min (d/dt) (Q2(t)) =…