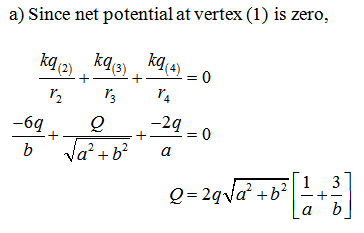
Two point loads of the value (-2Q) and (-6q) are arranged at two opposite vertices of a
rectangle of sides a and B (A<B), as seen in the figure. Calculated:
a) the value of the charge Q that we have to place on the vertex (3) so that the potential
vertex (1) be zero.
Considering from now on the three charges, calculate
(1)
- 6q (2) b) the electric field at Point O (center of the
-2q (4)
fo
(3)
rectangle) due to the three loads.
(C) the electrical potential in Point 0 and in the
P-point (side mid-point).
d) the work we must do to bring
another point load of Qo value from point P
Point O.
Note
Accurately define all the physical quantities used.
In mathematical developments clearly justify the steps.

Hi due to the complexity of the question and time limit, the first two sub parts have been answered.
Step by stepSolved in 2 steps with 2 images

- Please answer (a), (b), and (c).arrow_forwardOnly 2 c)arrow_forward(1) A small droplet with a mass of 0.2 g is placed in between the plates. In order to make the droplet float at its original position, how much charge do we need to put on the droplet? Is the charge positive or negative? (2) You are given three types of dielectric materials to fill up the space between the plates (highlighted in light blue). Each material has to take up at least 20% of the total volume of the space. The table below provides the dielectric constant of the materials. Suppose the materials can form any shape. (i) Find a way to fill up the space such that the capacitance of the capacitor is smaller than 24 × 10-10 F. (ii) Find a way to fill up the space such that the capacitance of the capacitor is larger than 52 x 10-10 F. (3) For 2(ii), suppose that the materials are filled sequentially in the order of A–B C and that before filling the space is filled with air. (The dielectric constant of air is 1.) Calculate the electric potential energy stored by the capacitor at each…arrow_forward
- A non-conducting sphere of radius R = 5 cm has its center at the origin O of coordinate system as shown in Fig. Its has two spherical cavities of radius r = 1 cm, whose centers are at (0,3 cm), (0.-3 cm), respectively, and solid material of the sphere has uniform positive charge density p = 1/π µСm-³. Calculate electric potential at point P (4 cm, 0). y Fig. Parrow_forwardSome practice with an infinite line charge. (a) What is the electric field on an infinite line charge that has a constant charger per unit length of X? (b) What is the electrostatic potential in this case? Is it possible to make the potential vanish at infinity? (c) Consider an infinite conducting cylinder of radius a that is grounded. Suppose there is an infinite line charge with a constant charge per unity length that is located inside the cylinder, such that it is parallel to the cylinder, but displaced from the central axis by an amount b, with b < a. Determine the electrostatic potential everywhere inside the cylinder. I suggest using the method of images, by placing another line charge outside the cylinder.arrow_forward