
Elements Of Electromagnetics
7th Edition
ISBN: 9780190698614
Author: Sadiku, Matthew N. O.
Publisher: Oxford University Press
expand_more
expand_more
format_list_bulleted
Question
thumb_up100%
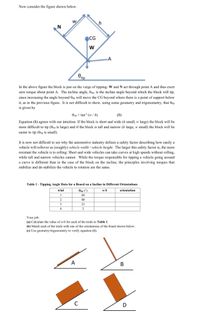
Transcribed Image Text:Now consider the figure shown below.
N
CG
-A
tip
In the above figure the block is just on the verge of tipping: W and N act through point A and thus exert
zero torque about point A. The incline angle, Otip, is the incline angle beyond which the block will tip,
since increasing the angle beyond Otip will move the CG beyond where there is a point of support below
it, as in the previous figure. It is not difficult to show, using some geometry and trigonometry, that Otip
is given by
Orip = tan' (w / h)
(1)
Equation (1) agrees with our intuition: If the block is short and wide (h small, w large) the block will be
more difficult to tip (Otip is large) and if the block is tall and narrow (h large, w small) the block will be
easier to tip (0tip is small).
It is now not difficult to see why the automotive industry defines a safety factor describing how easily a
vehicle will rollover as (roughly) vehicle width / vehicle height. The larger this safety factor is, the more
resistant the vehicle is to rolling: Short and wide vehicles can take curves at high speeds without rolling,
while tall and narrow vehicles cannot. While the torque responsible for tipping a vehicle going around
a curve is different than in the case of the block on the incline, the principles involving torques that
stabilize and de-stabilize the vehicle to rotation are the same.
Table 1 - Tipping Angle Data for a Board on a Incline in Different Orientations
trial
Otip (°)
w/h
orientation
1
69
2
88
3
21
4
Your job:
(a) Calculate the value of w/h for each of the trials in Table 1.
(b) Match each of the trials with one of the orientations of the board shown below.
(c) Use geometry/trigonometry to verify equation (1).
A
В
D
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 2 steps with 2 images

Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- Please all choose with explainarrow_forwardQ6: A right triangle whose hypotenuse is v3 m long is revolved about one of its legs to generate a right circular cone. Find the radius, height, and volume of the cone of greatest volume that can be made this way? V3 rarrow_forwardMirror the triangle shown below along a 45° - axis. ) (1,3) P₂ D P₁ 45° (1, 1)! (3, 3)arrow_forward
- Learning Goal: To understand the concept of moment of a force and how to calculate it using a vector formulation. A man wishes to spin a merry-go-round in the x-y plane centered at the origin (0.000, 0.000, 0.000). (Figure 1) Because he must pull upward and cannot stand on the merry-go-round, he must apply a force in the direction of the unit vector u = (-1/3)i+(2/3)j+(2/3) k. He pulls with a force F = 47.0 lb from point A[(3.00, 4.00, 0.000) ft]. Part A What are the x, y, and z components of the resulting moment acting on the merry-go-round about the origin? Express your answers numerically in pound-feet to three significant figures separated by commas. ► View Available Hint(s) VE ΑΣΦ Mz, My, M₂ = Submit Part B Complete previous part(s) Provide Feedback vec ? lb-ftarrow_forwardA vector B is 10 cm. long and has a direction – sense of 45°. Vector C is 15 cm. long and has a direction sense of 150°, Find the vector sum or resultant magnitude and its direction by; y 1. Mathematical Solution, 2. Graphical Solution. Scale: 1:1 cm. 3. Draw the position of resultant. 1500 e 450arrow_forwardFind the magnatidue of VB(ft/s): Find the angle of VB(degrees)arrow_forward
- show all steps in detail, units and formulas please.arrow_forwardshow all steps in detail, units and formulas please.arrow_forwardMany aspects of a gymnast's motion can be modeled by representing the gymnast by four segments consisting of arms, torso (including the head), thighs, and lower legs, as in the figure below. Thigh O Arm Leg 60° 60° Torso a b In the figure, (b) shows arrows of lengths r, locating the center of gravity of each segment. Use the data below and the cg coordinate system shown in figure (b) to locate the center of gravity of the gymnast shown in figure (a). Masses for the arms, thighs, and legs include both appendages. (Enter your answers in m, to at least three significant figures.) Segment Mass (kg) Length (m) (m) Arms 6.89 0.548 0.242 Torso 33.6 0.613 0.337 Thighs 14.1 0.376 0.145 Legs 7.50 0.350 0.227 HINT m %D m y cg E Earrow_forward
- See image that is attached. Thank you.arrow_forwardWhat is the equation of the circle that has radius of 9 and centre at 3 8)2 O (x+3)°+(y-8)°-9 O (x-3)²+(y+8)² – 81 O (x-3)° +(y18)²-9 O (x+3)² + (y-8)²=81 A Moving to another question will save this response.arrow_forwardPlease do this carefully.arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
 Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press
Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON
Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education
Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY
Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY
Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY

Elements Of Electromagnetics
Mechanical Engineering
ISBN:9780190698614
Author:Sadiku, Matthew N. O.
Publisher:Oxford University Press

Mechanics of Materials (10th Edition)
Mechanical Engineering
ISBN:9780134319650
Author:Russell C. Hibbeler
Publisher:PEARSON

Thermodynamics: An Engineering Approach
Mechanical Engineering
ISBN:9781259822674
Author:Yunus A. Cengel Dr., Michael A. Boles
Publisher:McGraw-Hill Education

Control Systems Engineering
Mechanical Engineering
ISBN:9781118170519
Author:Norman S. Nise
Publisher:WILEY

Mechanics of Materials (MindTap Course List)
Mechanical Engineering
ISBN:9781337093347
Author:Barry J. Goodno, James M. Gere
Publisher:Cengage Learning

Engineering Mechanics: Statics
Mechanical Engineering
ISBN:9781118807330
Author:James L. Meriam, L. G. Kraige, J. N. Bolton
Publisher:WILEY