MATLAB: An Introduction with Applications
6th Edition
ISBN: 9781119256830
Author: Amos Gilat
Publisher: John Wiley & Sons Inc
expand_more
expand_more
format_list_bulleted
Related questions
Concept explainers
Question
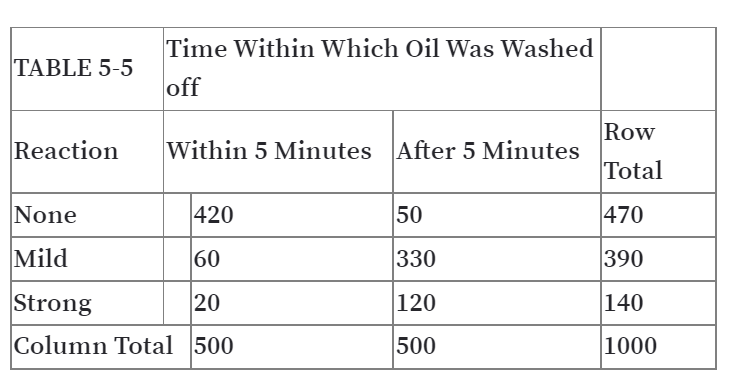
Survey: Reaction to Poison Ivy Allergic reactions to poison ivy can be miserable. Plant oils cause the reaction. Researchers at Allergy Institute did a study to determine the effects of washing the oil off within 5 minutes of exposure. A random sample of 1000 people with known allergies to poison ivy participated in the study. Oil from the poison ivy plant was rubbed on a patch of skin. For 500 of the subjects, it was washed off within 5 minutes. For the other 500 subjects, the oil was washed off after 5 minutes. The results are summarized in Table 5-5.
Let’s use the following notation for the various
M = mild reaction. S = strong reaction. Find the following
(a) P (N) , P (M) , P (S)
(b) P(N| W), P(S | W)
(c) P (N | A) , P (S |A)
(d) P(N and W), P (M and W)
(e) . Are the events N = no reaction and M = mild reaction mutually exclusive? Explain.
(f) Are the events N = no reaction and W = washing oil off within 5 minutes independent? Explain.
Transcribed Image Text:Time Within Which Oil Was Washed
TABLE 5-5
off
Row
Reaction
Within 5 Minutes After 5 Minutes
Total
None
420
50
470
Mild
|60
330
390
Strong
20
120
140
Column Total 500
500
1000
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 4 steps
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, statistics and related others by exploring similar questions and additional content below.Similar questions
- Allergic reactions to poison ivy can be miserable. Plant oils cause the reaction. Researchers at Allergy Institute did a study to determine the effects of washing the oil off within 5 minutes of exposure. A random sample of 1000 people with known allergies to poison ivy participated in the study. Oil from the poison ivy plant was rubbed on a patch of skin. For 500 of the subjects, it was washed off within 5 minutes. For the other 500 subjects, the oil was washed off after 5 minutes. The results are summarized in the following table. Reaction Within 5 Minutes After 5 Minutes Row Total NoneMildStrong 4025543 64323113 466378156 Column Total 500 500 1000 Let's use the following notation for the various events: W = washing oil off within 5 minutes, A = washing oil off after 5 minutes, N = no reaction, M = mild reaction, S = strong reaction. Find the following probabilities for a person selected at random from this sample of 1000 subjects. (Use 3 decimal places.) (d) P(N and…arrow_forwardBighorn sheep are beautiful wild animals found throughout the western United States. Let x be the age of a bighorn sheep (in years), and let y be the mortality rate (percent that die) for this age group. For example, x = 1, y = 14 means that 14% of the bighorn sheep between 1 and 2 years old died. A random sample of Arizona bighorn sheep gave the following information: x 1 2 3 4 5 y 15.8 17.3 14.4 19.6 20.0 Σx = 15; Σy = 87.1; Σx2 = 55; Σy2 = 1,540.45; Σxy = 272 (a) Find x, y, b, and the equation of the least-squares line. (Round your answers for x and y to two decimal places. Round your least-squares estimates to three decimal places.) x = y = b = ŷ = + x (b) Draw a scatter diagram for the data. Plot the least-squares line on your scatter diagram. (c) Find the sample correlation coefficient r and the coefficient of determination r2. (Round your answers to three decimal places.) r = r2 = What percentage of variation in y is…arrow_forwardA psychologist would like to examine the effects of a new drug on the activity level of animals. Three samples of rats are selected with n=5 in each sample. One group gets no drug, one group gets s small dose, and the third group gets a large dose. The psychologist records the activity level for each animal. The data from this experiment are presented below. No drug Small dose Large dose 5, 3 , 1 , 1 , 5 5, 5, 9, 6, 5 10, 12, 9, 6, 8 =3, SS=16 =6, SS=12 =9, SS=20 Do these data indicate any significant differences among the three groups? Test with α=0.05. Compute the effect size and interpret the result.arrow_forward
- Green Tea and Prostate Cancer A preliminary study suggests a benefit from green tea for those at risk of prostate cancer. The study involved 60 men with PIN lesions, some of which turn into prostate cancer. Half the men, randomly determined, were given 600 mg a day of a green tea extract while the other half were given a placebo. The study was double-blind, and the results after one year are shown in the table below. Treatment Cancer No Cancer Green Tea 1 29 Placebo 9 21 (a) Test at a 5% significant level to see if the sample provide evidence that taking green tea extract reduces the risk of developing prostate cancer. (b) Find 98% confidence interval for the difference between two population proportions of men with PIN lesions who get prostate cancer after taking green tea extract and after taking placebo for a year. Interpret the C.I. result.arrow_forwardBighorn sheep are beautiful wild animals found throughout the western United States. Let x be the age of a bighorn sheep (in years), and let y be the mortality rate (percent that die) for this age group. For example, x = 1, y = 14 means that 14% of the bighorn sheep between 1 and 2 years old died. A random sample of Arizona bighorn sheep gave the following information: x 1 2 3 4 5 y 13.8 19.3 14.4 19.6 20.0 Σx = 15; Σy = 87.1; Σx2 = 55; Σy2 = 1554.45; Σxy = 274b) Find the equation of the least-squares line. (Round your answers to two decimal places.) ŷ = + x (c) Find r. Find the coefficient of determination r2. (Round your answers to three decimal places.) r = r2 = d) Test the claim that the population correlation coefficient is positive at the 1% level of significance. (Round your test statistic to three decimal places.) t =arrow_forwardCollege students and STDs: A recent report estimated that 25% of all college students in the United States have a sexually transmitted disease (STD). Due to the demographics of the community, the director of the campus health center believes that the proportion of students who have a STD is higher at his college. He tests H0: p = 0.25 versus Ha: p > 0.25. The campus health center staff select a random sample of 150 students and determine that 43 have been diagnosed with a STD. Conduct a hypothesis test to address the director’s hypothesis. Use a 5% significance level to make your decision. Use the applet to determine the P‑value. Click here to open the applet. Which of the following is an appropriate conclusion based on the results? The data provides strong evidence that the proportion of students at his college who have a STD is more than 25%. The data suggests that the proportion of students at his college who have a STD is 29%. Of the students surveyed at his college, 29%…arrow_forward
- NEW STUDY: A researcher is interested in the relationship between levels of stress at work and self-reports of overall health. Stress at work is measured on a scale from 0 (no stress at work) to 100 (extreme levels of stress at work). The researcher conducts a study with 11 subjects and determines that the relationship between self-reports of stress and self-reports of overall heath is r = -.41. Which test was conducted for this study? Group of answer choices z-test t-test correlation chi-squarearrow_forwardBighorn sheep are beautiful wild animals found throughout the western United States. Let x be the age of a bighorn sheep (in years), and let y be the mortality rate (percent that die) for this age group. For example, x = 1, y = 14 means that 14% of the bighorn sheep between 1 and 2 years old died. A random sample of Arizona bighorn sheep gave the following information: x 1 2 3 4 5 y 15.8 17.3 14.4 19.6 20.0 Σx = 15; Σy = 87.1; Σx2 = 55; Σy2 = 1,540.45; Σxy = 272 (a) Find x, y, b, and the equation of the least-squares line. (Round your answers for x and y to two decimal places. Round your least-squares estimates to three decimal places.) x = y = b = ŷ = + xarrow_forwardPrices of diamonds are very sensitive to their weight (measured in carat). For example, the price of a 1 carat diamond tends to be much higher than the price of a 0.99 carats diamond. To study this phenomenon in more detail, we consider two random samples of diamonds, 0.99 carats and 1 carat, each sample of size 23. The average price of 0.99 carats diamonds in the sample is $4451, while the average price of 1 carat diamonds in the sample is $5681. From long-term studies, we know that both the prices of 0.99 carats diamonds and the prices of 1 carat diamonds are normally distributed with standard deviations oj = $1332 (0.99 carats) and o2 = $1613 (1 carat), respectively. (a) Carry out a hypothesis test at significance level 0.01 to evaluate whether the true average price µi of 0.99 carats diamonds is lower than the true average price µ2 of 1 carat diamonds. Specify Ho and Ha, the test statistic, and calculate the p-value or use p-value considerations to conclude. (b) Compute the type II…arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
- MATLAB: An Introduction with ApplicationsStatisticsISBN:9781119256830Author:Amos GilatPublisher:John Wiley & Sons Inc
Probability and Statistics for Engineering and th...StatisticsISBN:9781305251809Author:Jay L. DevorePublisher:Cengage Learning
Statistics for The Behavioral Sciences (MindTap C...StatisticsISBN:9781305504912Author:Frederick J Gravetter, Larry B. WallnauPublisher:Cengage Learning 
Elementary Statistics: Picturing the World (7th E...StatisticsISBN:9780134683416Author:Ron Larson, Betsy FarberPublisher:PEARSON
The Basic Practice of StatisticsStatisticsISBN:9781319042578Author:David S. Moore, William I. Notz, Michael A. FlignerPublisher:W. H. Freeman
Introduction to the Practice of StatisticsStatisticsISBN:9781319013387Author:David S. Moore, George P. McCabe, Bruce A. CraigPublisher:W. H. Freeman
MATLAB: An Introduction with Applications
Statistics
ISBN:9781119256830
Author:Amos Gilat
Publisher:John Wiley & Sons Inc
Probability and Statistics for Engineering and th...
Statistics
ISBN:9781305251809
Author:Jay L. Devore
Publisher:Cengage Learning
Statistics for The Behavioral Sciences (MindTap C...
Statistics
ISBN:9781305504912
Author:Frederick J Gravetter, Larry B. Wallnau
Publisher:Cengage Learning
Elementary Statistics: Picturing the World (7th E...
Statistics
ISBN:9780134683416
Author:Ron Larson, Betsy Farber
Publisher:PEARSON
The Basic Practice of Statistics
Statistics
ISBN:9781319042578
Author:David S. Moore, William I. Notz, Michael A. Fligner
Publisher:W. H. Freeman
Introduction to the Practice of Statistics
Statistics
ISBN:9781319013387
Author:David S. Moore, George P. McCabe, Bruce A. Craig
Publisher:W. H. Freeman