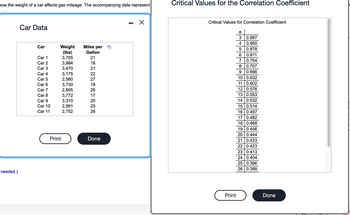
An engineer wanted to determine how the weight of a car affects gas mileage. The accompanying data represent the weights of various domestic cars and their gas mileages in the city for a certain model year. Complete parts (a) through (d) below. Click here to view the car data. Click here to view the table of critical values of the correlation coefficient (a) Determine which variable is the likely explanatory variable and which is the likely response variable. Choose the correct answer below. The explanatory variable is the weight and the response variable is the miles per gallon. O The explanatory variable is the miles per gallon and the response variable is the weight. (b) Draw a scatter diagram of the data. Choose the correct graph below. OA. 4100- 3300-1 2500- 15 23 Miles per Gallon Q B. r= (Round to three decimal places as needed.) 3. 154 ****** 2500 3300 4100 Weight (lbs) (c) Compute the linear correlation coefficient between the weight of a car and its miles per gallon. Q O C. 4100- 3300 2500- 15 23 31 Miles per Gallon Q G O D. 15-1 ****** > 2500 3300 4100 Weight (lbs) Q G

A survey was taken in 2018 that asked people about their saving habits. Researchers wanted to know if people who saved more also spent less. The scatterplot below shows their results when comparing two variables: the amount people reported that they put into savings each month, and the amount they reported that they spent on clothes. The researchers found the correlation coefficient for this data to be -0.239. Which of the following is true about these variables? a. There is no relationship between savings and money spent on clothes each month.b. There is a weak, positive linear relationship between savings and money spent on clothes each month.c. There is a perfect, negative linear relationship between savings and money spent on clothes each month.d. There is a weak, negative linear relationship between savings and money spent on clothes each month.

Susan, a personal trainer, was interested in whether or not there was a linear relationship between the number of visits her clients made to the gym each week and the average amount of time her clients exercised per visit. She took the following data.   Client 1 2 3 4 5 6 Number of visits per week 1 3 4 2 3 5 Average time spent exercising per visit (hours) 2 1.5 1 2 2 0.30   Is the correlation coefficient significant? Group of answer choices No Yes Perhaps Insufficient Information to make a decision

The price drivers pay for gasoline often varies a great deal across regions throughout the United States. The following data show the price per gallon for regular gasoline for a random sample of gasoline service stations for three major brands of gasoline (Shell, BP, and Marathon) located in eleven metropolitan areas across the upper Midwest region (OhioGasPrices.com website, March 18, 2012). Click on the datafile logo to reference the data. DATA file Shell BP Metropolitan Area Marathon Akron, Ohio Cincinnati, Ohio Cleveland, Ohio Columbus, Ohio Ft. Wayne, Indiana Indianapolis, Indiana Lansing, Michigan Lexington, Kentucky Louisville, Kentucky Muncie, Indiana Toledo, Ohio 3.77 3.72 3.87 3.76 3.78 3.87 3.89 3.79 3.83 3.83 3.85 3.77 3.83 3.85 3.93 3.84 3.84 4.04 3.87 3.87 3.99 3.79 3.78 3.81 3.69 3.78 3.84 3.84 3.83 3.79 3.79 3.86 3.86 Use a = .05 to test for any significant difference in the mean price of gasoline for the three brands. Round SS to 6 decimals, MS to 6 decimals, F to 2…

A researcher measures GPA and height for a group of high school students. What kind of correlation is likely to be obtained for these two variables?​

A random sample of college students was surveyed about how they spend their time each week.  The scatterplot below displays the relationship between the number of hours each student typically works per week at a part- or full-time job and the number of hours of television each student typically watches per week.  The correlation between these variables is r = –0.63, and the equation we would use to predict hours spent watching TV based on hours spent working is as follows:             Predicted hours spent watching TV = 17.21 – 0.23(hours spent working) Since we are using hours spent working to help us predict hours spent watching TV, we’d call hours spent working a(n) __________________ variable and hours spent watching TV a(n) __________________ variable. The correlation coefficient, along with what we see in the scatterplot, tells us that the relationship between the variables has a direction that is _________________ and a strength that is ______________________. According to the…

For the following research topics, what is the independent variable and what is the dependent variable? Examining the effect of IQ on Grades Gender and Self-Esteem Age and Political Views Race and level of education

Fully explain the issues and problems with the below correlational data. Focus on the data in the highlighted box versus the rest of the data. Do not try to explain the data, but rather focus on the statistical issues and problems. IF YOU CAN'T SEE THE IMAGE HERE. PLEASE GO TO THIS LINK Linke 10 6. 8. 7. 5. 4. 10 20 30 40 50 60 70 80 90 100 Age Enjoyment of Hip-Hop

For a data set of chest sizes (distance around chest in inches) and weights (pounds) of twelve anesthetized bears that were measured, the linear correlation coefficient is r= 0.276. Use the table available below to find the critical values of r. Based on a comparison of the linear correlation coefficient r and the critical values, what do you conclude about a linear correlation? Click the icon to view the table of critical values of r. The critical values are Table of Critical Values of r IT Number of Pairs of Data n Critical Value of r 4 0.950 0.878 0.811 7 0.754 0.707 0.666 10 0.632 11 0.602 12 0.576

Select the most appropriate response. If the correlation between a person’s age and annual income is 0.60, then the coefficient of determination tells us that: 36% of the variation in a person’s annual income can be explained by the predictor variable age. 36% of a person’s annual income can be explained by their age 60% of the variation in a person’s annual income can be explained by the predictor variable age 60% of a person’s annual income can be explained by their age