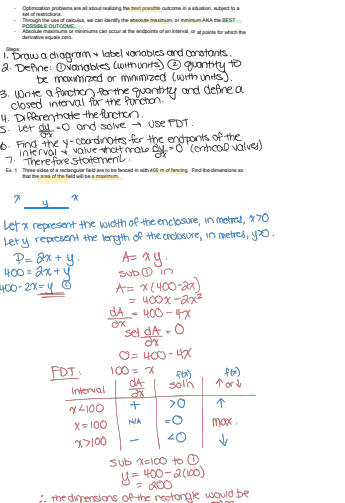
Optimization problem are all about realizing the best possible outcome in a situation, subject to a identify the absolute maximum or minimumAKAST SELE OUTCO derivative equ can can occur at the endpoints of an interval, or at points for which 1. Draw a diagram label variables and constants. 2. Define: Ovanables (with units) quantity to be maximized or minimized (with units). 3. Write a fonction for the quantity and define a closed interval for the function. 4. Differentiate the function. 3. Let dy - and solve Use PDT. 6. Find the y-coordinates for the endpoints of the interval value that make - Contical valves) 7. Therefore statemenil. Ex. 1 Three sides of a rectangular field fenced in with 400 m offencing. Find the dimensions x x Let x represent the width of the enclosure, in metres, x70 Lety represent the length of the enclosure, in metres, yoo. P=2x+y₁ 400=2x+y 400-2x=4 A= xy. Subin A= x(400-2x) = 400x-2x² dA όχι 400-47 set dA-O όχ 0=400-4 FDT 100 = x fox) fox) αA Interval Solh Ford ax 14100 + > x=100 N/A max. 07100 - <0 Sub x=100 to ①. y=400-2(100) = 00 the dimensions of the rectangle would be The tensile strength of a new plastic at temperature T (°C) is given by the relationship S = ln(T+500) - 4T + 2100, where S represents the tensile strength measured in megapascals (MPa). Determine the temperature at which the tensile strength is a maximum.

(This is a calculus optimization problem, please show all work and add the chart for the first derivative test just like this example I am giving here, do the work like the example and put that chart too)