
Introductory Circuit Analysis (13th Edition)
13th Edition
ISBN: 9780133923605
Author: Robert L. Boylestad
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Question
This question is split into parts from A to E please help with all parts since it's still one question
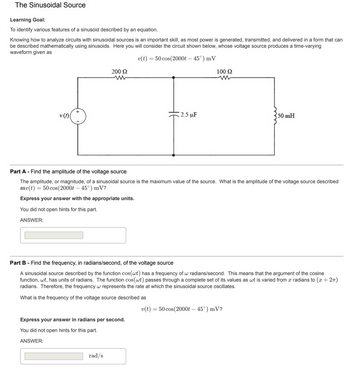
Transcribed Image Text:The Sinusoidal Source
Learning Goal:
To identify various features of a sinusoid described by an equation.
Knowing how to analyze circuits with sinusoidal sources is an important skill, as most power is generated, transmitted, and delivered in a form that can
be described mathematically using sinusoids. Here you will consider the circuit shown below, whose voltage source produces a time-varying
waveform given as
v(t) = 50 cos(2000t - 45°) mV
v (t)
200 S2
2.5 μF
Express your answer in radians per second.
You did not open hints for this part.
ANSWER:
rad/s
100 S2
www
Part A - Find the amplitude of the voltage source
The amplitude, or magnitude, of a sinusoidal source is the maximum value of the source. What is the amplitude of the voltage source described
asv(t) = 50 cos (2000t - 45°) mV?
Express your answer with the appropriate units.
You did not open hints for this part.
ANSWER:
Part B - Find the frequency, in radians/second, of the voltage source
A sinusoidal source described by the function cos(wt) has a frequency of w radians/second. This means that the argument of the cosine
function, wt, has units of radians. The function cos(wt) passes through a complete set of its values as wt is varied from a radians to (x + 2π)
radians. Therefore, the frequency w represents the rate at which the sinusoidal source oscillates.
What is the frequency of the voltage source described as
50 mH
v(t) = 50 cos(2000t – 45°) mV?
![Part C - Find the frequency, in hertz, of the voltage source
A sinusoidal source described by the function cos(wt) has a frequency of w radians/second. Using these units is often convenient but it may
[MathJax]/jax/output/HTML-CSS/fonts/TeX/Main/Italic/Latin1 Supplement.js hertz (abbreviated Hz) represents the number of cycles per second of
Loading
oscillation and provides an easy way to picture the sinusoid. We often use the symbol f to represent the frequency in hertz, while we use the
symbol w to represent the frequency in radians/second. The relationship between f and w is given by the following equation:
w = 2π f
What is the frequency of the voltage source described as
Express your answer to two digits after the decimal point in hertz.
You did not open hints for this part.
ANSWER:
Hz
v(t) = 50 cos(2000t - 45°) mV?
Part D - Find the period of the voltage source
The period of a sinusoidal source is the time required for the sinusoid to pass through all of its possible values. We use the symbol T to
represent the period of a sinusoid. The period and the frequency are inversely related. A sinusoidal source described by the function cos(wt)
has a frequency of w radians/second, or a frequency f = w/27 Hz. The units hertz represents the number of cycles per second. Since the
period is the number of seconds per cycle, the period is the inverse of the frequency in hertz:
T=
==
Substituting the frequency in radians/second, w, for the frequency in Hz gives us another way to calculate the period:
2π
T = 21
What is the period of the voltage source described as
v(t) =
= 50 cos(2000t - 45°) mV?
Express your answer to two digits after the decimal point and include the appropriate units.
You did not open hints for this part.
ANSWER:](https://content.bartleby.com/qna-images/question/53eb90d1-796e-4245-a7d6-0942bcbb431d/0e8e91d1-e1a4-4200-a97d-39b33fdf51bc/wuk0b5c_thumbnail.png)
Transcribed Image Text:Part C - Find the frequency, in hertz, of the voltage source
A sinusoidal source described by the function cos(wt) has a frequency of w radians/second. Using these units is often convenient but it may
[MathJax]/jax/output/HTML-CSS/fonts/TeX/Main/Italic/Latin1 Supplement.js hertz (abbreviated Hz) represents the number of cycles per second of
Loading
oscillation and provides an easy way to picture the sinusoid. We often use the symbol f to represent the frequency in hertz, while we use the
symbol w to represent the frequency in radians/second. The relationship between f and w is given by the following equation:
w = 2π f
What is the frequency of the voltage source described as
Express your answer to two digits after the decimal point in hertz.
You did not open hints for this part.
ANSWER:
Hz
v(t) = 50 cos(2000t - 45°) mV?
Part D - Find the period of the voltage source
The period of a sinusoidal source is the time required for the sinusoid to pass through all of its possible values. We use the symbol T to
represent the period of a sinusoid. The period and the frequency are inversely related. A sinusoidal source described by the function cos(wt)
has a frequency of w radians/second, or a frequency f = w/27 Hz. The units hertz represents the number of cycles per second. Since the
period is the number of seconds per cycle, the period is the inverse of the frequency in hertz:
T=
==
Substituting the frequency in radians/second, w, for the frequency in Hz gives us another way to calculate the period:
2π
T = 21
What is the period of the voltage source described as
v(t) =
= 50 cos(2000t - 45°) mV?
Express your answer to two digits after the decimal point and include the appropriate units.
You did not open hints for this part.
ANSWER:
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 2 steps with 1 images

Knowledge Booster
Similar questions
Recommended textbooks for you
 Introductory Circuit Analysis (13th Edition)Electrical EngineeringISBN:9780133923605Author:Robert L. BoylestadPublisher:PEARSON
Introductory Circuit Analysis (13th Edition)Electrical EngineeringISBN:9780133923605Author:Robert L. BoylestadPublisher:PEARSON Delmar's Standard Textbook Of ElectricityElectrical EngineeringISBN:9781337900348Author:Stephen L. HermanPublisher:Cengage Learning
Delmar's Standard Textbook Of ElectricityElectrical EngineeringISBN:9781337900348Author:Stephen L. HermanPublisher:Cengage Learning Programmable Logic ControllersElectrical EngineeringISBN:9780073373843Author:Frank D. PetruzellaPublisher:McGraw-Hill Education
Programmable Logic ControllersElectrical EngineeringISBN:9780073373843Author:Frank D. PetruzellaPublisher:McGraw-Hill Education Fundamentals of Electric CircuitsElectrical EngineeringISBN:9780078028229Author:Charles K Alexander, Matthew SadikuPublisher:McGraw-Hill Education
Fundamentals of Electric CircuitsElectrical EngineeringISBN:9780078028229Author:Charles K Alexander, Matthew SadikuPublisher:McGraw-Hill Education Electric Circuits. (11th Edition)Electrical EngineeringISBN:9780134746968Author:James W. Nilsson, Susan RiedelPublisher:PEARSON
Electric Circuits. (11th Edition)Electrical EngineeringISBN:9780134746968Author:James W. Nilsson, Susan RiedelPublisher:PEARSON Engineering ElectromagneticsElectrical EngineeringISBN:9780078028151Author:Hayt, William H. (william Hart), Jr, BUCK, John A.Publisher:Mcgraw-hill Education,
Engineering ElectromagneticsElectrical EngineeringISBN:9780078028151Author:Hayt, William H. (william Hart), Jr, BUCK, John A.Publisher:Mcgraw-hill Education,

Introductory Circuit Analysis (13th Edition)
Electrical Engineering
ISBN:9780133923605
Author:Robert L. Boylestad
Publisher:PEARSON

Delmar's Standard Textbook Of Electricity
Electrical Engineering
ISBN:9781337900348
Author:Stephen L. Herman
Publisher:Cengage Learning

Programmable Logic Controllers
Electrical Engineering
ISBN:9780073373843
Author:Frank D. Petruzella
Publisher:McGraw-Hill Education

Fundamentals of Electric Circuits
Electrical Engineering
ISBN:9780078028229
Author:Charles K Alexander, Matthew Sadiku
Publisher:McGraw-Hill Education

Electric Circuits. (11th Edition)
Electrical Engineering
ISBN:9780134746968
Author:James W. Nilsson, Susan Riedel
Publisher:PEARSON

Engineering Electromagnetics
Electrical Engineering
ISBN:9780078028151
Author:Hayt, William H. (william Hart), Jr, BUCK, John A.
Publisher:Mcgraw-hill Education,