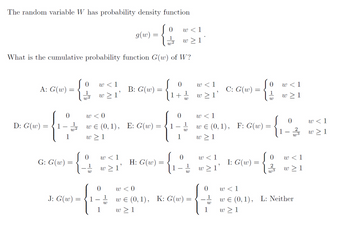
The random variable W has probability density function -{ = What is the cumulative probability function G(w) of W? g(w): 0 1 w < 1 w≥1

A shop receives a shipment of 1000 lamps. The probability that any individual lamp is defective is 0.2%. Assume the defectiveness is independent of cach lamp. Let X be the number of defective lamps in the batch of 1000. What is the probability mass function of X? O P(X = k) = (00) 0.002* (1 – 0.002)1000 , k = 0,1, 2, ..., 1000 O P(X = k) = 0.002* (1 – 0.002)1000–&, k = 0, 1, 2, .….., 1000 O P(X = k) = (1000) 0.002* (1 – 0.002)1000 , k = 1, 2, ..., 1000 O P(X = k) = (00)0.002100- (1 – 0.002)*, k = 0, 1, 2, ..., 1000

The probability that none of the three bulbs in a set of traffic lights will have to be replaced during the first 1200 hours of operation if the lifetime X of a bulb (in thousands of hours) is a random variable with probability density function f(x) = 6[0.25-(x-1.5)2] when 1 ≤ x ≤ 2 and f(x) = 0 otherwise is

Suppose X and Y are two independent Uniform(0, 1) random variables. Use the cumulative distribution function method to find the probability density function of their sum U = X + Y.