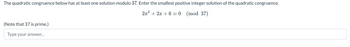
The quadratic congruence below has at least one solution modulo 37. Enter the smallest positive integer solution of the quadratic congruence. (Note that 37 is prime.) Type your answer... 2x2+2x+6=0 (mod 37)

c. Could you adapt Tylishia's method to other 183 49? If so, give at least two more examples subtraction problems, such as to the problem and show how to apply Tylishia's method in each 11. Tylishia says that she can calculate 324 - 197 by adding 3 to both numbers and calculating 327 – 200 instead. a. Draw a number line (which need not be perfectly to scale) to help you explain why Tylishia's method is valid. b. Explain in another way why Tylishia's method is valid. y -y ly, b- case. of

(a) The number N = 49,725 represents the ages of a group of teenagers multiplied together. How many teenagers are there and what are their ages? Explain. (b) Is there an integer N > 1 such that the square root, cube root, and fourth root of N are all integers, and if so, what is the smallest one?

Question 8b) I rounded to the nearest ten thousand and worked out that all of the numbers below will round to 420 000. The teacher marked it as incorrect as she rounded the numbers to the nearest thousand. By her textbook definition alone surely I was supposed to round to the nearest 10, 000? The number 420,000 has four zeros? Or am I wrong? Thanks for your time...

Problem 4. Let n be any positive integer. Prove that there exists a positive integer k (depending on n) such that the following list of n consecutive integers: k, k + 1, ·, k + n - 1 contains no prime number at all. = Hint. Use the factorial (but k n! is NOT the correct answer, start from this and try to see what are missing). You also need the 2-out-of-3 property of division.

please answer question 16 and 18 by using combination

Intro to Elementary Number Theory homework problem.

6. It is helpful to be able to think about very large numbers in terms of powers of 10. You should be familiar with many of these terms, but have you thought about how many 10's are multiplying each other? Here are some numbers to think about and examples of things that would be counted in these quantities. Fill in the proper power of 10. The first has been done for you. NUMBER POWER OF 10 EXAMPLE The distance between New York City and Boston is approximately 1 million feet. There are approximately 3 billion seconds in a century. There are 6 trillion miles in a light year, i.e. the distance light can travel in a year. There are approximately 1 quadrillion ants populating the earth at any time. There are approximately 8 quintillion grains of sand on all of the Earth's beaches. 1 million = 1,000 -1, 000 = 10 -10° = 10° 1 billion = 1,000 -1 million = 10' -10° = 1 trillion = (1 million) = (10")* = 1 quadrillion =1000-1 trillion = 1 quintillion = (1 billion)' =

Please show clear and concise work