Question
1
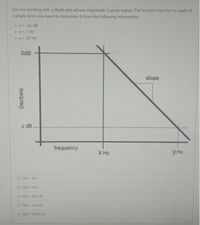
Transcribed Image Text:You are working with a Bode plot whose magnitude is given below. The transfer function is made of
a single term, you need to determine it from the following information.
•2- -26 dB
•X1 Hz
-y- 20 Hz
OdB
slope
z dB
frequency
X Hz
y Hz
G(s) - 1/s
Gis- s+1
O G) = 1/(s^2)
O Gi) - 1/s+1)
O Gi - 10/s+1)
Decibels

Transcribed Image Text:Question 6
The following Bode plot was derived from a system whose input is r(t) and output y(t). What is the
output when the input is r (t)
2. cos(2t – 90")?
%3D
Bode plot
20
10
-10
-20
-30
360
270
180
90
101
100
Frequency (rad/s)
10
102
O 8sin(2t+150)
O 0.2cos(2t+30)
O 12cos(21+90)
O 4cos(2t-90)
O 4cos(2t+90
Phase (deg)
Magnitude (dB)
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 3 steps with 1 images

Knowledge Booster
Similar questions