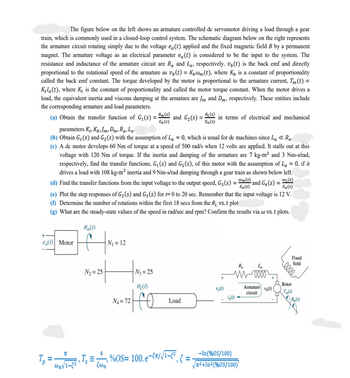
The figure below on the left shows an armature controlled de servomotor driving a load through a gear train, which is commonly used in a closed-loop control system. The schematic diagram below on the right represents the armature circuit rotating simply due to the voltage e(t) applied and the fixed magnetic field B by a permanent magnet. The armature voltage as an electrical parameter ea(t) is considered to be the input to the system. The resistance and inductance of the armature circuit are R₁ and La, respectively. v(t) is the back emf and directly proportional to the rotational speed of the armature as vy(t) = K₂@m(t), where K, is a constant of proportionality called the back emf constant. The torque developed by the motor is proportional to the armature current, Tm (t) = Kela(t), where K₂ is the constant of proportionality and called the motor torque constant. When the motor drives a load, the equivalent inertia and viscous damping at the armature are Jm and Dm, respectively. These entities include the corresponding armature and load parameters. (a) Obtain the transfer function of G₁(s) = m(s) and G₂ (s) = (s) in terms of electrical and mechanical Ea(s) Eg(s) parameters Kt, Kb.Jm. DmRa, La- (b) Obtain G₁ (s) and G₂ (s) with the assumption of La≈ 0, which is usual for de machines since La << Ra (c) A de motor develops 60 Nm of torque at a speed of 500 rad/s when 12 volts are applied. It stalls out at this voltage with 120 Nm of torque. If the inertia and damping of the armature are 7 kg-m² and 3 Nm-s/rad, respectively, find the transfer functions, G₁ (s) and G₂ (s), of this motor with the assumption of La = 0, if it drives a load with 108 kg-m² inertia and 9 Nm-s/rad damping through a gear train as shown below left. (d) Find the transfer functions from the input voltage to the output speed, G3 (s) = m(5) and G₂ (s) = (S) Ea(s) Ea(s) (e) Plot the step responses of G₂ (s) and G3 (s) for r=0 to 20 sec. Remember that the input voltage is 12 V. (1) Determine the number of rotations within the first 18 secs from the 0₁ vs. t plot (g) What are the steady-state values of the speed in rad/sec and rpm? Confirm the results via w vs. t plots. e(t) Motor M. N₂=25 |N₁ = 12 N₁ = 72 |N₂=25 B₁(t) Load (1) 400 0000 Armature ) circuit 0000 Rotor T (1) (1)

Note: Don't use chatGPT or google or any other source to copy paste. I will dislike the solution if you do so.I need unique solution. if you are not able to solve please let other expert review. The given diagram represents a sequential circuit. This circuit has a single input labeled "x" and a single output labeled "Z." It also has two state variables called Q1 and Q2, which together can have four different combinations of present states: 00, 01, 10, and 11.Create the state table and state diagramand boolean equation as well.

Read and solve carefully please write clearly and box the final answer

Kindly provide a COMPLETE and CLEAR solution. Answer it ASAP because I really need it right now. Answer should be typewritten. Instructions: Write the complete solution of the example given in the photo.

Hello, a step by step guide would be greatly appreciated. Please follow the NOTES & SUGGESTIONS at the bottom when solving, answering what is asked as I need to understand doing it and checking/verifying results, and please show every step for understanding, thanks so much :)