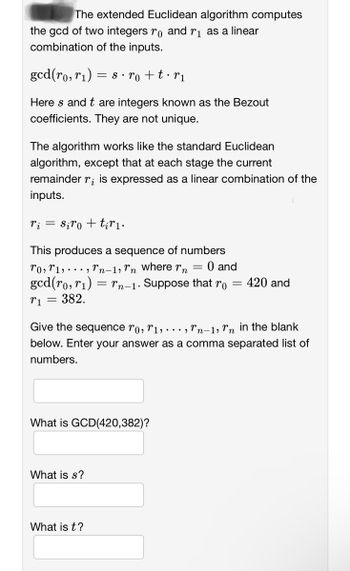
The extended Euclidean algorithm computesthe gcd of two integers ro and r₁ as a linearcombination of the inputs.gcd(ro, r₁) = s.ro + t •rıHere s and t are integers known as the Bezoutcoefficients. They are not unique.The algorithm works like the standard Euclideanalgorithm, except that at each stage the currentremainderri is expressed as a linear combination of theinputs.ri = siro + tir₁.This produces a sequence of numbersTo, T1,Tn-1, Tn where r = 0 andgcd(ro, r₁) = Tn-1. Suppose that ro = 420 andT1 = 382.Give the sequence ro, T1,..., Tn-1, în in the blankbelow. Enter your answer as a comma separated list ofnumbers.What is GCD(420,382)?What is s?What is t?

Given Information:The numbers are given as and .Objective:Find the of and by Euclidean Algorithm.…

The extended Euclidean algorithm computes the gcd of two integers ro and r₁ as a linear combination of the inputs. gcd(ro, r₁) = Here s and t are integers known as the Bezout coefficients. They are not unique. = s · ro + t · r₁ The algorithm works like the standard Euclidean algorithm, except that at each stage the current remainder î¿ is expressed as a linear combination of the inputs. rį = sįro + t¿r₁. = This produces a sequence of numbers To, 1,,Tn-1, n where n 0 and gcd(ro, r₁) = Tn-1. Suppose that ro T₁ = 382. What is GCD(420,382)? What is s? Give the sequence ro, T1,..., Tn-1, în in the blank below. Enter your answer as a comma separated list of numbers. What is t? = 420 and

The extended Euclidean algorithm computes the gcd of two integers ro and r₁ as a linear combination of the inputs. gcd(ro, r₁) = Here s and t are integers known as the Bezout coefficients. They are not unique. = s · ro + t · r₁ The algorithm works like the standard Euclidean algorithm, except that at each stage the current remainder î¿ is expressed as a linear combination of the inputs. rį = sįro + t¿r₁. = This produces a sequence of numbers To, 1,,Tn-1, n where n 0 and gcd(ro, r₁) = Tn-1. Suppose that ro T₁ = 382. What is GCD(420,382)? What is s? Give the sequence ro, T1,..., Tn-1, în in the blank below. Enter your answer as a comma separated list of numbers. What is t? = 420 and

Advanced Engineering Mathematics

10th Edition

ISBN:9780470458365

Author:Erwin Kreyszig

Publisher:Erwin Kreyszig

Chapter2: Second-order Linear Odes

Section: Chapter Questions

Problem 1RQ

See similar textbooks

Similar questions

Use Euclid's Algorithm to show that there are integers s and t such that 57s + 73t = 1. Showing all your working, compute [57]- in Z73. How many elements of Z73 have a multiplicative inverse?
Please answer only required in question Thanks
Use the inversion algorithm to find the inverse of A = 1 2 3 0 1 2 011
Use Euclid's algorithm to find gcd(340, 144). Hence find Icm (340, 144).
Please do the following questions with full handwritten working out
Find the inverse of the congruence 6x = 1 (mod 29) using Euclidean algorithm.
According to the Master Theorem, what is the run time of the algorithm described by the function T(n) - 4T () +e(nº")
Express efficient algorithms clearly for the below problem. Also, explain the asymptotic behavior of the algorithm. Assume a list of building blocks. Each block is a cube with a given size, and has ontop, a list of all the other blocks that can be placed directly on top of it. You are also given a number k. Determine whether or not you can build a tower with block 0 at the bottom and block 1 at the top using no more than k blocks. If so, we want to know the height of the shortest such tower. Example: given 0: 8, ontop=2,3 1: 15, ontop=0 2: 11, ontop=1,3,4 3: 10, ontop=0,1,2 4: 7 ontop=1 if k were 2 the answer would be no. if k were 4 the answer would be yes, with height 33 (block0 then block3 then block1)
please send complete handwritten solution for Q6 both part a & b
Someone used the Euclidean Algorithm to compute gcd(44,140) and found the following. Use this to write the gcd as a linear combination of 44 and 140. Show work. 140 = 3(44) + 8 44 = 5(8) + 4 8 = 2(4) + 0
(a) Use the Euclidean algorithm to find gcd(131,326). (b) Use the above to find a solution to 131x+326y=gcd(131,326) (c) Does 131 have an inverse modulo 326? If so, find a value in {0,1,2,3,…,325} that is an inverse. If not, explain why not?
(a) For integers a, b, q, r, prove that if a = bq + r then (a, b) = (b, r). (b) Determine (1000, 2025) by using the Euclidean algorithm. Then express the answer as a linear combination of 1000 and 2025. Show all your working. (c) Suppose that G = (a), a ‡e, and a5 : = e. Construct a Cayley table for the group (G,.). (d) Determine the right cosets of ((1 2), [1]) € S3 × Zi₂. (e) Find the subgroups of Z24.