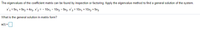
The eigenvalues of the coefficient matrix can be found by inspection or factoring. Apply the eigenvalue method to find a general solution of the system. x'1 = 9x, + 9x2 + 4x3, x'2 = - 10x, – 10x2 - 9x3, x'3 = 10x1 + 10×2 + 9x3 What is the general solution in matrix form? x(t) =

By using the method of least squares, find the best parabola through the points: (-1,-3). (1,3). (-2,-3). (0,-3) Step 1. The general equation of a parabola is c₂+₁+c₂2²-3. Plugging the data points into this formula gives a matrix equation Ac=y. 1 ATA- ATy= 1 Step 3. Solving the normal equation gives the answer -8 which corresponds to the formula a Step 2. The matrix equation Ac=y has no solution, so instead we use the normal equation A¹A = A¹y O 0 3

Use the Jacobi iterative method (Vector-Matrix Form) with r0) = (2, 2) to solve the linear system. Find one iteration only. 5x1 – 3r2 = 7 -4x1 + 7x2 = -1

If possible, only solve for x1 and x2. I have solved the other parts but having problem with x1 and x2.      Also, the the matrix is below. Just in case it does not appear clear.  0 -3 9 -4 4 -18   0 0 4