
Ciccarelli: Psychology_5 (5th Edition)
5th Edition
ISBN: 9780134477961
Author: Saundra K. Ciccarelli, J. Noland White
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Question
this is what I need to show full work on for psych stats

Transcribed Image Text:**Positive Events and Risk-Taking Behavior**
Recent research suggests that unexpected positive outcomes, such as an unseasonably sunny day, can lead to higher levels of risk-taking and gambling (Otto, Fleming, & Glimcher, 2016). This was demonstrated by comparing lottery sales—a measure of risk-taking—on normal days to those on days with unexpected positive events in the city. An increase in sales was observed after such positive occurrences.
In an experimental setup, participants are divided into two groups. Group 1 receives an unexpectedly large payment for participation, while Group 2 receives the expected compensation. The researcher then measures the amount each participant is willing to gamble in a game of chance.
**Table Summary**
The table compares the two groups:
- **Unexpected Positive Outcome**
- Sample size (\( n \)) = 16
- Mean (\( M \)) = 5.75
- Sum of squares (\( SS \)) = 6.5
- **Expected Outcome**
- Sample size (\( n \)) = 16
- Mean (\( M \)) = 5.00
- Sum of squares (\( SS \)) = 10.0
**Hypothesis Testing**
The hypothesis being tested is whether an unexpected positive outcome increases the amount participants are willing to gamble, using a one-tailed test.
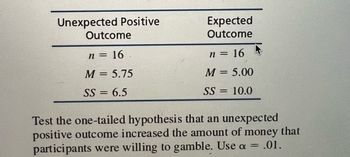
Transcribed Image Text:### Analysis of Gambling Outcomes
The table compares two conditions in an experiment related to gambling behavior:
#### Unexpected Positive Outcome
- **Sample Size (n)**: 16
- **Mean (M)**: 5.75
- **Sum of Squares (SS)**: 6.5
#### Expected Outcome
- **Sample Size (n)**: 16
- **Mean (M)**: 5.00
- **Sum of Squares (SS)**: 10.0
#### Hypothesis Testing
The task is to test the one-tailed hypothesis that an unexpected positive outcome increases the amount of money participants are willing to gamble. The significance level is set at α = 0.01.
This involves comparing the means of the two sample groups using statistical methods to determine if the unexpected positive outcome has a significant effect on gambling behavior.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 4 steps with 10 images

Knowledge Booster
Recommended textbooks for you
 Ciccarelli: Psychology_5 (5th Edition)PsychologyISBN:9780134477961Author:Saundra K. Ciccarelli, J. Noland WhitePublisher:PEARSON
Ciccarelli: Psychology_5 (5th Edition)PsychologyISBN:9780134477961Author:Saundra K. Ciccarelli, J. Noland WhitePublisher:PEARSON Cognitive PsychologyPsychologyISBN:9781337408271Author:Goldstein, E. Bruce.Publisher:Cengage Learning,
Cognitive PsychologyPsychologyISBN:9781337408271Author:Goldstein, E. Bruce.Publisher:Cengage Learning, Introduction to Psychology: Gateways to Mind and ...PsychologyISBN:9781337565691Author:Dennis Coon, John O. Mitterer, Tanya S. MartiniPublisher:Cengage Learning
Introduction to Psychology: Gateways to Mind and ...PsychologyISBN:9781337565691Author:Dennis Coon, John O. Mitterer, Tanya S. MartiniPublisher:Cengage Learning Psychology in Your Life (Second Edition)PsychologyISBN:9780393265156Author:Sarah Grison, Michael GazzanigaPublisher:W. W. Norton & Company
Psychology in Your Life (Second Edition)PsychologyISBN:9780393265156Author:Sarah Grison, Michael GazzanigaPublisher:W. W. Norton & Company Cognitive Psychology: Connecting Mind, Research a...PsychologyISBN:9781285763880Author:E. Bruce GoldsteinPublisher:Cengage Learning
Cognitive Psychology: Connecting Mind, Research a...PsychologyISBN:9781285763880Author:E. Bruce GoldsteinPublisher:Cengage Learning Theories of Personality (MindTap Course List)PsychologyISBN:9781305652958Author:Duane P. Schultz, Sydney Ellen SchultzPublisher:Cengage Learning
Theories of Personality (MindTap Course List)PsychologyISBN:9781305652958Author:Duane P. Schultz, Sydney Ellen SchultzPublisher:Cengage Learning

Ciccarelli: Psychology_5 (5th Edition)
Psychology
ISBN:9780134477961
Author:Saundra K. Ciccarelli, J. Noland White
Publisher:PEARSON

Cognitive Psychology
Psychology
ISBN:9781337408271
Author:Goldstein, E. Bruce.
Publisher:Cengage Learning,

Introduction to Psychology: Gateways to Mind and ...
Psychology
ISBN:9781337565691
Author:Dennis Coon, John O. Mitterer, Tanya S. Martini
Publisher:Cengage Learning

Psychology in Your Life (Second Edition)
Psychology
ISBN:9780393265156
Author:Sarah Grison, Michael Gazzaniga
Publisher:W. W. Norton & Company

Cognitive Psychology: Connecting Mind, Research a...
Psychology
ISBN:9781285763880
Author:E. Bruce Goldstein
Publisher:Cengage Learning

Theories of Personality (MindTap Course List)
Psychology
ISBN:9781305652958
Author:Duane P. Schultz, Sydney Ellen Schultz
Publisher:Cengage Learning