Suppose X₁,..., X₁, Z are independent N(µ, t−¹) random variables and the prior density of (T, μ) is -zª-¹e-Bt. (2π)-¹/2(kt)¹/2 exp +/+ (1 - 1)²} as in Example 3.3. Recall that in Example 3.3 we showed that the posterior density of (t, µ), given X₁, ..., Xn, is of the same form with parameters (a, b, k, v) replaced by (a', p', k', v'). Suppose now that the objective is prediction about Z, conditionally on X₁,..., X. Show that, if л(t, µ; α', ß', k', v') is the posterior density of (t, μ) and Z|(t, µ) ~ N(µ, -¹), then the joint posterior density of (T, Z) is of the form □(t, Z; α", ß", k", v") and state explicitly what are a", B", k", v". Show that the marginal predictive density of Z, given X₁,..., X, is л(т, μ;α, ß, k, v) = Ba r(a)

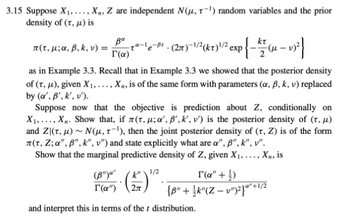
Given independent normal random variables X1, ..., Xn ~ N(u, T^-1), and a normal random variable Z ~ N(u, T^-1), and the prior density of (T, u) given by

π(T, u; a, B, k, v) = βα(a) T^(-a-1) (2π)^(-1/2) (kT)^(-1/2) exp{-B/2T}

where βα(a) = Γ(a)/[Γ(a/2)π^(1/2)], and Γ(a) is the gamma function.

The posterior density of (T, u) given X1, ..., Xn is given by:

π(T, u|X1, ..., Xn; a', B', k', v') = β''α''(a'') T^(-a''-1) (2π)^(-1/2) (k''T)^(-1/2) exp{-B''/2T}

where β''α''(a'') = Γ(a''/[Γ(a''/2)π^(1/2)], and a'', B'', k'', and v'' are updated parameters given by:

a'' = a + n/2 B'' = B + (Σ(xi - u)² + n/(1+n/2)(Σxi/n - u)²)/2 k'' = k + n/2 v'' = (kv + Σti)/(k+n)

Now, the joint posterior density of (T, Z) given X1, ..., Xn is proportional to the likelihood times the prior, that is:

π(T, Z|X1, ..., Xn) ∝ f(X1, ..., Xn, Z|T, u) π(T, u; a', B', k', v')

where f(X1, ..., Xn, Z|T, u) is the joint density of the observed data and Z given T and u, which is given by:

f(X1, ..., Xn, Z|T, u) = (2π)^(-n/2) T^(-n/2) exp{-[(Z - u)^2 + Σ(Xi - u)^2]/(2T)}

Substituting the expressions for the prior and likelihood into the joint posterior density and simplifying, we obtain:

π(T, Z|X1, ..., Xn) = β'''α'''(a''') T^(-a'''-1) (2π)^(-1/2) (k'''T)^(-1/2) exp{-B'''/2T}

where β'''α'''(a''') = Γ(a'''/2)/[Γ(a'''+1/2)π^(1/2)], and a''', B''', k''', and v''' are updated parameters given by:

a''' = a'' + 1/2 B''' = B'' + (Z - u)^2/2 + k''/[2(T + n)](Z - v'')^2 k''' = k'' + 1/2 v''' = (kv'' + Z)/(k''' + 1)