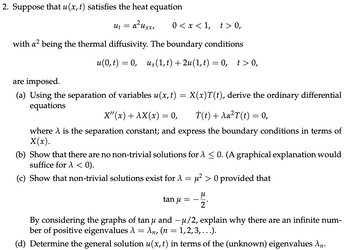
Suppose that u(x, t) satisfies the heat equation Ut = α-Uxx, a²uxx, 0 0, with a² being the thermal diffusivity. The boundary conditions u(0, t) = 0, ux(1, t) +2u(1, t) = 0, t> 0, are imposed. (a) Using the separation of variables u(x, t) = X(x)T(t), derive the ordinary differential equations X"(x) + AX(x) = 0, İ(t) + Aa²T (t) = 0, where A is the separation constant; and express the boundary conditions in terms of X(x). (b) Show that there are no non-trivial solutions for A ≤ 0. (A graphical explanation would suffice for A< 0). (c) Show that non-trivial solutions exist for λ = µ²> 0 provided that tan μ == 2 By considering the graphs of tan µ and -µ/2, explain why there are an infinite num- ber of positive eigenvalues A = An, (n = 1,2,3,...).

For the equation af = k at with boundary conditions f(x = 0, t) = f(x 1, t) 0, and initial condition f(x,t = 0) = x(1-x), determine the full solution.

H2. A particle of mass m moves in a straight line under the action of a conservative force F(x) with potential energy U(x) = x²e-*. (i) Calculate F(x) and find the two equilibrium points of the system (i.e., points xẻ such that F(x) = 0). Compute if the equilibria are stable (i.e., local minima of the potential energy: U" (xe) > 0) or unstable (i.e., local maxima of the potential energy: U"(xe) 0. Find the initial energy Eo of the particle. Using (ii), show that the particle reaches x = 2 only if vo > ŷ, with 8e-2 v= and in this case the particle's velocity in x = 2 is v(2): = (a) x = 0, vo=0 (b) x=2, vo = 0 (c) x = 0, vo= 0.5 (d) x = 0, vo = -0.5 (e) x = 0, vo=2 (f) x = 0, vo= -10. m 8e-2 v². m (iv) Assume the particle starts in xo =0 with positive initial velocity vo > ŷ. Use (ii) to find the expression for v(x) and find the terminal velocity of the particle as x → ∞. If the particle starts with negative initial velocity vo < 0, can it escape to x →→∞? (v) Assume m= 1, show that the…

Given yı (t) = t and y2(t) = t satisfy the corresponding homogeneous equation of t'y" – 2y = 1 – 2t°, t > 0 Then the general solution to the nonhomogeneous equation can be written as y(t) = c1y1(t) + ©2Y2(t) + Yp(t). Use variation of parameters to find a particular solution yp(t). Y,(t) = Tip: Before you use the formula Y29(t) Yı9(t) JW(n, y2) Yp = - Y1 + y2 W (y1, Y2) the ODE should be in the form y'' + a(t)y' + b(t)y = g(t)