A First Course in Probability (10th Edition)
A First Course in Probability (10th Edition)
10th Edition
ISBN: 9780134753119
Author: Sheldon Ross
Publisher: PEARSON
Bartleby Related Questions Icon

Related questions

Q: Let X and Y continuous random variable with joint pdf fx,y) = 24xy, for 0<x<1, 0<y<1-x %3D Find P(Y…
A: Let X and Y continuous random variable with joint pdf fx,y=24xy,   0<x<1, 0<y<1-x…
Q: Let X be a continuous random variable with PDF fx(x) = { 5x4 0<x< 1 otherwise If Y = 1/X², calculate…
A: The  is a continuous random variable. The PDF is, Here, The objective is to find .
Q: Suppose X is a continuous random variable defined on (-∞, ∞) and its probability density is of the…
A:
Q: Suppose we have the quadratic function f(x)=A(x^2)+C where the random variables A and C have…
A: A quadratic equation is written as f(x)=Ax2+Bx+C. f(x) has real roots if B2-4AC≥0.
Q: Suppose we have the quadratic function f(x)=A(x^2)+2X+C where the random variables A and C have…
A: Let the quadratic equation is f(x)=Ax2+2X+C. From the question, f(A)=A2     0≤A≤2 f(C)=3C2    0≤C≤1…
Q: Suppose that X₁, X2, X3 are independent and identically distributed random variables with…
A: Solution
Q: If the continuous random variable X has the probability density ( 62(1 - 2) for 0<z<1 elsewhere f(2)…
A:
Q: Suppose that X1,.…,X, is a random sample from a distribution with density f(x; 0) = 0x-?, r E (8,…
A: We have given that Let X1 X2 ....Xn be the random sample of size n having the probability density…
Q: ) Write the joint probability density function. p) Express F(z) = P(¥ <z) using integrals and…
A: Let X and Y be continuous independent random variable have exponential distribution with mean θ
Q: Let X ~ U(a,b). Use the definition of the mean of a continuous random variable to show that μX =…
A: The mean of the uniform continuous random variable can be calculated as:
Q: The 4-dimensional random vector X has PDF fX(x)={1 when 0≤xi≤1, i=1,2,3,4 and 0 otherwise}. Are the…
A: Step 1: Step 2: Step 3: Step 4:
Q: Let X be a continuous random variable with density f (x) = 24x-4 for æ > 2. Then Var (X) is equal to
A:
Q: =. Let X, Y and Z have the joint pdf x² + y? + z x2 + y? + z2 (2т) 3/2 1+ xyz exp exp where -o < x <…
A: The joint density pdf of X, Y and Z is, fx,y,z=12π32e-x2+y2+z221+xyze-x2+y2+z22 Consider,…
Q: Let  X  be a continuous random variable with range  [0,1]  and its probability density function is…
A:
Q: Consider the random variable X with the uniformdensity having α = 1 and β = 3.(a) Use the result of…
A:
Q: Suppose that X is a random variable with the density: f(x) = (  θxθ−1  if      x ∈ (0, 1)…
A: Suppose that X is a random variable s.t. f(x)=θxθ-1 ,  0<x<1 PART A By the Moment method ,…
Q: Let X1, X2,..., X, is a random sample from a distribution with density function f(x; 0) = 302e-0³…
A: The given probability density function (PDF) is:f(x;θ)=302e^-θ3for0x∞,otherwise
Q: Suppose that X₁, X2, X3 are independent and identically distributed random variables with…
A: Given Cdf  F(x)=1-2-x; x≥0
Q: Let X1,., X, denote a random sample from the probability density function f(r) = 0x-1, 0 0. Show…
A:
Question
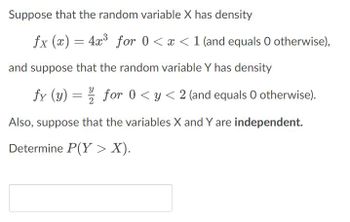
Suppose that the random variable X has density fx (x) = 4x³ for 0 < x < 1 (and equals 0 otherwise), and suppose that the random variable Y has density fy (y) =/ for 0 < y < 2 (and equals 0 otherwise). Also, suppose that the variables X and Y are independent. Determine P(Y > X).
expand button
Transcribed Image Text:Suppose that the random variable X has density fx (x) = 4x³ for 0 < x < 1 (and equals 0 otherwise), and suppose that the random variable Y has density fy (y) =/ for 0 < y < 2 (and equals 0 otherwise). Also, suppose that the variables X and Y are independent. Determine P(Y > X).
Expert Solution
Check Mark
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
See solutionCheck out a sample Q&A here
Step 1
VIEW
Step 2
VIEW
bartleby

Step by stepSolved in 2 steps with 1 images

See solution
Check out a sample Q&A here
Blurred answer
Still need help?
Follow-up Questions
Read through expert solutions to related follow-up questions below.
Follow-up Question
Suppose that the random variable X has density ƒx (x) = 4x³ for 0 < x < 1 (and equals 0 otherwise), and suppose that the random variable Y has density fy (y) = for 0 ≤ y ≤ 2 (and equals O otherwise). Also, suppose that the variables X and Y are independent. Determine P(Y> 4X).
expand button
Transcribed Image Text:Suppose that the random variable X has density ƒx (x) = 4x³ for 0 < x < 1 (and equals 0 otherwise), and suppose that the random variable Y has density fy (y) = for 0 ≤ y ≤ 2 (and equals O otherwise). Also, suppose that the variables X and Y are independent. Determine P(Y> 4X).
Solution
Bartleby Expert
by Bartleby Expert
SEE SOLUTION
Follow-up Questions
Read through expert solutions to related follow-up questions below.
Follow-up Question
Suppose that the random variable X has density ƒx (x) = 4x³ for 0 < x < 1 (and equals 0 otherwise), and suppose that the random variable Y has density fy (y) = for 0 ≤ y ≤ 2 (and equals O otherwise). Also, suppose that the variables X and Y are independent. Determine P(Y> 4X).
expand button
Transcribed Image Text:Suppose that the random variable X has density ƒx (x) = 4x³ for 0 < x < 1 (and equals 0 otherwise), and suppose that the random variable Y has density fy (y) = for 0 ≤ y ≤ 2 (and equals O otherwise). Also, suppose that the variables X and Y are independent. Determine P(Y> 4X).
Solution
Bartleby Expert
by Bartleby Expert
SEE SOLUTION
Knowledge Booster
Background pattern image
Similar questions
Recommended textbooks for you
Text book image
A First Course in Probability (10th Edition)
Probability
ISBN:9780134753119
Author:Sheldon Ross
Publisher:PEARSON
Text book image
A First Course in Probability
Probability
ISBN:9780321794772
Author:Sheldon Ross
Publisher:PEARSON
SEE MORE TEXTBOOKS